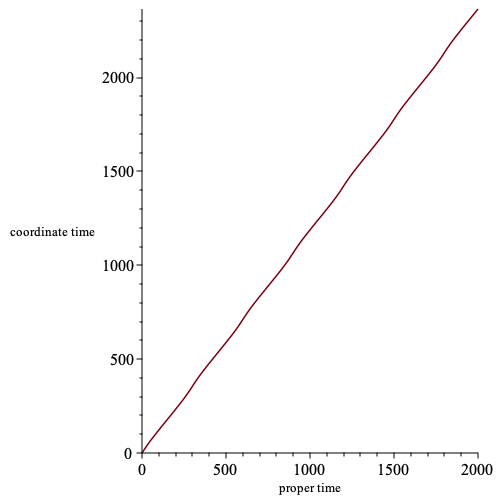
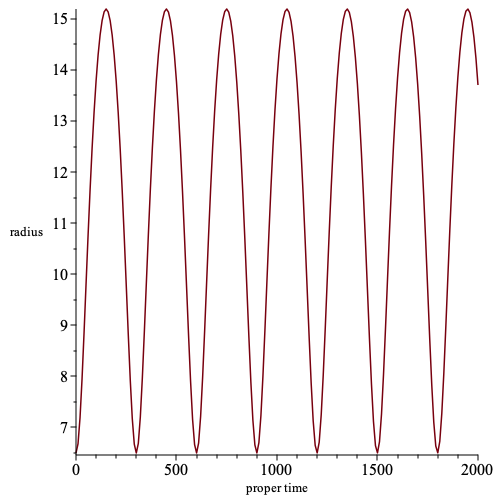
I'm solving the Schwarzschild geodesics numerically, and I'm wondering if the result I'm getting makes physical sense. The parameter of the path $x^{\mu}=(t,r,\theta,\phi)$ is the proper time $\tau$, and when I graph the coordinate time $t$ against the proper time, I get a really straight, linear relation, which, considering the wild oscillation in the radial coordinate (ie: 6+ oscillations between 6.5 and 15 $GM$), I have a hard time believing.
Granted, the coordinate time is always greater than the proper time, so time-dialation IS taking place.
Below I've attached the 2 corresponding plots.
For reference, I'm integrating the equations from the wiki page https://en.wikipedia.org/wiki/Schwarzschild_geodesics#Geodesic_equation with maple's dsolve(numeric), rk45 .