3
$\begingroup$

I have been recently brushing up on my elementary physics concepts, specifically quantum physics. If I set up a single photon emitter and conducted the double slit experiment, it is possible for me to see interference. When I thought about this I realized that a photon is mass-less and travels at the speed of light, so time does not affect a photon. Therefore, a photon can interact with another instance of itself (which in our frame of reference exists in another time).

My question is, is this same effect observable when a single electron is fired at speeds much lower than the speed of light?

If so, how? For this to happen, the electron (which has mass and experiences time the way we do) has to be physically present at two locations at the same time (in both slits).

Improve this question
$\endgroup$
1
  • 4
    $\begingroup$ The fact that a photon can be nonlocalized has nothing to do with the fact that it doesn't have a varying proper time $\tau$. When we observe interference, it's because two different parts of the wave coincide at the same coordinates $(t,x,y,z)$ in some frame such as the lab. $\endgroup$
    – user4552
    Commented Apr 30, 2013 at 12:50

4 Answers 4

Reset to default
8
$\begingroup$

Yes, electrons can be brought to interfere with themselves. This can actually be shown in a double slit experiment, just as with photons.

The electron you are thinking of is a localized particle in space. Instead, you have to consider the electron's position as a wave function. The wave function can be non-zero at both slits and interfere with itself afterward. With electrons, you will also find the typical stripes (or rings, if you use a circular aperture as a single slit) that you found with photons.

This is one of the groundbreaking experiments that one can conduct in schools to prove that electrons actually are both, a wave and a particle.

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ Well, right, except that the OP talks about "interaction" and the interference of two portions of a wave function isn't an interaction. $\endgroup$
    – Luboš Motl
    Commented Apr 26, 2013 at 13:14
  • 2
    $\begingroup$ I suspect that the OP is using "interact" in a colloquial rather than formal sense. It is certainly true that electrons can generate two-slit interference patterns even when the rate is turned down low enough that the mean number in transit drops below unity, which is how I interpreted the question. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Apr 26, 2013 at 13:57
  • $\begingroup$ I see it as dmckee. The OP refers to a Photon's self-interaction in the double slit experiment. As uncharged particles, photons have no (tree-level) self-interaction which makes me interpret "interaction" as "superposition of wave function". $\endgroup$
    – Neuneck
    Commented Apr 29, 2013 at 5:55
  • 1
    $\begingroup$ I think I would add to "you have to consider the electron's position as a wave function. " 'whose square gives the probability of finding the electron at (x,y,z,t) ' . too often people think that the mass of the electron is spread all over the space. When one is given a probability for living to 80years one is not spread out from now to then ;) $\endgroup$
    – anna v
    Commented Apr 30, 2013 at 9:41
  • $\begingroup$ What do you mean by wave function interfering with itself? Wave function is a mathematical abstraction to tell about probability where the electron might land. But what will it land anywhere else apart from the line of sight of the both slits? Something is really wrong with the double slit experiment and people still don't know how to explain it well. $\endgroup$
    – Thinker-101
    Commented Oct 27 at 17:15
4
$\begingroup$

Yes, exactly the same happens with electrons. Indeed, the experiment has been done and is described in this paper. Electrons were sent through a Young's slit experiment configuration one at a time, and the usual diffraction pattern was obtained. Proof, if any were necessary, that the electrons are delocalized when they pass through the slits.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Very interesting paper! What strikes me most is that it appears as-if the single slit experiments also show some diffraction pattern (Figure 2). What is the explanation for that? $\endgroup$
    – atlaste
    Commented Mar 30, 2018 at 5:48
4
$\begingroup$

I am adding this answer because the previous ones, though correct, do not emphasize that it is the probability of interaction of the electron that waves.

According to the well-validated theory of quantum mechanics, the wave function solution of a given potential or scattering problem, $Ψ$ is complex, i.e., not measurable. It is $Ψ^*Ψ$, a real number, that can be compared with the electron position measurement, and according to the postulates of quantum mechanics, it gives the probability for the electron to be measured at (x,y,z) at time t. It is not the electron's mass or energy that is waving, i.e., have sinusoidal behavior by showing interference patterns in the single electron double slit experiment.

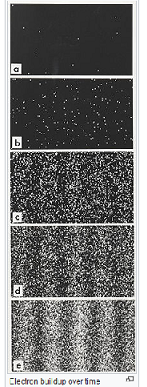
dblslit

electron build up over time

The electron is a point particle whenever it is measured, as in the pictures above, and this assumption is checked by experiments which give very tiny limits.

The accumulation of many electrons with the same scattering boundary conditions, as in the double slit experiment, shows a wave nature, confirming the probability behavior predicted by quantum mechanics.

So the electron interacts with the potentials and boundary conditions it is subjected to, and in interacting displays a probabilistic mechanical behavior, not deterministic as in classical mechanics interactions. This probability is what is predictable (determinable) in quantum mechanics, and is responsible for what is called the "wave" nature of a particle, which can only appear under accumulated interactions with the same boundary conditions.

This also answers @rolls.

Improve this answer
$\endgroup$
2
  • $\begingroup$ @atlaste this may answer your comment $\endgroup$
    – anna v
    Commented Jul 2, 2018 at 5:17
  • $\begingroup$ @rolls this may answer your deleted question $\endgroup$
    – anna v
    Commented Jul 2, 2018 at 5:18
1
$\begingroup$

An electron and photon are quite different particles. However, they have something in common that makes them both interfere in double-slit experiments. The common feature is their wave function, which means they obey the same laws describing their probabilistic propagation in space and time. In the double-slit experiments, their masses are particular properties having an influence on their interference patterns.

Thus, I would rather not say an electron interacts with itself, but rather, its possible trajectories of propagation interfere. If you send a single electron through a double-slit screen, your detector indicates it as a single spot. But if the experiment is repeated, you will get an interference picture.

My question is, is the same effect observable when a single electron is fired at speeds much lower than the speed of light?

In quantum mechanics, speed (momentum) is related to the wavelength of the particle. So the interference is dependent on the momentum. At larger speeds, slits have to be smaller to observe interference.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.