I have seen that:
In the ground state ($T = 0$) formulation of the Green’s function written in terms of operators in the interaction picture, the Green’s function reads:
$$G(r,t;r',t') = -i\frac{\langle T[\Psi(r,t)\Psi^{*}(r',t')S(+\infty,-\infty)]\rangle}{S(+\infty,-\infty)}.$$
One then finds that the denominator $S(+\infty, −\infty)$ i cancels the contribution of disconnected diagrams so that:
$$G(r,t;r',t') = -i\langle T[\Psi(r,t)\Psi^{*}(r',t')S(+\infty, −\infty)]\rangle_{\rm connected}.$$
In Keyldysh formalism, the Keldysh Green function reads: $$G^{i,i'}(r,t;r',t') = -i\langle T_{k}[\Psi(r,t_{i})\Psi^{*}(r',t'_{i'})S_{k}(+\infty, −\infty)]\rangle_{\rm connected}.$$
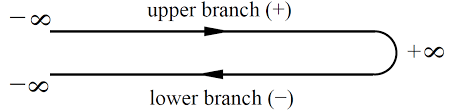
$T_{k}$ is the time ordering on a Keldysh countour. The Keldysh contour goes from $−\infty$ to $+\infty$ (+ time label) and back from $+\infty$ to $-\infty$ (− time label).
This expression contains connected and disconnected diagram but why in practice only the connected diagram need to be included in the series?