Motion of electrons in current

Question

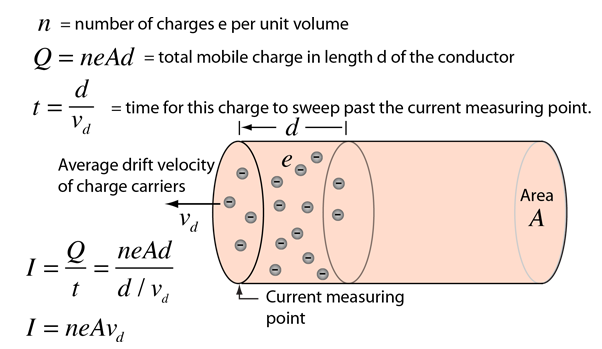
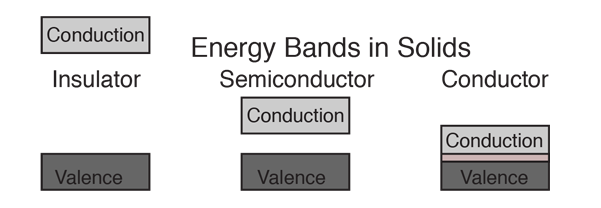
When electrons move in an electric current, do they move by jumping from atom to atom or by moving between the spaces of atoms?

Answer 1

It's convenient to tell new students that electrons are point particles which are sometimes found "orbiting a nucleus" and are other times found "outside of a nucleus." But that's a fiction which we use to help our primate brains apply our macroscopic experience to the microscopic world. When we say that an electron is a "point particle" we mean it is structureless, in contrast to the way that protons and neutrons have internal degrees of freedom which can be excited. But if you want a mental picture of an electron's size, you have to know about its wavelength, and the wavelength of an electron changes depending on what interactions it's participating in.

Let's skip the motivating details of band theory and use the result that a conduction electron is a quasi-free particle with a thermal-ish energy:

$$ \mathit{KE} = \frac{p^2}{2m_e} \approx kT \approx 25\,\mathrm{meV} $$

Here I'm using $\mathit{KE}$ for kinetic energy, $p$ for momentum, $m_e$ for electron mass, $k$ for the Boltzmann constant, and $T$ for the temperature. The milli-electron-volt (meV) is an energy unit. These electrons will have a characteristic wavelength

$$ \newcommand{\AA}{\unicode{x212B}} \lambda = \frac hp \approx 77\, \AA $$

Typical lattice spacings in a solid are twenty times smaller than this (about $3.5\,\AA$ for copper). In the jargon we say these electrons are "de-localized," because their wavelength is huge compared to the size of any single atom. I prefer to say "electrons are huge at this temperature," but I find it upsets people who are working with the model that "an electron" is a zero-size particle.

It doesn't make sense to say either that an electron "hops from atom to atom" nor that it "moves in the spaces between atoms." It makes a little more sense to say that each conduction electron is "smeared out" in a quantum-mechanical way, and overlaps with many atoms at once.

(Note that we can apply this same analysis to the ions on the lattice. For copper we find

$$ \lambda_\text{ion} = \frac h{p_\text{ion}} = \frac h {\sqrt{ 2 m_\text{Cu} kT } } \approx 0.2\,\AA $$

This says that any quantum-mechanical strangeness in locating a copper ion starts at distances much smaller that the actual distance between copper ions in the lattice, and we can get away with pretending we know "where the atoms are" in a way that we can't for the conduction electrons.)

Answer 2

Electrons and atoms are within the realm of quantum mechanics, and quantum mechanical models are needed to really describe correctly what is happening. This means that the motion you envisage is a macroscopic end effect, and is illustrated here:

The correct quantum mechanical models use the band theory of solids

It is the electrons in the conduction band that are the ones acting as the first image. They are shared with the whole lattice of the solid and act as "free" when a potential difference is applied.

Answer 3

Yes, the electrons are moving between the spaces of atoms. In electrolytes, positively charged ions move too - to the opposite electrode (cathode). The may stick to the cathode and be removed from the electrolyte. Read something about Faraday experiments, for example.

Answer 4

Electrons do not move in an electric current, they are the electric current.