How much pressure will be generated if liquid helium is placed in a sealed container at room temperature? [closed]

Question

I know that helium has the lowest boiling point so I was curious how much pressure it would exert if liquified and trapped?

Answer 1

He cannot be a liquid at room temperature. You would have to have very cold liquid He to start with then let the temperature increase and evaporate the He in the container. I assume the container is not initially completely filled with He. (If completely filled, as the liquid He heats up it could rupture the container, since the density decreases with temperature and a liquid is essentially incompressible.)

Case (1) You keep the He sufficiently cold as to always have liquid He present.

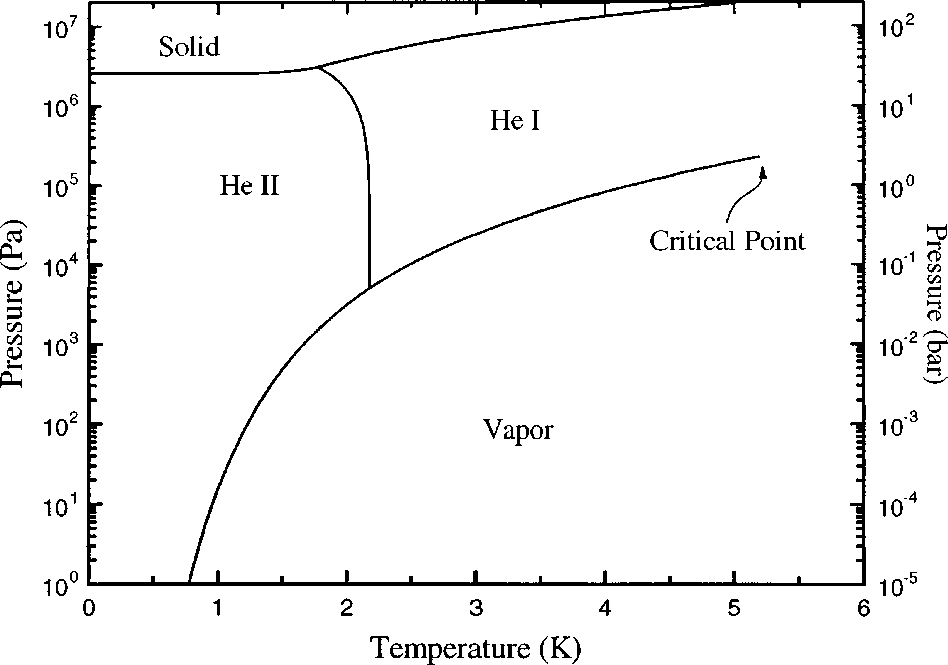
The vapor pressure depends on the temperature as follows for He 4. He I and He II in the figure indicate the two liquid phases of He 4. A pressure of 1 atmosphere is about $10^5$ Pa. Above the critical point there is no distinction between the liquid and gas states, so you need to keep the temperature below about 5 K to maintain any liquid He. Note that at these low temperatures (below 5 K), any air/water vapor trapped in the top of the container will not contribute to the pressure.

Updated response: Case (2) If the He is at room temperature it is all gas. You can estimate the pressure using the ideal gas law $pV = nRT$. You know the temperature and volume, and you calculate the moles n from the initial mass of liquid He that has all evaporated; then you calculate pressure p. For a more accurate estimate, not assuming an ideal gas, you need to have a table with the state properties of He at room temperature. The total pressure will be the partial pressure of air/water vapor plus the partial pressure of He gas.

Answer 2

If you look up the phase diagram of Helium-4, you'll find that at pressures sufficiently high, when the temperature is above about 4.7K the distinction between liquid and gas phases disappears. This implies that at room temperature you could, in principle, just keep pumping in helium pretty much forever, increasing the pressure to extremely high levels until it solidifies- unless, of course, a point is reached where there is no distinction between liquid, gas, and solid. I'm pretty sure that we have, at best, only theoretical knowledge of how helium behaves under those conditions.

As you've posed the problem, though, the mass of the gas/liquid will not exceed a mass equivalent to the density of liquid helium times the volume of the container. This will set an upper limit to the pressure. To estimate that upper limit, you can use the Ideal Gas Law and insert the appropriate values.

Answer 3

The internet suggests that the density of liquid helium is about $125 \space\text{kg/m}^3$. It's $4 \space\text{g/mol}$, so $n/V=31250\space \text{mol/m}^3$.

You close a container that is completely full of liquid helium and let it warm to room temperature ($T=293 \space\text{K}$). The container stays the same size so the density doesn’t change.

If we assume helium at that density and room temperature behaves as an ideal gas (for this to be a really good approximation the atoms can’t be crammed too close together, and these ones are pretty crammed), then $P=(n/V)RT$.

This gives $P=76.1 \space\text{MPa}$. That's about $751 \space\text{atm}$. You would need a very strong container.