Is there a loss of Angular momentum in this question?

Question

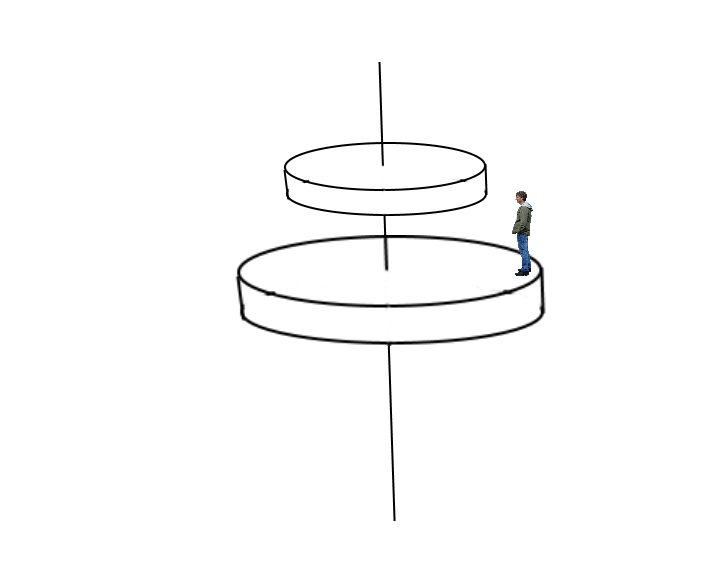
I am learning about angular momentum and I have a confusion. According to the guidelines of the website, I am not here just randomly asking random questions without thinking. In fact, to prove one point, I had to find suitable example so please comment on any issues (if you have) before downvoting and I will resolve that. The term Angular momentum is defined as $r \times mv$ now what I don't understand here is that why do we have $r$ in that formula. So to understand it deeply, I have an example and I will rely mostly on liner terms rather than angular terms because then we can easily apply Newton's Laws.

Let's call the bigger disk B and the smaller one A. Now assume that the disk B is rotating with angular velocity $\omega$ and disk A is rotating with $2\omega$. The radius of disk B is $2R$ and that of disk A id $R$. Let's say the mass of the man is $m$ and that if disk A and disk B is same and is $M$. Consider friction in such amount that all of tasks in example is successfully carried out. These are the variables required for me (You can assign your own if needed). Now the man jumps and catches disk A. When this happened, linear momentum of the man was $2Rm \omega $ and when he was about to land the other disk, his momentum in the XZ plane should be conserved and he would have the same momentum which is $2Rm \omega $. Now since the velocity at the tip (by tip I mean any point on circumference of the disk) on the other disk is also the same which is $R 2 \omega$ hence he would land easily and if we go into the frame of man, we see that according to him the tip of disk A and tip of disk B are having relative linear velocity $0$. So the man would land easily on the other disk and the other disk would feel no change in its rotational motion (please correct me if I am wrong). Now according to the axis of the disk, previously the angular momentum of the disk was $$\frac{\omega M(2R)^2}{2} + \frac{\omega 2M(R)^2}{2} + \omega m(2R)^2$$ and now after the jump, it has become $$\frac{\omega M(2R)^2}{2} + \frac{\omega 2MR^2}{2} + \omega 2mR^2.$$ Now since there was no external force in effect then the Angular momentum should not change but why did it change. and I think that $R$ is creating any issue here.

Answer 1

A force is required to move the man inward. If that force is radial (directly toward the axle) it speeds him up, and he is actually moving faster than the outer edge of the smaller disk when he gets there. Conversely, if the man exerts a force on the large disk in order to move himself inward, and if that force is such that his speed remains constant, then there is a component of it that is not radial, and increases the angular velocity of the large disk.