The effects of Lorentz transformation on shape

Question

Imagine a solid 3D cube. Now imagine that this cube is traveling close to the speed of light. To what degree will the spatial geometric properties of this object (or in general of any 3D object) change (as predicted by the Lorentz transformations)?

I assume that despite the changes to shape, some fundamental geometric features of 3D objects are preserved no matter the frame of reference. I assume that a cube will preserve some of its fundamental geometry Namely, a cube undergoing Lorentz contraction will not become a torus or vice-versa. (I might be wrong about this) But what is the formal limit to these changes? Will the affine structure of a 3D object undergoing Lorentz contractions change or would its topology also change?

Answer 1

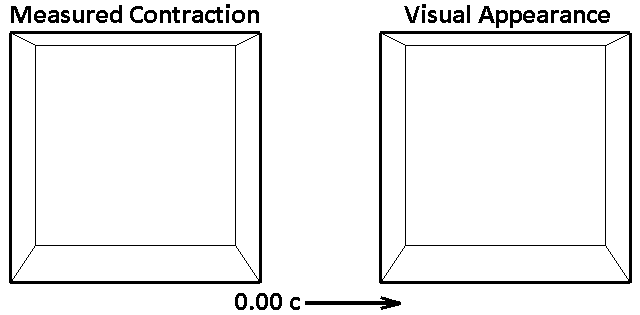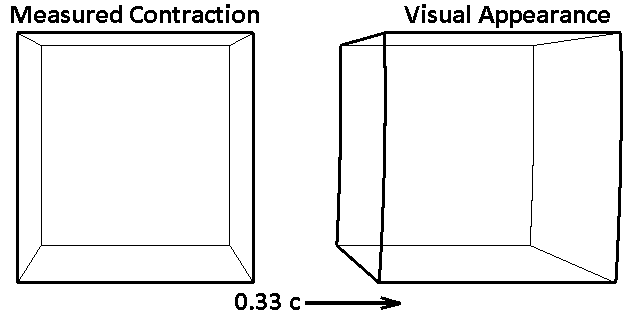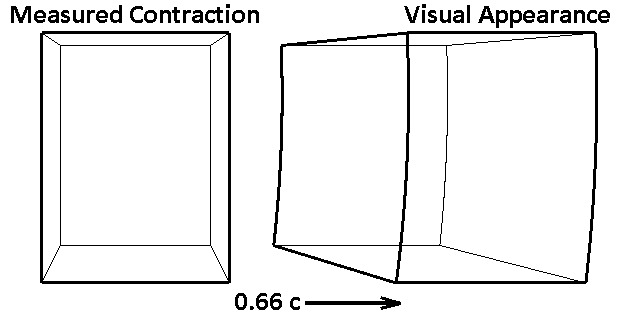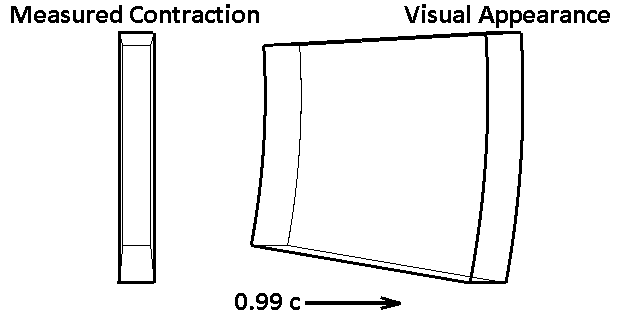
The Lorentz contraction is a statement about how a given object, which should be regarded as a region of spacetime (not just space since the object continues to exist over time), will be determined to have different spatial dimensions depending on which inertial reference frame is being adopted in order to specify how spacetime is to be divided into "space" and "time".

The above opening statement may seem a bit perplexing if you have only met the Lorentz contraction in its simplest form, but in the following you will see why I have written this somewhat more careful and technical statement.

In the simplest scenario, one considers an object which is itself in internal equilibrium (so not vibrating or sloshing internally) and is moving inertially (not accelerating overall) and without any rotation. In this case the Lorentz contraction is a simple linear "squeeze" in one direction (the direction of motion of the object relative to the observer reporting the contraction). The object is not really being "squeezed" by any force, it is simply that it occupies less of space along one direction than it does to another observer. There is no change in topology.

For an object which is accelerating or vibrating or rotating the situation is a good deal more complicated. In order to understand it it is best to adopt the spacetime point of view, which I will illustrate for the case of a flat object (one taking up just two spatial dimensions---consider for example a picture frame, a very flat sports car moving horizontally, a flat rigid steel plate moving in the plane, etc.) In spacetime the flat object persists over time so it traces out a 3-dimensional region of spacetime called a "world tube". Make the time direction be vertical in your picture of spacetime (in your imagination) and then this tube extends in the vertical direction. In the case of a picture frame the tube will have a rectangular cross section. In the case of the steel plate the cross section will be whatever shape the steel plate has. Similarly for the sports car.

When we observe this object, we take it upon ourselves to say "where it is". In other words, we give a statement about the spatial extent of the object: which region of space it occupies at some given time. But how can we do this? Our answer will depend on which part of spacetime we choose to designate as "space". This is done by taking a slice through spacetime. That is, we (some given observer) pick a time (as indicated by our own clock), and cut a plane through spacetime, asserting that all events in this plane take place at the same moment in time. But the direction of this plane will depend on the state of motion of the observer. The plane will be broadly speaking in near-to-horizontal directions on the diagram, but will slope differently for different inertial observers, depending on their state of motion relative to one another. (I am not going to prove this here; we are just doing ordinary special relativity). The region where this plane cuts through the world-tube of the given object (picture frame, steel plate, sports car) is then the spatial region occupied by the object, as determined by the given observer.

You can now see, I hope, that for an object in inertial motion, this sloping slice through will simply change the object's extent in one direction. It appears at first as if the object must get longer when observed by an observer in motion relative to it, but in fact there is also a scale factor which has to be applied to this diagram I have been talking about, and overall one ends up with a contraction not expansion. But the purpose of talking about the spatial "slice" effect is so that you can now see what will happen if the object is accelerating or vibrating or rotating. Things get a lot more interesting!

For an accelerating object, the world tube bends as it goes through spacetime, so now when one slices through the tube, many more possibilities arise. An accelerating banana can be found to be straight (for a moment) for some observer; an accelerating rigid rod can be found to be bent. And if the object is itself in vibration then more possibilities arise. A rotating cylinder can be observed to be twisted. An oscillating picture frame will be observed to have all sorts of interesting shapes.

But in all this the topology will not change. This is because the parts of the body cannot exceed the speed of light relative to one another, and neither can an observer exceed the speed of light relative to the body. This means that the bodies' world-tube cannot tip over beyond 45 degrees on our diagram, and the observer's spatial slices cannot tip up or down beyond 45 degrees on the diagram. So the spatial slice never manages to find a hole in the body where one was not there in some other reference frame. It's a bit complicated to really convince oneself of that but I think it is true.

Finally, note that the Lorentz contraction effects we have discussed are not to do with the body itself. They are just a result of the way different observers choose to adopt different planes in spacetime as defining what they mean by the word "now". The fact that different observers must, logically, make different definitions of simultaneity was the great contribution of Einstein's 1905 paper. To prove it, for anyone not already familiar with the proof, I recommend a good introductory text on special relativity (and I have written a couple).

Answer 2

The object will only contract by a factor of

$$V'=\frac{V}{\gamma}=V\sqrt{1-\frac{v^2}{c^2}}$$

where $V'$ is the measurement* made from our "stationary frame" (from which the object's frame is moving at relative velocity $v$) and $V$ is the measurement made from the object's rest frame.

^$*$ While $V$ usually indicated Volume, the same relationship also holds for length and areas; see How does the area of moving circle change?

For an object to change it's shape fundamentally, one would need to have different contraction or stretching at different points on the object. However, since length contraction only means, well, contraction which is the same across the whole object, the fundamental shape cannot change.

The object will simply contract in the direction it's moving.

---

As mentioned by Joshua Lin, there is another effect called Terrell rotation that occurs when observing objects moving at relativistic velocities. Since the speed of light is finite and thus the light from different parts of an object reaches the observer at different times,

a receding object would appear contracted, an approaching object would appear elongated (even under special relativity) and the geometry of a passing object would appear skewed, as if rotated

This is shown in the following animation:

(Source)

It must however be mentioned that Terrell Rotation is only an observational change (and is thus not "real"), while length contraction actually contracts the object.

Answer 3

Length contraction gives $L' = \frac{L}{\gamma}$. Say the system $S'$ moves at a certain velocity $v_0\hat{x}$, and lets look at the distance between two points:$$d=\left| p_1 - p_2 \right|$$ $$d' = \left|\frac{p_1}{\gamma} - \frac{p_2}{\gamma}\right|=\left| \frac{p_1-p_2}{\gamma}\right|$$ So the relation between two points can't switch with regards to the origin $O'$, if $p_1$ is closer than $p_2$ to the origin it'll remain this way. That is because $\gamma=\frac{1}{\sqrt{1-v^2/c^2}}>0$. So my understanding is that objects won't change the number of "holes"/"gaps" they have, and will only contract. The equation for contraction of a parameterized circle for example with regards to $x$ is: $$x=r\cos(\theta), \quad y = r\sin(\theta)\longrightarrow x'=\frac{ r\cos(\theta)}{\gamma}, \quad y = r\sin(\theta)$$ or with an equation (note the differences in the "contraction term"): $$x^2+y^2=r\rightarrow (\gamma x)^2+y^2=r$$

Answer 4

Your question is ambiguous. You ask whether the shape will "change as predicted by the Lorentz transformation". But for an object in uniform motion, the Lorentz transformation does not predict any change in shape over time; it predicts that the shape will be different (but unchanging) in different frames.

So there are two ways to interpret your question:

1. If the moving object is a cube in its own frame, what shape does it have in the lab frame? (Notice the absence of the word "change".) The other responders seem to have interpreted your question this way and answered accordingly.

2. If the object is initially a cube at rest in the lab frame, and then it accelerates to a high velocity, how will its shape (as measured in the lab frame) change? This interpretation preserves your apparent intention to ask about changes. You can also answer it without knowing anything about relativity: In order to accelerate the object, you've got to apply some forces to it, and those forces can warp the object's shape in any way at all depending on how you apply them.

Answer 5

In the object's rest frame, assume that the geometrical shape of the object is determined by the Cartesian function below:

$$z=f(x,y)\space.$$

Then, from the viewpoint of the observer who moves along the $x$-axis relative to the object, the above function would change into:

$$z'=f(\gamma x',y')\space,$$

where $\gamma$ is the traditional Lorentz factor. This means that the object looks contracted along $x'$.