Calculate Tipping Force of Table [closed]

Question

How do you calculate the force it would take to push over/lean on a table for that table to tip.

The table top is 144" X 40" and weighs 281 lbs. The table is supported by (4) table bases measuring 16"W X 4"D X 32"H and each weighs, lets say 55 lbs. The table is not a permanent fixture and will not be bolted to the floor, so I need to know what it would take to tip if someone were to apply downward force on the edge OR decide to lean up against the table.

Answer 1

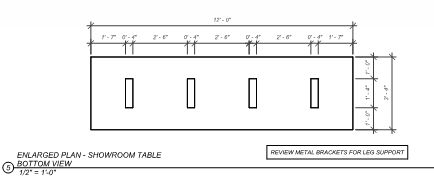
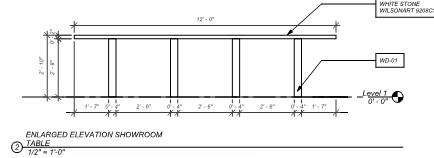
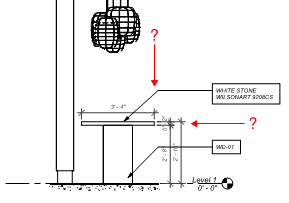
Since $500$ lb is enough to kill someone, you definitely want it not to tip.

The force needed to tip it is a good thought, but might not tell you what you need to know. If you bump it, it isn't obvious how big the force is. Non-slip rubber feet make it easier to tip over than if it was on furniture glides.

So without calculating, a $12$" overhang with a $16$" base makes me nervous.

Another point you might want to consider is that someone might bump the end of the table. That would put a torque on the joints between the bases and the top. They need to be strong so that a bump doesn't break them.

---

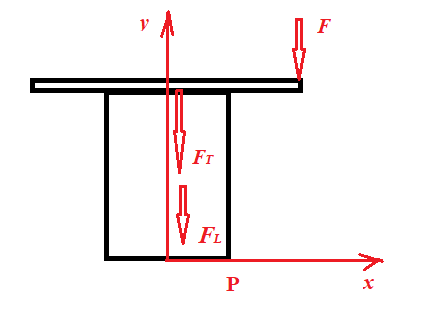
Edit Here are some numbers required to tip it over by putting a weight on the edge. A weight on the edge creates a torque, $\tau_W$, that will try to tip the table by rotating it around the bottom edge of the legs. The weight of the table creates a torque, $\tau_T$, that will prevent tipping. The table will tip when

$$\tau_W > \tau_T$$

Using Gert's notation below,

$$F(\frac{L_T-L_L}{2}) > (F_T + F_L)\frac{L_L}{2}$$

$$F > (F_T + F_L)\frac{L_L}{L_T-L_L}$$

$$= (281 lb + 4*55lb) \frac{16"}{40" - 16"}$$

$$= 334 \space lb$$

So a couple medium large people could tip it over by leaning on it or trying to climb onto it. Or a strong person could tip it by trying to pick it up by one side.

If you widen the base, it quickly gets better. Here are some numbers. I get that the artistic vision is a floating table top with a strong but recessed base. Perhaps your designer would be open to a trapezoidal base. Even with a wider base, the forces on the joint get stronger than you might suppose.

• 16" - 334 lb
• 18" - 364 lb
• 20" - 501 lb
• 22" - 612 lb

Also consider the sideways push to tip over a top heavy table with a tall narrow base can be small. If h is the height of the table,

$$\tau_S > \tau_T$$

$$Fh > (F_T + F_L)\frac{L_L}{2}$$

$$F > (F_T + F_L)\frac{L_L}{2h}$$

$$= (281 lb + 4*55lb) \frac{16"}{2 * 32"}$$

$$= 125 \space lb$$

You know someone will try to move a $500$ lb table by pushing it. Probably with someone one the other side helping by pulling.

Again with wider bases

• 16" - 125 lb
• 18" - 140 lb
• 20" - 158 lb
• 22" - 172 lb

This isn't so easy to fix. At least with a wider base, you have a better chance of stopping the push before you tip it all the way over. But 22" is not near good enough.

If this does get built, you should try as hard as you can to tip it over. If you succeed, you have proved it isn't safe. If you fail, you haven't proved it. But neither have you proved it safe. I live in California. I hate to imagine a 500 lb table dancing around in an earthquake.

Some of this sounds far fetched. But the table weighs as much as a candy vending machine. Those are bolted down now because people got annoyed when the candy didn't drop all the way. They tried to tip the machines. Some machines are on marine bases. Some marines are strong enough to pull the machines over and kill themselves.

Answer 2

Firstly, I assume there's good friction between the table's legs and the floor, so slipping isn't an option.

Define:

• $F_T$ table top's weight
• $F_L$ table top's legs total weight
• $F$ tipping force
• $L_T$ table top's total width
• $L_L$ table leg's total width

The balance of torques about the point $P$ is:

$$F\frac{L_T}{2}=F_T\frac{L_L}{2}+F_L\frac{L_L}{2}$$

Tipping occurs when: $$F>(F_T+F_L)\frac{L_L}{L_T}$$

So the danger of tipping depends mainly on:

$$\frac{L_L}{L_T}$$