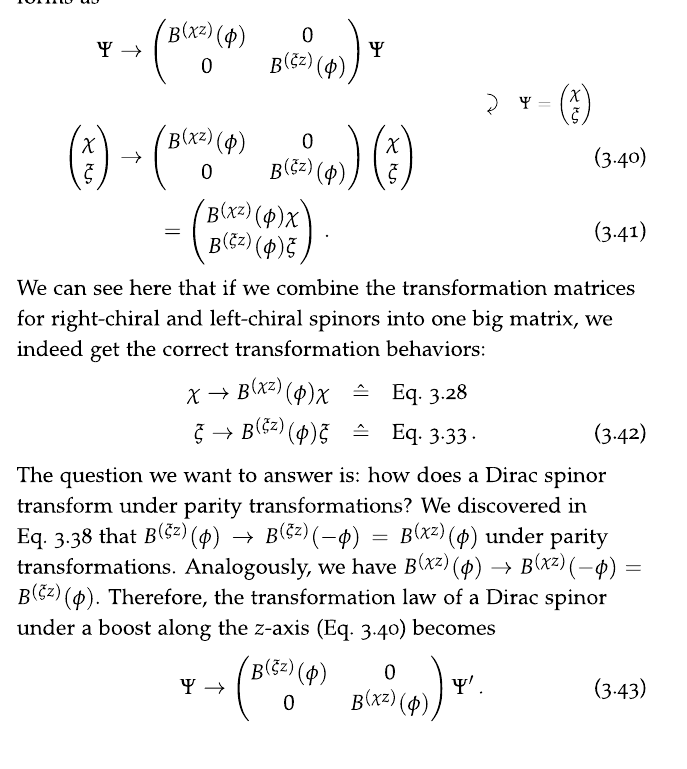
I'm reading "No-Nonsense quantum field theory" and I have some doubts about the transformation law for Dirac Spinors as explained by the author. In the book the left chiral spinors $\chi$ and right chiral spinors $\xi$ are introduced as objects that have two components and behave under rotations $R$ around $x$-axis and boosts along $z$-axis $B$ as follows:
$$\chi_a \rightarrow R_{ab}^{(\chi x)} \chi_b \\ \chi_a \rightarrow B_{ab}^{(\chi z)} \chi_b $$
where $$R_{ab}^{\chi z} = \begin{pmatrix} \cos(\theta/2) & i\sin(\theta/2)\\\ i\sin(\theta/2) & \cos(\theta/2)\end{pmatrix} \\ \\ B_{ab}^{(\chi z)} = \begin{pmatrix} e^{\phi/2} & 0\\\ 0 & e^{-\phi/2}\end{pmatrix}$$
and
$$\xi_a \rightarrow R_{ab}^{(\xi x)} \xi_b \\ \xi_a \rightarrow B_{ab}^{(\xi z)} \xi_b $$
where $$R_{ab}^{\xi z} = \begin{pmatrix} \cos(\theta/2) & i\sin(\theta/2)\\\ i\sin(\theta/2) & \cos(\theta/2)\end{pmatrix} \\ \\ B_{ab}^{(\xi z)} = \begin{pmatrix} e^{-\phi/2} & 0\\\ 0 & e^{\phi/2}\end{pmatrix}$$
Then the author introduces the Dirac spinor: $$\Psi = (\chi, \xi)^T$$ which tranforms under boosts as
$$(\chi, \xi)^T \rightarrow \begin{pmatrix} B^{(\chi z)} (\phi) & 0\\\ 0 & B^{(\xi z)} (\phi)\end{pmatrix} (\chi, \xi)^T$$. So far I'm following the argument, but then the author claims the equation just above becomes:
$$(\chi, \xi)^T \rightarrow \begin{pmatrix} B^{(\xi z)} (\phi) & 0\\\ 0 & B^{(\chi z)} (\phi)\end{pmatrix} (\xi, \chi)^T$$ because under parity transformation we have $B^{(\xi z)} (\phi) \rightarrow B^{(\xi z)} (-\phi) = B^{(\chi z)} (\phi)$ and $B^{(\chi z)} (\phi) \rightarrow B^{(\chi z)} (-\phi) = B^{(\xi z)} (\phi)$. And then asserts that this implies that the Dirac Spinor $\Psi$ transforms under parity transformations as $$ \Psi = (\chi, \xi)^T \rightarrow (\xi, \chi)^T$$ I'm confused about why the last statement follows from the discussion above. I've also attached a picture of the section of the book where I got this from: