Wave equations for two intervals at Potential step

Question

Lets say we have a potential step as in the picture:

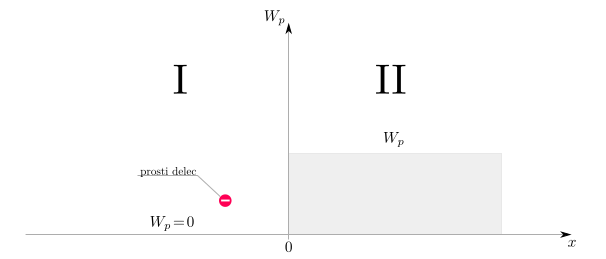
In the region I there is a free particle with a wavefunction $\psi_I$ while in the region II the wave function will be $\psi_{II}$.

Let me take now the schrödinger equation and try to derive $\psi_I$:

\begin{align} &~~W \psi = -\frac{\hbar^2}{2m}\, \frac{d^2 \Psi}{d\, x^2} + W_p \psi ~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~\nonumber \\ &~~W \psi = -\frac{\hbar^2}{2m}\, \frac{d^2 \Psi}{d\, x^2}\nonumber \\ &\frac{d^2 \Psi}{d\, x^2} = -\frac{2m W}{\hbar^2}\,\psi \nonumber\\ {\scriptsize \text{DE: }} &\boxed{\frac{d^2 \Psi}{d\, x^2} = -\mathcal L\,\psi}~\boxed{\mathcal{L} \equiv \sqrt{\tfrac{2mW}{\hbar^2}}} \nonumber\\ &~\phantom{\line(1,0){18.3}}\Downarrow \nonumber\\ {\scriptsize \text{general solution of DE: }} &\boxed{\psi_{I} = C \sin\left(\mathcal{L}\, x \right) + D \cos \left(\mathcal{L}\, x \right)}\nonumber \end{align}

I got the general solution for the interval I, but this is nothing like the solution they use in all the books: $\psi_{I} = C e^{i\mathcal L x} + D e^{-i \mathcal L x} $ where $\mathcal L \equiv \sqrt{{\scriptsize 2mW/\hbar^2}}$. I have a personal issue with this because if $x= -\infty$ part $De^{-i \mathcal L x}$ would become infinite and this is impossible for a wavefunction! I know that i would get exponential form if i defined constant $\mathcal L$ a bit differently as i did above:

\begin{align} {\scriptsize \text{DE: }} &\boxed{\frac{d^2 \Psi}{d\, x^2} = \mathcal L\,\psi}~\boxed{\mathcal{L} \equiv -\sqrt{\tfrac{2mW}{\hbar^2}}} \nonumber\\ &~\phantom{\line(1,0){18.3}}\Downarrow \nonumber\\ {\scriptsize \text{general solution of DE: }} &\boxed{ \psi_{I} = C e^{\mathcal L x } + D^{-\mathcal L x} }\nonumber \end{align}

This general solution looks more like the one they use in the books but it lacks an imaginary $i$ and $\mathcal L$ is defined with a - while in all the books it is positive. Could anyone tell me what am i missing here so i could understand this?

Answer 1

The solution is $A\sin(ax) + B\cos(ax)$ when $\psi'' = -(a^2\psi)$

but $Ce^{bx} + De^{-bx}$ when $\psi'' = (b^2\psi)$ where $b=ia$

There derivatives may be same but they themselves are not the same and but do give the same wavefunction and these solutions cannot be shown to be same mathematically unless you differentiate both the sides to show their eigenvalues are the same.

But we can impose some rules because both are must give the same wavefunction so we can show a correlation

$\psi(0)_{ exponentialsoln. } = \psi(0)_{cos \& sin soln.}$

$Ce^0 + De^0 = B$