Calculation of the oscillation frequency of a rotating system that performs small oscillations

Question

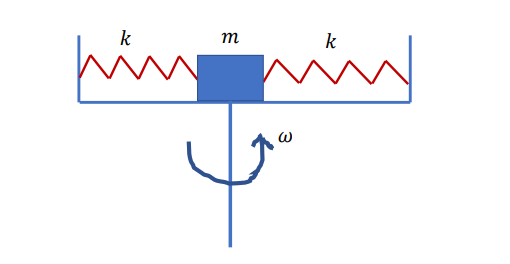
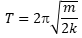I'm currently learning physics and sometimes I don't know how to approach a problem. I ran into this problem with oscillations combined with a circular motion, and I can't really find the answer.

Problem:

An object of mass 𝑚 is placed in the configuration (on a tray) below between two identical springs. The whole system rotates around an axis that passes through the middle of the tray with angular speed 𝜔. Does this system perform small oscillations? If it does, what are the oscillation frequency and its equilibrium position?

I started my demonstration with the following statement: If the body is in the equilibrium position, then there will be no small oscillations.

But since it's not specified, there comes to mind the idea of the body NOT being in the equilibrium position. I assume it will oscillate because there will be a return force proportional to the distance from the eq. position.

I thought about the case where the system is not rotating:

So the equivalent force would be: 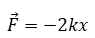and the period of oscillation:

I know that this is an answer to a whole other case, and my feeling is (because I am not sure how to prove it) that the period should depend on the rotation frequency of the tray.

What do I need to do in order to prove that? And what should the answer be in that case?

Answer 1

You state that:

$$\vec{F}=-2kx$$

But one side cannot be a vector and the other not. Either you choose both sides to be scalars ($F=-2kx$) or both sides to be vectors ($\vec{F}=-2k\vec{x}$)

You've also not really specified a coordinate system. I'm guessing you're looking at $m$ in a rotational frame with origin at the centre and an $x$-axis pointing horizontally outward.

You state that the period of oscillation is:

$$T=2\pi \sqrt{\frac{m}{2k}}$$

For a simple harmonic oscillator (SHO) that would be true and it is derived from the Newtonian equation of motion (NEM) of the system:

$$m\ddot{x}=-2kx$$

But your system is slightly different because there's an extra force in play: to keep on a circular track the mass $m$ has to experience a Centripetal Force $F_c$:

$$F_c=mx\omega^2$$

So that the NEM becomes:

$$m\ddot{x}=-2kx+mx\omega^2$$

Or:

$$m\ddot{x}=(-2k+m\omega^2)x$$

Solve this ODE to find $T$.

Answer 2

Since this is a homework question, I will only give a strategy how to approach this problem.

1. You have already found the force by the two springs acting on the mass $m$.
2. Now you need to find the centrifugal force acting on the mass $m$.
3. Add these forces to get the total force.
4. Then apply Newton's second law to get a differential equation.
5. Discuss this differential equation to see if this equation even describes an oscillation or something else. Hint: The answer may be different depending on the values of $m$, $k$ and $\omega$.