We know electromagnetic waves are made of oscillating electric and magnetic fields that can travel at the speed of light without a need for a medium. But, how about matter waves proposed by de Broglie? What are they made of? What is their speed? Do they need a medium to travel?
7
-
1$\begingroup$ Electromagnetic waves ARE electric and magnetic fields that travel at the speed of light. They are not made of anything else than themselves. Electromagnetic waves are perturbations of the electromagnetic field so this last can be thought of as a medium, though it is not really the case. I am afraid there is no real link between electromagnetic waves and de Broglie waves other than the fact that they both are waves. $\endgroup$– Jeanbaptiste RouxCommented Nov 25, 2020 at 12:58
-
$\begingroup$ @JeanbaptisteRoux why have you written answer in a comment? $\endgroup$– UmaxoCommented Nov 25, 2020 at 13:27
-
2$\begingroup$ @Umaxo Because I do not answer the four questions asked, I just corrected some mistakes that were made. $\endgroup$– Jeanbaptiste RouxCommented Nov 25, 2020 at 14:03
-
$\begingroup$ yes but what are matter waves composed of? $\endgroup$– mad112Commented Nov 25, 2020 at 14:09
-
1$\begingroup$ @JeanbaptisteRoux Which should be written as an answer. This sites is to share knowledge and information. It should no be written in a comment $\endgroup$– UmaxoCommented Nov 25, 2020 at 14:31
|
Show 2 more comments
2 Answers
$\begingroup$
$\endgroup$
6
We don't really look at 'matter waves' as... erm... matter waves anymore. The understanding of the concept has moved on considerably since de Broglie (but textbooks tend to lag behind newer ways of thinking) One physicist is alleged to have quipped, a propos 'matter waves': "nothing oscillates there".
Instead it's 'safer' to look at 'matter waves' in the following way.
Suppose we have a subatomic particle. In accordance with Schrödinger's equation a wave function $\Psi(\mathbf{r})$ is associated with the particle.
One of Quantum Mechanics' postulates (possibly the most important one) is that the wave equation contains all the information about the particle there is to know. These observables, like momentum $p$, are obtained by applying quantum operators (e.g. $\hat{p}$) to the wave function.
One of the most important observables is the probability function $P$, which according to the Born interpretation is given by:
$$P(x,\Delta x)=\int_x^{x+\Delta x}\Psi^*\Psi \text{d}x=\int_x^{x+\Delta x}|\Psi|^2 \text{d}x$$
This is for the probability of finding a particle in a $\text{1D}$ domain in a $\Delta x$ interval, located at position $x$.
The probability density function is given by:
$$P(x)=|\Psi(x)|^2$$
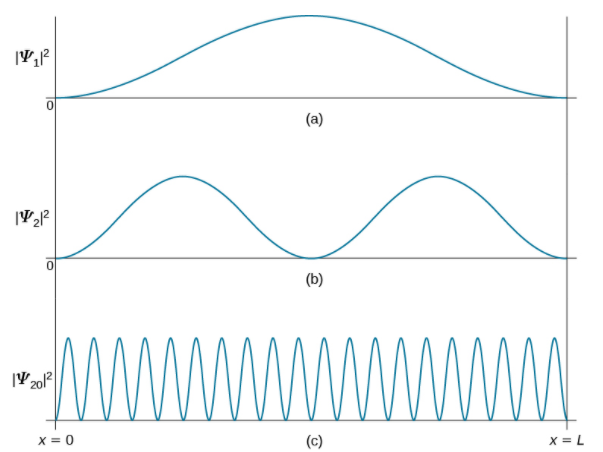
Below are some probability densities for some quantum states of a particle in a $1D$ box with infinite potential on the boundaries:
So what is really tangibly 'wavy' here are these probability densities $P(x)$. Rather than 'matter waves', think of them as probability waves.
These probability waves (and not so much the actual wave functions) explain the interference patterns in two-slit electron beam experiments that were responsible for the emergence of the matter/wave duality worldview and QM itself in the early 20th Century.
There's also the minor issue of the unit of measurement of the wave function. For example for the aforementioned particle in a $\text{1D}$ box the wave functions are given by:
$$\Psi_n(x)=\sqrt{\frac{2}{L}}\sin\frac{n\pi x}{L}$$ For $n=1,2,3,...$
The unit of measurement is:
$$\mathbf{[\Psi]}=\mathbf{m}^{-1/2}$$
There's no mass in sight, as $\mathbf{m}$ here stands for 'meter'.
-
$\begingroup$ Shouldn't the integration be from x to x+∆x? $\endgroup$ Commented Nov 28, 2020 at 19:35
-
-
$\begingroup$ I want to check whether i have understood your answer correct. You are saying that what de Broglie meant was that the probability curves are sinosoidal look alike , that's what matter waves are? $\endgroup$ Commented Nov 28, 2020 at 20:37
-
1$\begingroup$ No, at that time de Broglie and Schrodinger almost certainly believed in the existence of matter waves. But later research/interpretation claims the only 'waviness' are the probabilities (often actually referred to as probability amplitudes) $\endgroup$– GertCommented Nov 28, 2020 at 20:44
-
$\begingroup$ i didn't get a notification for your reply . Nevertheless a great answer as as usual. $\endgroup$ Commented Nov 30, 2020 at 19:47
$\begingroup$
$\endgroup$
1
E-M waves are not "made" of anything other than the current state of various fields. Matter waves are similarly not "made" of anything; they simply represent the wavelike behavior of things we treat as solid mass, e.g. electrons. The whole point of wave-particle duality is that you cannot separate one from the other.
answered Nov 25, 2020 at 15:14
-
1$\begingroup$ except that for subatomic particles what is waving is the probability of finding the particle at (x,y,z,t), not the energy/mass of the particle .over (x,y,z.t) as with classical elecromagnetic waves, and sound waves .... $\endgroup$– anna vCommented Nov 26, 2020 at 5:21