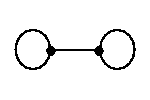
Consider the dumbbell graph decorated with propagator $P$ one the edges and with integration variables $x$ and $y$ on the vertices. We associate to it the following integral: $$ I = \int_{x,y} P(x,x)P(x,y)P(y,y). $$ Does $I$ appear in BRST gauge fixed perturbative Chern-Simons theory (for some gauge group, which one?) on a $3$-manifold $M$ for which $P = d^*G$ is the codifferential of the Green kernel and $x$, $y\in M$? (Note that $P$ is a distributional $2$-form on $M\times M$.) How is $I$ even defined? The propagator $P$ is namely singular on the diagonal and the expression $P(x,x)$ does not make any sense. Thanks!
