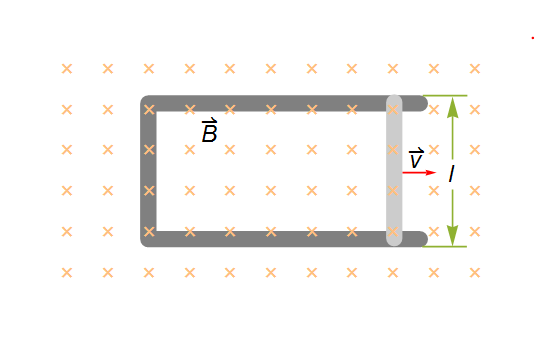
Consider the following situation:
We know this is a classic example frequently used when teaching Faraday’s Law, and the voltage/electromotive force (emf) $\mathcal{E}$ induced across terminals of the moving conductor (lighter gray bar in the picture) is
$$\mathcal{E} = B l v$$
where $B$ is the uniform magnetic field perpendicular to the area, $l$ is the length of the conductor and $v$ is the speed of conductor relative to the field. So far so good, but…
How can I deduce the formula by directly solving Maxwell's equations $$\begin{aligned} \nabla \cdot \mathbf{D} &= \rho_\text{f}\\ \nabla \cdot \mathbf{B} &= 0\\ \nabla \times \mathbf{E} &= -\frac{\partial \mathbf{B}} {\partial t}\\ \nabla \times \mathbf{H} &= \mathbf{J}_\text{f} + \frac{\partial \mathbf{D}} {\partial t} \end{aligned}$$ ?
By directly I mean calculate the electric field $\mathbf{E}$ first (by solving Maxwell's equations with proper initial conditions (i.c.) and boundary conditions (b.c.), using techniques taught in partial differential equation (PDE) course), and then calculate emf using the definition
$$\mathcal{E}=\oint_{C} \mathbf{E} \cdot \mathrm{d} \boldsymbol{ l }$$