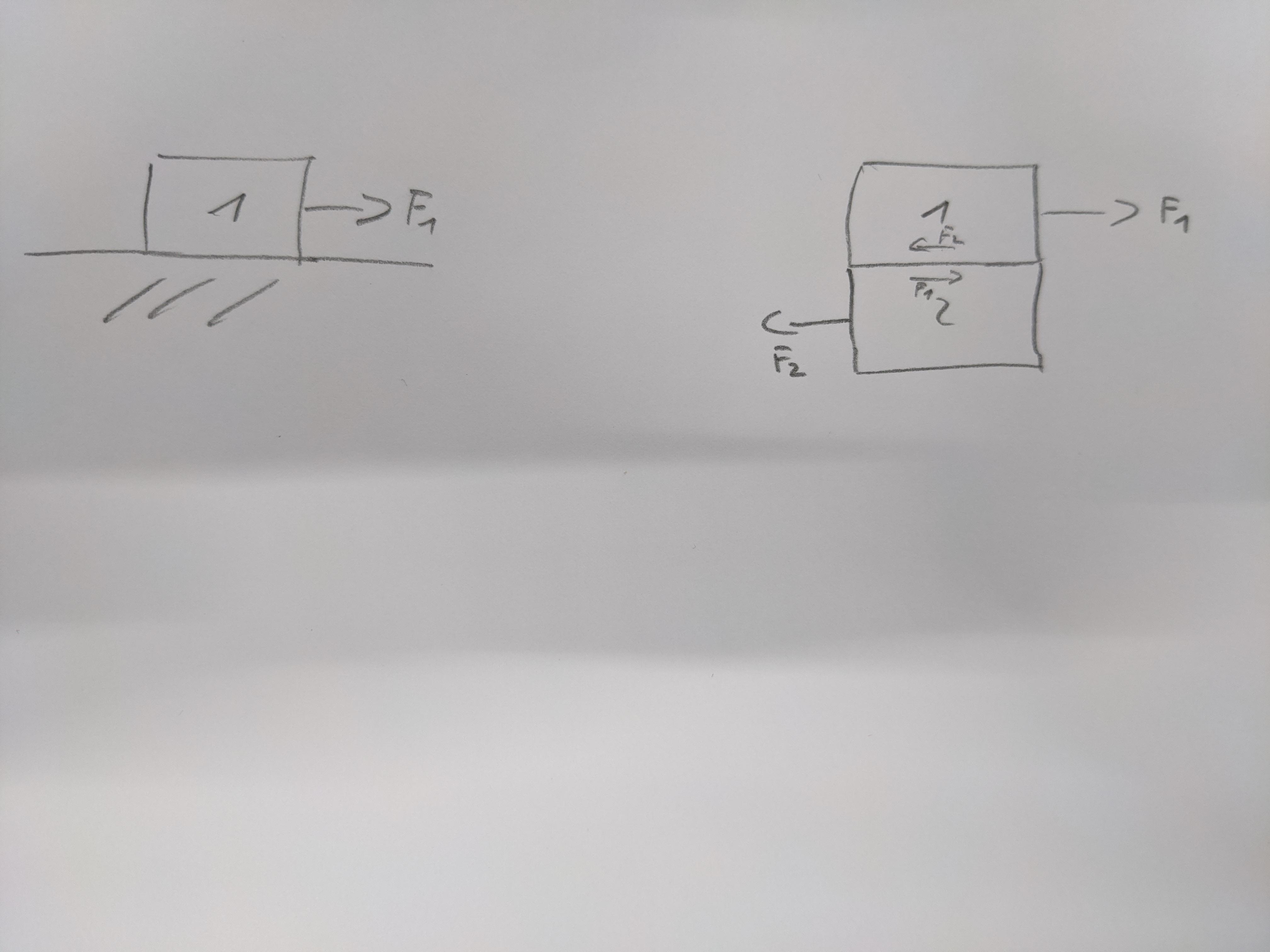
When a body is lying on the floor, the following is true.
The maximum static friction is Frmax=μ*Fn, where Fn is the normal force and μ the static friction coefficient.
If a force F1 is applied to the body in a first direction, the body will move if F1 is bigger than Frmax.
However, what happens if the body is not lying on the floor but on another body that is being forced in the opposite direction?
In which case does a movement between the bodies occur?
F1 + F2 > Frmax
F1 or F2 > Frmax
|F1 - F2| > Frmax?
As long as static friction is present F1 and F2 will be transmitted to the respective other body and cancel each other. But is this transmission relevant for overcoming the static friction?
Fr = F1-F2 (if Fr is defined parallel to F1) So it should be the third formula in my opinion. But that feels wrong. Why would it be harder to push something off a table if someone is trying to move the table at the same time? Additionally, when using superposition, there would then be a partial friciton greater than Frmax.
Bonus question: Why is every website, book, whatever that I find always looking only at one force? Is this question that trivial or that irrelevant?