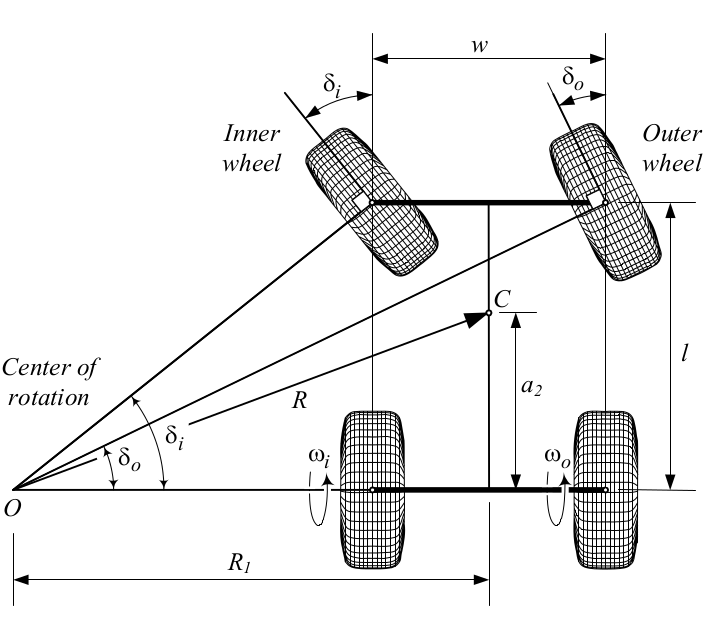
The automobile steered by an Ackerman mechanism has the schematics below. According to the author (Reza N Sazar, Vehicle Dynamics: Theory and Application), the steering angles must not violate the relation below.
\begin{equation} \cot{\delta_o} - \cot{\delta_i} = \frac{w}{L} \end{equation}
However, the book is not explicit about the relation between $\omega_i$ and $\omega_o$. I would like to request some help from you fellows. Any help is sincerely thanked.
those two wheels are rotating on the same axle, but at different radii. This means that if the axle makes one complete turn or revolution around one of its ends, the wheels will trace out different circumferences as they roll on the ground, and their angular velocities will be different. The innermost wheel will trace out a smaller circumference and rotate at a slower speed than the outermost wheel, which will trace out a larger circumference and rotate at a higher speed while doing so.
apply coefficient to your vehicle speed
coefficient has a relation on left steering wheel to your rear wheel speed
W - pivot point width here can be track width;
L - Wheel Base
