1
$\begingroup$

The current density equation states that $J = I/A$.

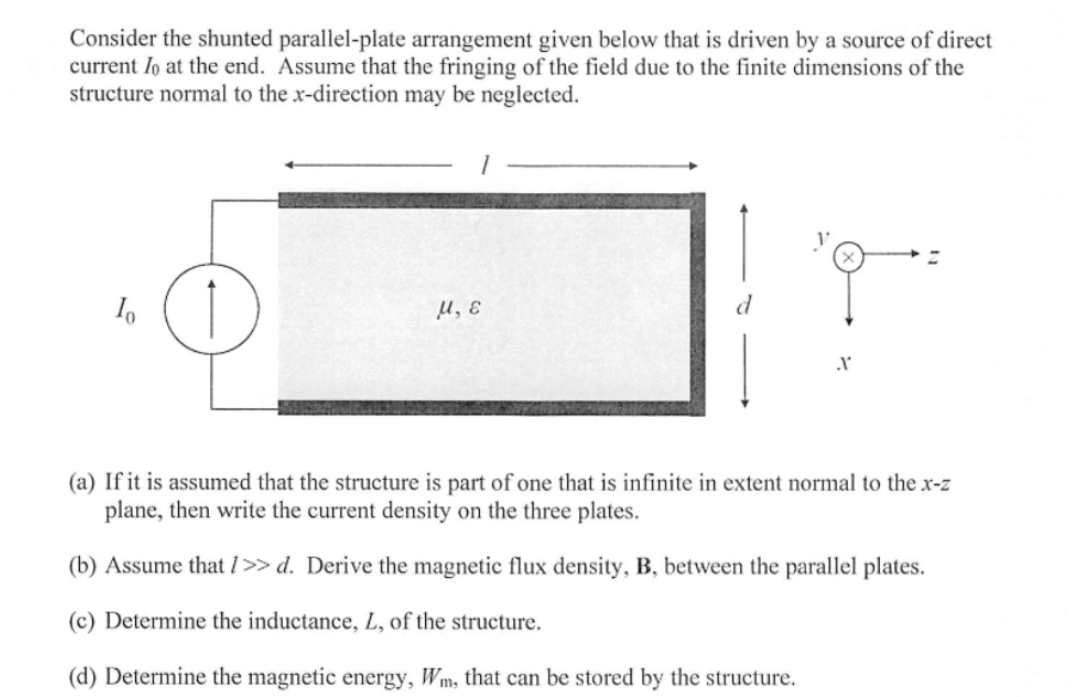
But I got a question that asking the current density over an infinite plate. Say the current $I_0$ flows into the infinite plate that extent infinitely on $z$ direction from $x = 0$ to $d$, What's the current density on the plate? The plate in unspecified on $y$.

I have asked a professor regarding this, and the answer is $J = I$. I have trouble to understand why infinite count as 1 in this case. Could anyone sent some help?

Thanks in advance.

edit:

Here is the question, question (a). enter image description here

Improve this question
$\endgroup$
6
  • $\begingroup$ Related: Surface current density confusion $\endgroup$
    – G. Smith
    Commented Aug 21, 2020 at 23:04
  • 1
    $\begingroup$ I have asked a professor regarding this, and the answer is J = I. Don’t ask that professor any more questions about EM. $\endgroup$
    – G. Smith
    Commented Aug 21, 2020 at 23:05
  • $\begingroup$ Thank you for your reply. .I still don`t get the answer. How can I write the current density? $\endgroup$
    – Chtholly
    Commented Aug 21, 2020 at 23:59
  • 1
    $\begingroup$ It seems to me that it’s zero times a delta function. The situation you have described is sufficiently strange than I am skeptical it is really what the problem asked. Can you please edit your question to quote the problem exactly as it is stated? $\endgroup$
    – G. Smith
    Commented Aug 22, 2020 at 0:20
  • $\begingroup$ I have added the original question. I think those problems are pretty straightforward except (a). Maybe I have a misunderstanding here. $\endgroup$
    – Chtholly
    Commented Aug 22, 2020 at 0:37

1 Answer 1

Reset to default
0
$\begingroup$

The question is given in a rather vague language. The interpretation that the question is demanding is probably the following:

Instead of thinking about I as the total current that is spreading in the infinite area , you could imagine I being supplied to each unit strip where the strips are taken perpendicular to the $x-z$ plane.That is to mean that the source is providing I to all such strips extending in the $y$ direction and hence the net current through the whole sheet is infinite while current density is I itself.

Improve this answer
$\endgroup$
2
  • $\begingroup$ If that`s the case, then (b),(c) and(d) would become infinite. $\endgroup$
    – Chtholly
    Commented Aug 22, 2020 at 18:10
  • $\begingroup$ Yes. You are right , I didn't even look at the other sub-parts. You might have to consider what G.Smith has said. Maybe the question is requiring to neglect the infinite extension. $\endgroup$
    – Lost
    Commented Aug 22, 2020 at 18:27

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.