2
$\begingroup$

For a quantum harmonic oscillator in a coherent superposition, what happens if the energy is measured? Would it collapse to an energy eigenstate (a single excitation) corresponding to the result of the measurement, thus destroying the coherence? Similarly, for a quantum field in a coherent state (classical EM field wave), does the entire field configuration collapse to a single eigenstate after the field is measured? Wouldn't this destroy the coherence?

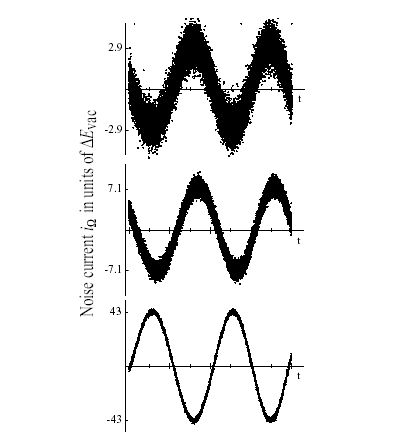
EDIT: I do realize that it should collapse, but I'm not sure how to interpret pictures of coherent state measurements, such as this one from Wikipedia ("Coherent State" article):

Coherent electric field measurement

Shouldn't the coherent state collapse after the very first measurement (at t=0), thus destroying the "classical wave" pattern for the rest of the measurements? Yet it looks classical in this picture...

Improve this question
$\endgroup$
4
  • $\begingroup$ The wikipedia explains this measurement was made using a technique called Homodyne detection. And this are measurements of the electric field. For sure an energy measurement would destroy coherence, but not necessarily you need to maintain coherence in order to measure something about the coherent state. Maybe the measurement was made with many many waves and each one of them the coherent state was destroyed, or maybe something else were measured that didn't destroy coherence. I'm sorry but I don't know enough about this experimental technique in order to explain what's going on. $\endgroup$
    – PedroDM
    Commented Jul 23, 2020 at 17:27
  • $\begingroup$ But I do hope my comment will make you realize that there are many ways of making a measurement and you don't necessarily need to maintain the coherent state intact for that. $\endgroup$
    – PedroDM
    Commented Jul 23, 2020 at 17:28
  • $\begingroup$ nobody is suggesting that what you’re seeing on the screen is a single system on which measurements have been made... $\endgroup$
    – ZeroTheHero
    Commented Jul 23, 2020 at 19:13
  • $\begingroup$ "Shouldn't the coherent state collapse after the very first measurement (at t=0), thus destroying the "classical wave" pattern for the rest of the measurements? " one possible interpretation of this statement seems to imply that. Even if it the OP knows it's not a single system, he does not make that clear, my comment just tried to make that clear. $\endgroup$
    – PedroDM
    Commented Jul 23, 2020 at 19:38

3 Answers 3

Reset to default
1
$\begingroup$

It depends on the type of the interaction. More precisely, on the interaction (part of the) Hamiltonian $H_I$. Usually, the interaction brings the system to the eigenbasis of $H_I$, the so called pointer states. These can be but not need to be the energy eigenstates of the system Hamiltonian. Depending on that, the coherence is fully lost, partially lost or preserved.

Improve this answer
$\endgroup$
1
$\begingroup$

yes it collapses to an energy eigenstate.

Improve this answer
$\endgroup$
0
$\begingroup$

If the wavefunction can collapse for position measurements then why can't coherence be destroyed by energy measurements? It's approximately the same reason.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ I've edited the post to clarify my question... $\endgroup$
    – Dmitry Pugachev
    Commented Jul 23, 2020 at 17:12
  • $\begingroup$ This, arises due to the energy and time uncertainty relationship $\endgroup$
    – Tim Crosby
    Commented Jul 23, 2020 at 17:14

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.