0
$\begingroup$

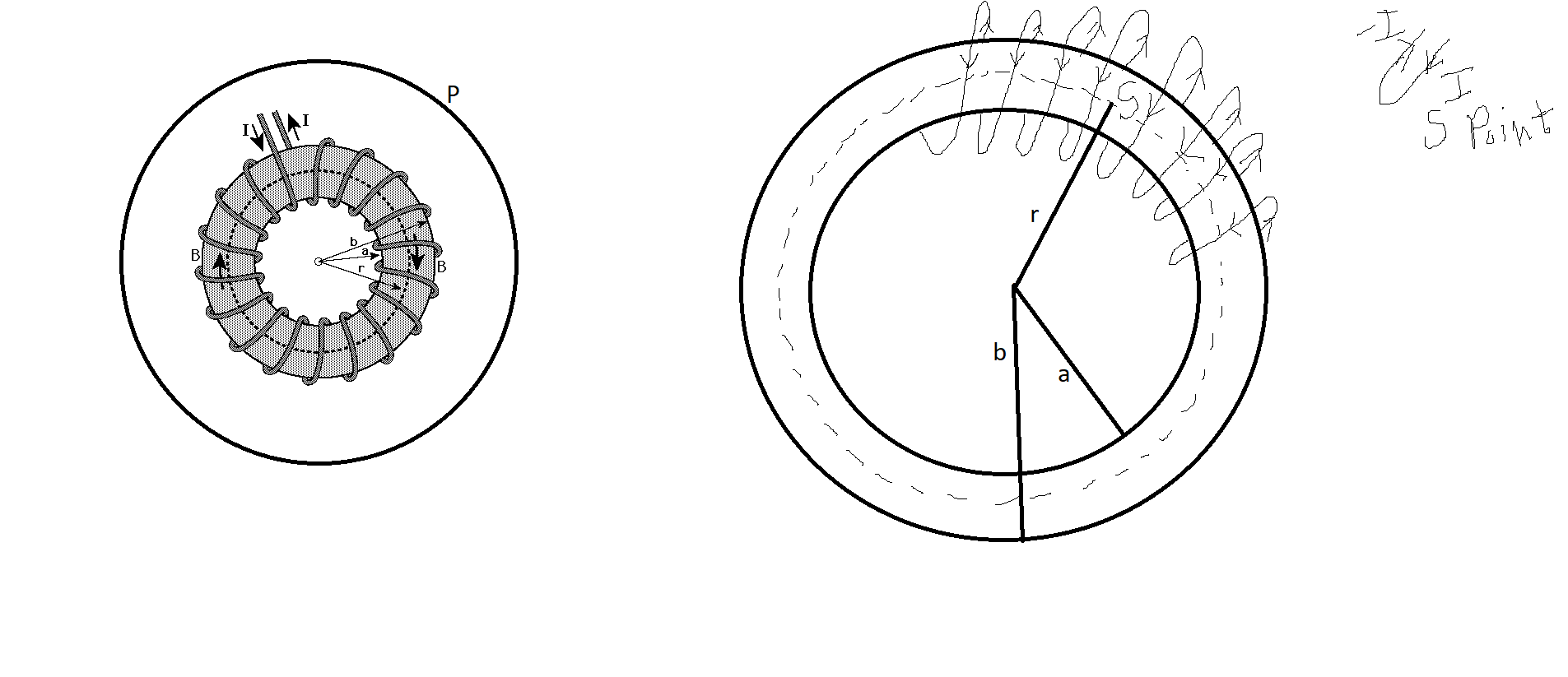
Toroid

Is at point S current entering is not equal to current leaving? I am confused over this concept. Like we have net current zero when we consider a point outside toroid, shown in first diagram - current entering equals current leaving. So why don't currents cancel when we consider a point between radii a and b- of radius r? As I have shown in diagram, current entering r radius circle (outside screen) is equal and opposite of current going inside page (inside screen) (at point S) even when we consider amperian loop in between two radii (not outside or inside). So, by law "net enlclosed" current should be zero but in book we get N times I as total current. Why? According to ampere circuital law, Bdl = uI.

Please explain thoroughly!

Improve this question
$\endgroup$
2
  • $\begingroup$ Your dimensoins 'a', 'b', and 'r' in the right drawing don't match the similarly-designated dimensions in the left drawing. That's going to make it confusing to discuss the question. $\endgroup$
    – The Photon
    Commented Jul 15, 2020 at 1:02
  • $\begingroup$ @The Photon I have corrected that. But main problem is unsolved! $\endgroup$
    – Nikhil Sharma
    Commented Jul 15, 2020 at 8:50

3 Answers 3

Reset to default
0
$\begingroup$

I will give you a simple answer.

Divide the wire in as many pieces as you can. Each current element will produce an element of magnetic field inside the toroid. All magnetic elements are in the same orientation (it is determined by the right-hand rule. Let's say, for instance, that it is clockwise). The magnetic field will not be null simply because the currents do not overlay, and the currents do not cancel each other by the same reason (they do not overlay, and this is why there is still a net flow in the wire).

Now I want to make it clearer. Think about a system of two identical balls. They have the same velocity in opposite directions. The net momentum do cancel each other, because the total momentum in this case is proportional to the sum of velocity vectors (which is null). However, the total kinetic energy is not null, since the kinetic energy is proportional to the square of the modulus of the vectors, so that the energy depends on something else. The magnetic field, in the given example, depends not only in the direction of the currents, but also on the position of the element flow (something else).

Now imagine two straight wires with the same current, but in opposite directions. You could only think about cancelling the currents if the wires are near enough to each other so that the influence of the distance between the wires is sufficiently small. If they are not so near each other, even if the net current is null, the effects produced by the currents separately will not be null (for example, the magnetic field).

Improve this answer
$\endgroup$
3
  • $\begingroup$ But in the case of toroid each winding is close and opposite to another. In one current flows outwards and in another inwards. Current (I) and small element dl are in same direction, so for one the product will be Idl and for another -Idl. (Your argument number 2). $\endgroup$
    – Nikhil Sharma
    Commented Jul 15, 2020 at 11:02
  • $\begingroup$ In the case of toroid each winding is close (Your argument number 3) and opposite to another. As for argument number 1, it's like circular logic that net current exists because that's why there is a current. I want to understand it by Ampere circuital law and considering a loop that law says. Also magnetic field is always in direction of element dl so BdlcosA (A is the angle between B and dl element) can't be zero because angle is not 90 degree- its zero. Why then law opposes reality? $\endgroup$
    – Nikhil Sharma
    Commented Jul 15, 2020 at 11:11
  • $\begingroup$ If you consider a amperian loop containing S or any point on the toroid (solid part not inside or for a<r<b), then you can see clearly that the current entering that surface (outwards) is equal to the current leaving that surface (inwards). So net I enclosed is zero. I know that a magnetic field can be there even when net I enclosed is zero but that's when that angle between B (constant) and dl element is 90 degree but that's not the case here. For me, the net enclosed current will be zero regardless of wherever you choose loop- on toroid or outside. I want to understand it through loop law! $\endgroup$
    – Nikhil Sharma
    Commented Jul 15, 2020 at 11:23
0
$\begingroup$

Your are confused because you did apply correctly Ampere's law:

  1. Identify the amperian loop. In your case, it is the circle of radius r.
  2. Find a surface delimited by the current. In your case, the simplest choice is the disk of radius r.
  3. Orientate the surface. Let's pick as positive side of the disk the one facing us.
  4. Count how many times the current enters your surface by the negative side and leave by the positive side of the surface. In your case, it enters N times.

If you choose an amperian loop with a radius R>b then there are N currents crossing the positive side of the surface and N currents crossing the negative side and the result is zero.

Improve this answer
$\endgroup$
0
$\begingroup$

Imagine the toroid is made of flexible material so that you can cut it and straighten it out so that now you have a straight bar with a current carrying coil wrapped around it. This is the simplest classic electromagnet and the analysis is simple and it is easy to see a magnetic field is generated.

enter image description here

Diagram from Iowa State University together with analysis.

Folding the bar back into a circle does not remove the magnetic field..

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.