Evaluating sum of torques for different choices of origin when solving equilibrium problem

Question

My textbook says,

When applying equilibrium conditions for a rigid body, we are free to choose any point as the origin of the reference frame.

(source)

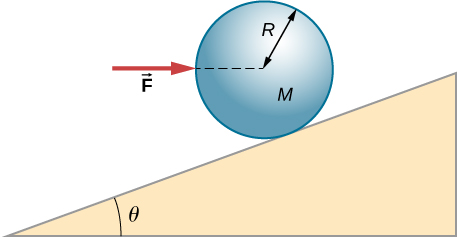
I am trying to understand this by looking at the following picture (from an exercise problem), in which the ball is in static equilibrium because of the applied horizontal force $\vec F$ and the friction between the ball and the surface.

(source)

If we pick the point where the surface and the ball are in contact as the origin, then I can see how the torques cancel out. We have a negative torque $F \cos \theta$ acting on the center of the ball at a distance of $R$ and a positive torque from gravity $ mg \sin \theta$ acting again on the center of the ball at distance $R$. So

$$\sum \tau = 0 = - F R \cos \theta + mg R \sin \theta$$

and we can begin solving for the external force.

Alternatively, let's try defining the center of the ball as the origin. Since the applied force and gravity both act on the center of the ball, they provide zero torque. Likewise, the normal force from the surface is pointed directly at the ball's center and therefore provides zero torque.

The only nonzero torque I can see is provided by the frictional force, whose magnitude is $mg \cos \theta$, and acts at a distance of $R$ in the positive (CCW) direction.

$$\sum \tau' = 0 = \mu_s mg R \cos \theta$$

Since the friction force is nonzero, what force causes the torque to balance when choosing the center of the ball as the origin?

Answer 1

The force of static friction is given by $$|\vec F_f| \le \mu_s |\vec N|$$ Since this is an inequality, you cannot use this to find the magnitude of friction, only whether $\mu_s$ is large enough to provide the necessary friction. Instead, the way to find static friction is based on the fact that it is static, i.e. not moving, so you can just use Newton's Second Law to solve for friction by solving $$\sum \vec F = 0$$

If you solve for the external force using your first equation, you will find that the friction is zero, which is consistent with your second equation.

Answer 2

The friction force is proportional to the normal force, which depends on both gravity and the applied force. Letting the friction force $F_s$ stand on its own, the correct expression for the torque about the origin is

$$\sum \tau' = 0 = F_s$$

From this it follows that the friction force is, in fact, zero.

The same can be seen by taking the torque about the point of contact:

$$\sum \tau = 0 = - F R \cos \theta + mg R \sin \theta \\ \implies F\cos\theta = mr\sin\theta$$

and the sum of force in the $x$-direction (parallel to the slope):

$$\begin{align}\sum F_x =0 &=F_s + F \cos\theta - mg \sin\theta \\ & = F_s + 0\end{align}$$