1
$\begingroup$

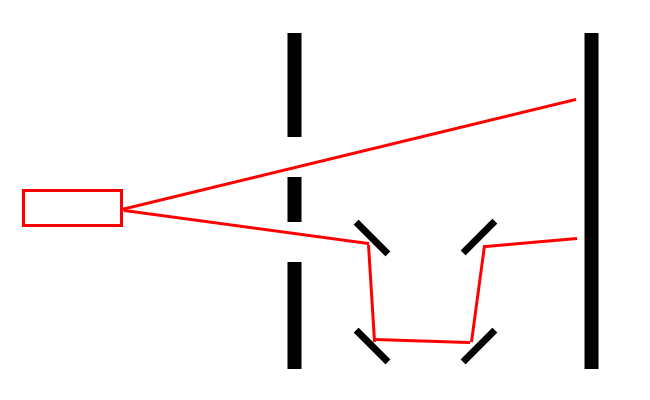
What if we modify the double-slit experiment to have the path between slit $A$ to the board shorter then the path from slit $B$ to the board.

Will we still have an interference pattern? If the answer is yes, do we have a way to calculate path length from the $B$ slit to the board(assuming it's the only unknown)?

enter image description here

Improve this question
$\endgroup$
3
  • $\begingroup$ Given that wave function propagates through both slits there would still be some interference pattern on the first obstacle , i believe. First obstacle is now some sort of a detector. $\endgroup$
    – Žarko Tomičić
    Commented May 24, 2020 at 13:39
  • $\begingroup$ What you propose is a doubled one slit experiment. As I told, even behind edges fringes appear. The best way to verify the answers is to setup this experiment. My prediction: you get intensity distributions behind every slit. $\endgroup$
    – HolgerFiedler
    Commented May 24, 2020 at 18:27
  • $\begingroup$ Where does Ruslan stand with his experimental abilities? (The question about the flame was astonishing.) $\endgroup$
    – HolgerFiedler
    Commented May 25, 2020 at 4:30

3 Answers 3

Reset to default
1
$\begingroup$

Your diagram shows a beam of light going directly to the first mirror. This is not what will happen. Light will act as a wave as it passes through the slits and start to spread out. The mirrors will block most of this wave an diffract the wave on their edges. Some of this expanding wave of light will get passed the mirrors and hit the screen. Because of all of the diffraction going on there will be some “random” interference, but it will be very dim and not convey much info regard the double slit.

Improve this answer
$\endgroup$
-1
$\begingroup$

I would assume you still have an interfernece pattern. At the end, the pattern is caused by phaseshift - which is induced by different path length.

And I think there should be a way to determine the path lenght of part B by since this information is stored in the intensity distribution

Improve this answer
$\endgroup$
1
  • $\begingroup$ Wouldn't there be an ambiguity in determining the path length, e.g. you get constructive interference when the path length difference is one wavelength, two wavelengths, etc? $\endgroup$
    – Not_Einstein
    Commented May 24, 2020 at 14:33
-1
$\begingroup$

If the path length difference is too large, there may not be interference. It depends on the coherence length of the light source. When the light from A and B combines on the screen, they must be coherent in order to interfere.

The two light beams of course have to combine in order to interfere. This is not shown in your diagram.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.