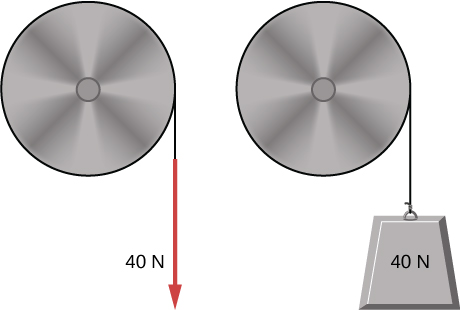
A cord is wrapped around the rim of a solid cylinder of radius $0.25$ m, and a constant force of $40$ N is exerted on the cord shown, as shown in the following figure. The cylinder is mounted on frictionless bearings, and its moment of inertia is $6.0 \mathrm{~kg⋅m}^2$. (a) Use the work energy theorem to calculate the angular velocity of the cylinder after $5.0$ m of cord have been removed. (b) If the $40$-N force is replaced by a $40$-N weight, what is the angular velocity of the cylinder after 5.0 m of cord have unwound?
The answer given is
a. $ω = 8.2$ rad/s; b. $ω = 8.0$ rad/s
In (a), I used
$$\begin{align}W &= 40 \text{ N} \cdot 5\text{ m} = 200 \text{ J}\\ = \Delta K &= \frac{1}{2} I \omega^2 \\ &\implies \omega = 8.16 \text{ rad/s} \end{align}$$
The same result follows from solving
$$\tau = Fr = I \alpha$$
for $\alpha$ and then
$$\bar\alpha \, \Delta \theta = \frac{1}{2}\bar{\omega} ^2$$
(where $\Delta \theta = 5/ .25 = 20$ m) for $\omega$.
Why is the answer to (b) different? What is a $40$-N weight but a source of a constant $40$-N force?
It occurred to me that perhaps in (b) we are supposed to account for gravity as it acts on the wheel itself, but the wheel's center of mass is on the axis of rotation, so the torque due to gravity is zero.
Problem source: https://openstax.org/books/university-physics-volume-1/pages/10-challenge-problems (#125)