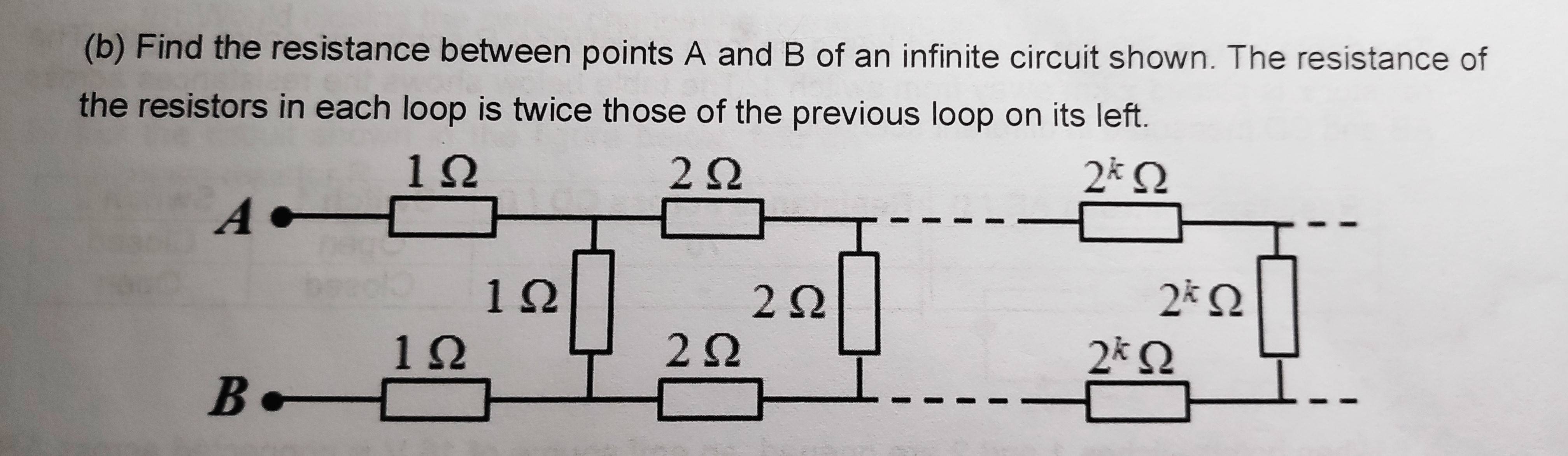
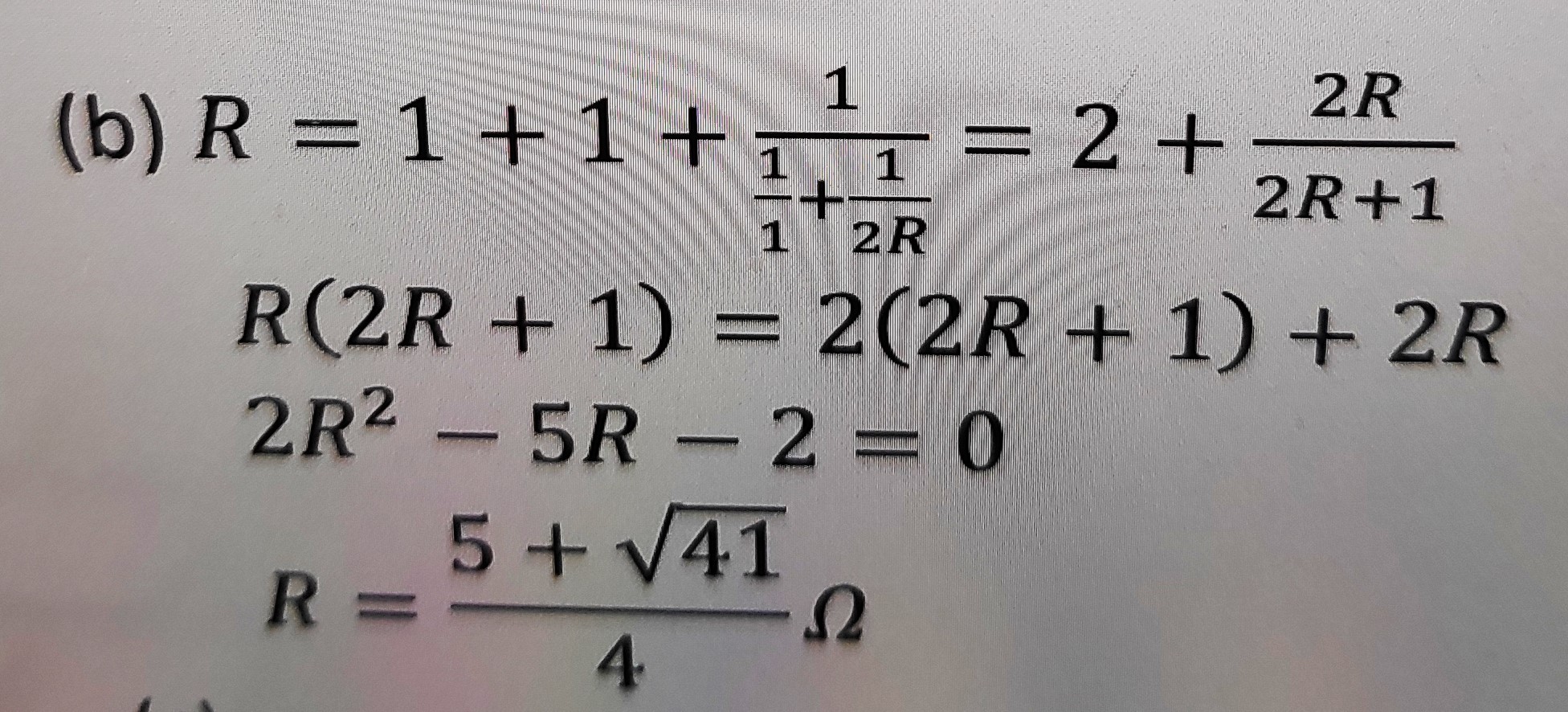-1
$\begingroup$

Here is the infinite series[![][1]]2

Here is the question

My solution is wrong because the denominator of the fraction in the first equation is $1/R$ when in the correct answer, you can see it is $1/2R$. I can't seem to find out why or how they obtained $1/2R$ though, since the effective resistance is infinite.

Here is the solution

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

R is the resistance between A and B. If you imagine removing the three 1 Ohm resistances and measuring the resistance before the 2 Ohm resistances, you have exactly the same configuration but with all the resistance values doubled, so 2R.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thank you!! I see what you mean, so the sum of this second infinite series is technically double of the first, helped me a lot :) $\endgroup$
    – Lucas Tan
    Commented May 9, 2020 at 8:37

Not the answer you're looking for? Browse other questions tagged or ask your own question.