1
$\begingroup$

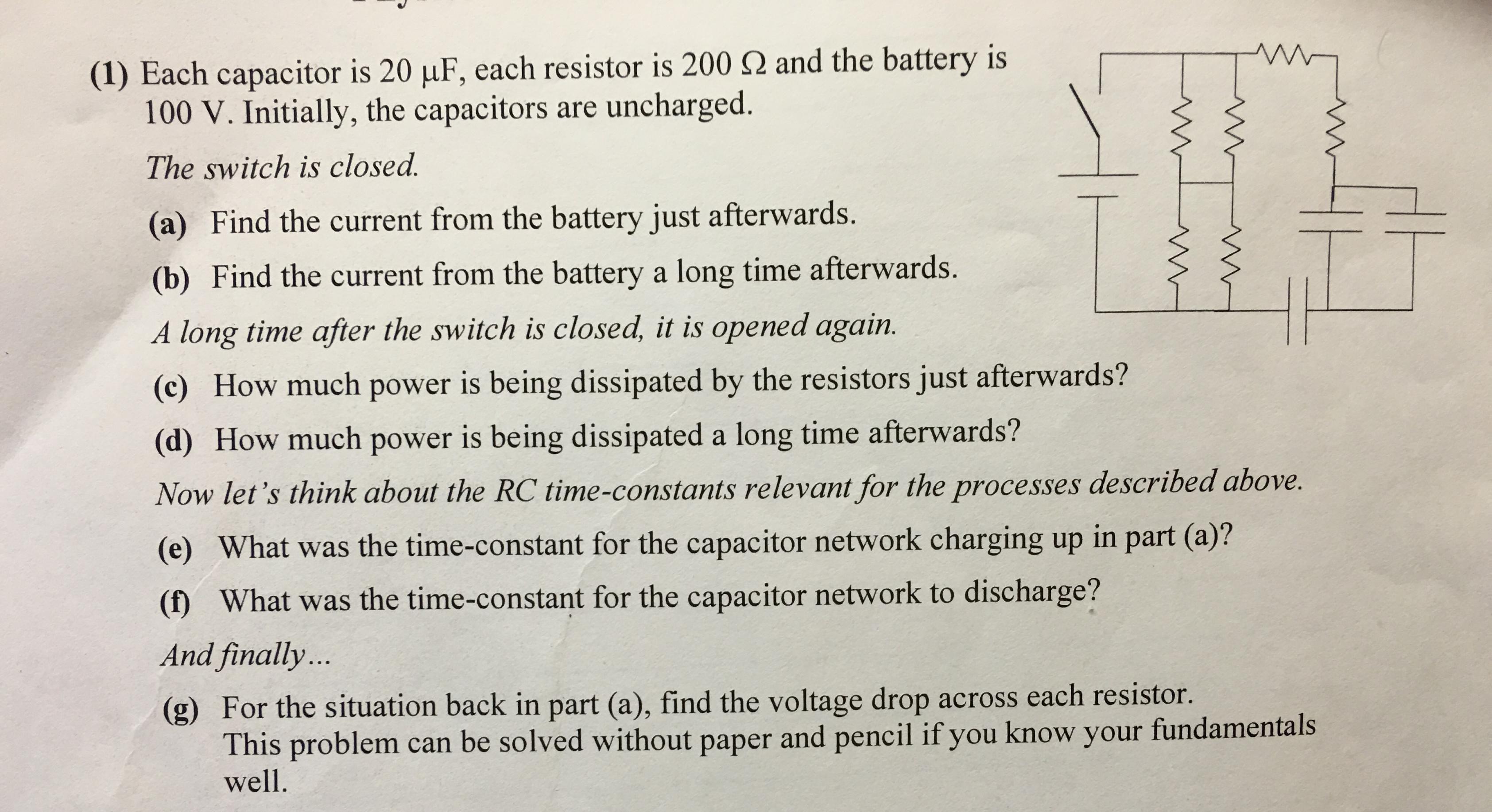
enter image description here

Hi, I am confused on how to find the time-constant for part E. I calculated the capacitance to be 13.3 microfarads and the resistance to be 133.33 ohms (my work is shown below labeled as C), but multiplying that did not give the correct answer of 5.3 ns. In the solution, they only used 200 as the resistance, and I don't understand how they got that because aren't capacitance and resistance in the time constant equation just the equivalent capacitance and resistance of the circuit? If anyone could explain that to me, it'd be really appreciated. Thanks! 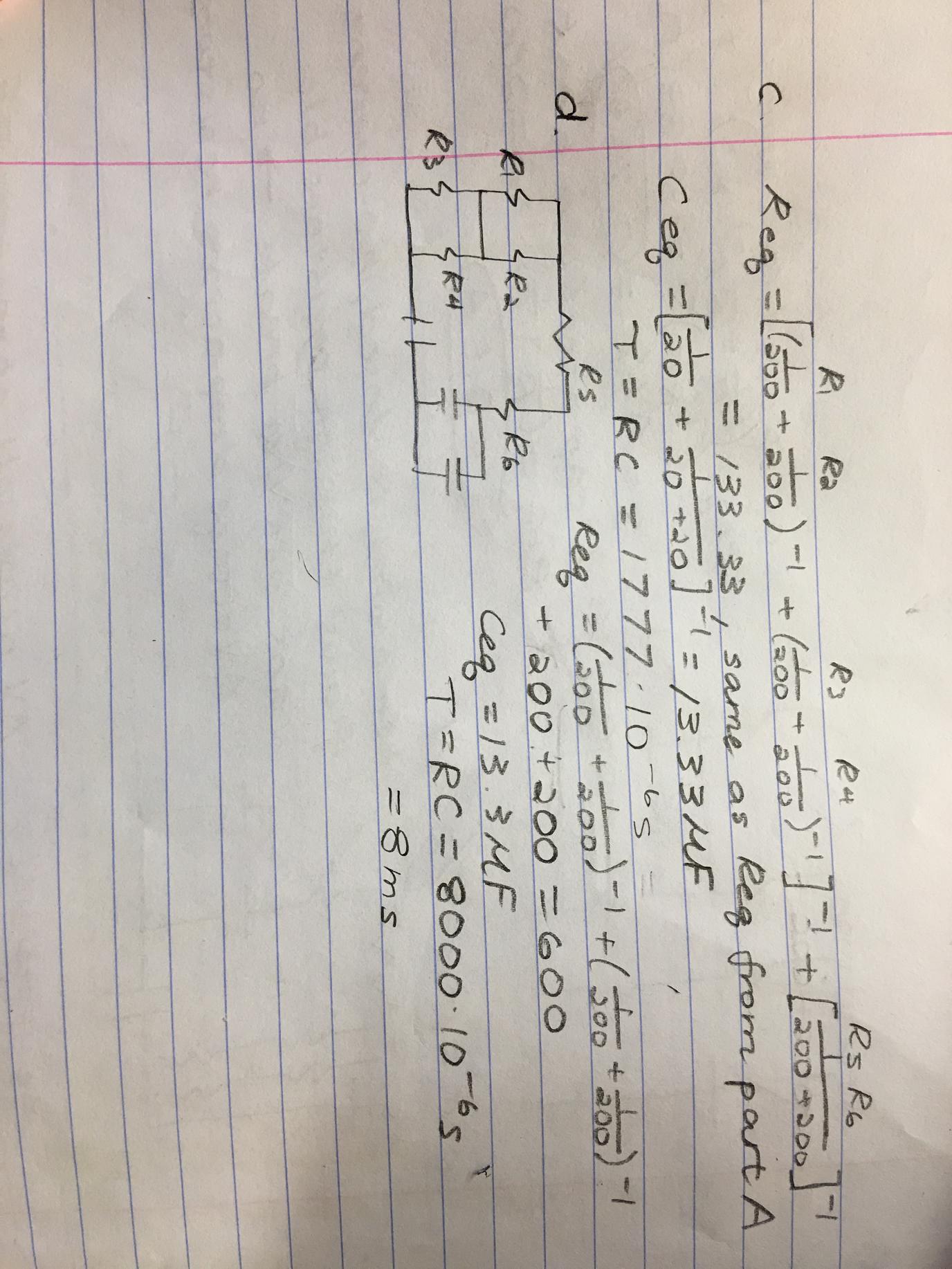enter image description here

Improve this question
$\endgroup$
1
  • $\begingroup$ Laplace transform and node analysis or mesh analysis is my favorite approach to solving circuits. $\endgroup$
    – Emil
    Commented Dec 3, 2017 at 9:37

2 Answers 2

Reset to default
2
$\begingroup$

Revised Answer

It is almost always an advantage to draw a simpler equivalent circuit then calculate from that.

The 3 capacitors can be combined into one equivalent capacitor $C_0$ using the series and parallel combination rules. You have done that and your calculation is correct.

The resistor and voltage-source network can be replaced with an equivalent circuit, consisting of a voltage source $V_{th}$ and resistor $R_{th}$ in series, using Thevenin's Theorem.

To apply this theorem, take the terminals AB as being those across the equivalent capacitor $C_0$. The equivalent resistance $R_{th}$ is that obtained across AB in your network after shorting all ideal voltage sources. The double-parallel resistors are then "shorted out", so $R_{th}=2R$ where $R$ is the value of each identical resistor.

The time constant for the circuit is $R_{th}C_0$.

(What I wrote about there being two different time constants, one for charging and one for discharging was incorrect. There is only one time constant. The resistances in the branch of the circuit parallel to the series RC branch also make a difference, and cannot be ignored.)

The equivalent voltage $V_{th}$ is the open circuit voltage across the terminals AB of the equivalent capacitor $C_0$. In this case it is 100V. So $C_0$ will charge to 100V.

References :

RC Circuit , Calculate Time Constant
All About Circuits : Complex Circuits, Chapter 16 - RC and L/R Time Constants

Improve this answer
$\endgroup$
4
  • $\begingroup$ So, R1 would equal 400 ohms, and multiplying that by the equivalent capacitance gives the correct answer! Why are you allowed to ignore the other branch, though? Do you only include the resistors in the branch with the capacitor? Because what if there were capacitors that couldn't be combined to one equivalent capacitor, and there were different resistors in each branch with a capacitor; would the time constant be the sum of R multiplied by C for each branch? Also, for the disconnected battery, I got the time constant to be 8 ms, which matches the correct answer. $\endgroup$
    – christina
    Commented Feb 26, 2017 at 21:57
  • $\begingroup$ The PD across the $R_1C$ branch is not affected by what is in the $R_2$ branch, so it can be ignored (or removed) without effect on the $R_1C$ branch. ... There are no general rules : you need to identify which resistors affect charging and which affect discharging. ... Yes : If there capacitors in parallel branches then there is a separate time constant for each branch to charge (because the branches are independent). When the battery is disconnected the capacitors will not discharge because there is no PD between connected plates. $\endgroup$
    – sammy gerbil
    Commented Feb 26, 2017 at 22:29
  • $\begingroup$ I think I understand now; the only resistors that affect the time constant for a given branch are those that affect the potential difference across it, so resistors in parallel branches can be ignored. Thanks so much for explaining! $\endgroup$
    – christina
    Commented Feb 26, 2017 at 23:08
  • $\begingroup$ I just realised that my answer (and my comment above) are incorrect, so I have revised my answer. I apologise for misleading you. $\endgroup$
    – sammy gerbil
    Commented Apr 5, 2017 at 23:59
0
$\begingroup$

What you did for equivalent capacitance is right. For equivalent resistance use thevenin's Rth calculation technique where you can consider the equivalent capacitance as your load. Short circuit the source which will eliminate the branch with 4 resistance and you will be left with just 2 resistor in series as equivalent resistance.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.