Using the spacetime diagram, calculate the speed $v$ of the $Σ'$ frame relative to the $Σ$ frame, in terms of $t_A, t_B, c, x_A, x_B$

Question

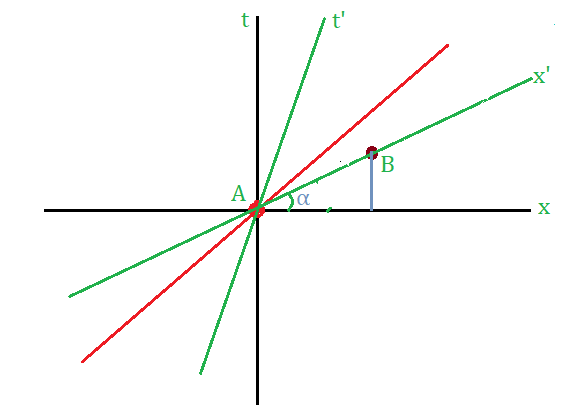
As measured in frame $Σ$, the coordinates of events $A$ and $B$ are respectively $(ct_A,x_A)$ and $(ct_B,x_B)$. The interval between the events are spacelike. There is then a frame $Σ'$ in which the two events are simultaneous.

Using the spacetime diagram, calculate the speed $v$ of the $Σ'$ frame relative to the $Σ$ frame, in terms of $t_A, t_B, c, x_A, x_B$.

Using a Lorentz transformation I found quite easily that: $\Delta t'=\gamma_v (\Delta t - \frac{v\Delta x}{c^2})$ which reduces to:

$v=(\frac{t_A-t_B}{x_A-x_B})c^2$ as $\Delta t'=0$.

I am struggling to derive this equation from the spacetime diagram. I have constructed a diagram similar to the one seen here {https://physics.stackexchange.com/q/367150} but I don't fully understand how to complete this problem from this.

I know I am just missing something simple but I could do with some help.

Answer 1

Sorry for the terrible graph but that's all I can do. For simplicity let me take A as the origin of the $\Sigma$ such that $A(ct_A, x_A)$ and $B(ct_B, x_B)$.

$A$ and $B$ are simultaneous means that they lie on the same plane w.r.t $\Sigma'$. Which mathematically also corresponds to $t'=0$. As you can see there is an angle between them so by using the below equation, we can obtain $$tan(\alpha) = v/c$$

$$\frac{c(t_B - t_A)}{x_B-x_A} = \frac{v}{c}$$