If a photon travels at $+c$ velocity and reflected to $-c$ it follows that there must be $0$ in between these velocities, just as if you threw a ball at a wall. But that presents a problem, as light can never be at rest since it is massless? Is the light absorbed and re-emitted at the surface? How exactly does this process work?
$\begingroup$
$\endgroup$
2
-
3$\begingroup$ Related: Is reflection instantaneous? $\endgroup$– VishnuCommented Apr 15, 2020 at 4:15
-
2$\begingroup$ Also related: How are classical optics phenomena explained in QED (Snell's law)?, How does reflection work?, Explain reflection laws at the atomic level. Remember that photons and their behavior cannot be properly understood using classical physics (quantum physics is required), but you can at least get closer to understanding this particular question by thinking of a photon as an extended wavepacket instead of trying to think of it as a tiny classical object. $\endgroup$– Chiral AnomalyCommented Apr 15, 2020 at 4:24
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
If photons always have an well-defined speed, and speed varies continuously, then it seems there logically must be a moment the speed is zero. But the real conclusion is that the notion of "speed" for a photon is breaking down.
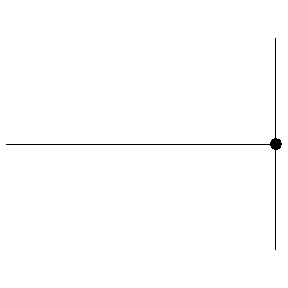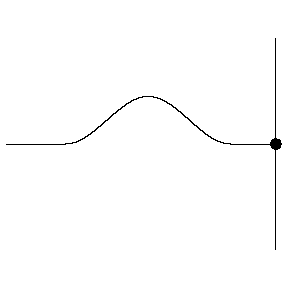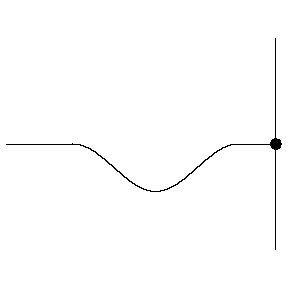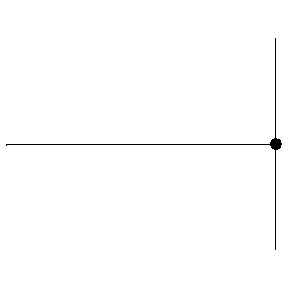
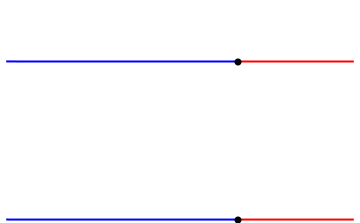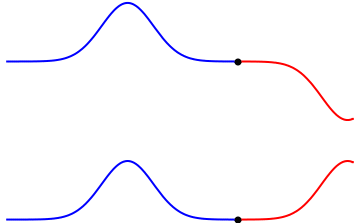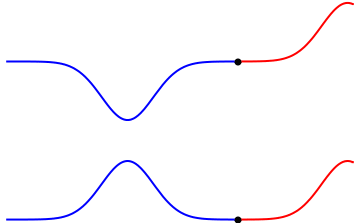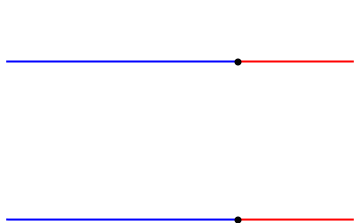
Consider a wave on a string reflecting off a wall (credit to Dr. Dan Russell, Penn State):
At some level, it's already ambiguous what the velocity the wave is before it reflected, because velocity is defined as the rate of change of position, and there isn't an unambiguous position; the wave is spread out. The only reason we can talk about a velocity at all is because the wave is keeping the same shape as it travels, so we can talk about the speed of that shape.
When the wave reaches the boundary, its shape rapidly changes. It doesn't get "absorbed and reemitted", there's no moment when the string stops moving. But for a while, it doesn't have a well-defined velocity in any direction. Something analogous happens for photons. When we say that photons always have speed $c$, we mean that plane waves of the electromagnetic field in free space travel at that speed (and hence so do wavepackets built around a given wavevector $\mathbf{k}$). This idea breaks down when you hit a boundary.
I suppose that if you really insisted on assigning a speed at that moment, you could use the fact that imposing a boundary condition is formally like taking the initial wave, and superposing it with a reflected, upside-down wave that travels "out of the wall". This is illustrated on top below:
Thus, the string wave is always a superposition of a part that travels with uniform velocity to the right, and to the left. So if you wanted to think about a photon this way, then during the reflection, it's in a quantum superposition of going in and out, with all elements of the superposition having speed $c$. But perhaps at this point it's better to step away from the idea of speed here: it's only making things more confusing, and an idea that does that isn't worth keeping!
