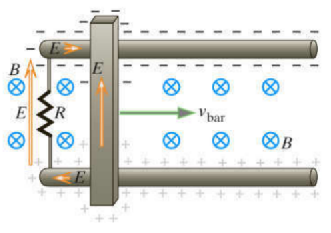
Conductors have always an intrinsic resistance, let's say $r$ the resistance of the bar and $r'(t)$ the resistances of the parallel rails (increasing with time as well as the length of the circuit), we consider $r<<R$ and $r'(t)<<R$. Let's call $I_{ideal}$ the current needed to equalize the magnetic field effect and $I_{real}$ the current $I$ that flows into $R$ due to the EMF $\varepsilon$. Surely it will be $I_{real}<I_{ideal}$ since the resistance in the circuit is slightly bigger than the ideal case, moreover the real current will depend on time. We can calculate $I_{real}$ by firstly obtaining the velocity of the bar $v_{bar}(t)$ in this way:
$$m\frac{dv_{bar}(t)}{dt}=\frac{v_{bar}(t)l^2B^2}{R+r+2r'(t)}$$
where $m$ is the mass of the bar and $l$ is the vertical length of the circuit. When we have the solution $v_{bar}(t)$ we can calculate $I_{real}(t)$ with this formula:
$$I_{real}(t)=\frac{v_{bar}(t)lB}{R+r+2r'(t)}$$
For simplicity we can neglect the term $2r'(t)$ since the dependence on time leads to a complicated calculus, this assumption gives rise to an exponential law:
$$I_{real}(t)=\frac{v_{0}lB}{R+r}\exp\left(-\frac{l^2B^2}{m(R+r)}t\right)$$
where $v_{0}$ is the initial velocity of the bar. Note that also velocity will decrease exponentially with time. The variations of current on time imply a variation of the flux of the magnetic field that generate EMF' different from the one associated with the field B. This new EMF' always opposes to the variation of current and it is given by
$$\varepsilon'(t)=-L(t)\frac{dI_{real}}{dt}$$
where $L(t)$ is the inductance of the circuit, it is a time dependent quantity since the circuit's area is changing on time. Now, the electric field can be calculated using the following formula:
$$\int \vec{E}(t)\cdot d\vec{l}=\varepsilon(t)+\varepsilon'(t)$$
Since the $\varepsilon'(t)$ has a negative sign, we have (at any time $t$):
$$eE(t) < ev_{bar}(t)B$$
Having an idea of the quantity "slightly less" implies facing a problem that can be solved computationally, I'll try to give an example: you can choose an interval of time $[0, t_{final}]$ and divide it into many steps $\Delta t$. Given a step $t_{i}$ you can calculate $L(t_{i})$ (since you know $v_{bar}(t_{i}$) you know how the area changes) and multiply it by the derivative $\frac{dI_{real}}{dt_{i}}$ to obtain $\varepsilon'(t_{i})$. Then you can easily obtain $\varepsilon(t_{i})$ and $E(t_{i})$ by simply
$$E(t_{i})=\frac{\varepsilon(t_{i})+\varepsilon'(t_{i})}{P(t_{i})}$$
where $P(t_{i})$ is the perimeter of the circuit. At this point, you can see how $eE(t_{i})$ differs from $ev_{bar}(t_{i})B$ and you can also see what happens when you increase the time by calculating $eE(t_{i}+\Delta t)$ and $ev_{bar}(t_{i}+\Delta t)B$.