About the explanation Purcell gives for why the electric field of a charge starting from rest looks the way it looks

Question

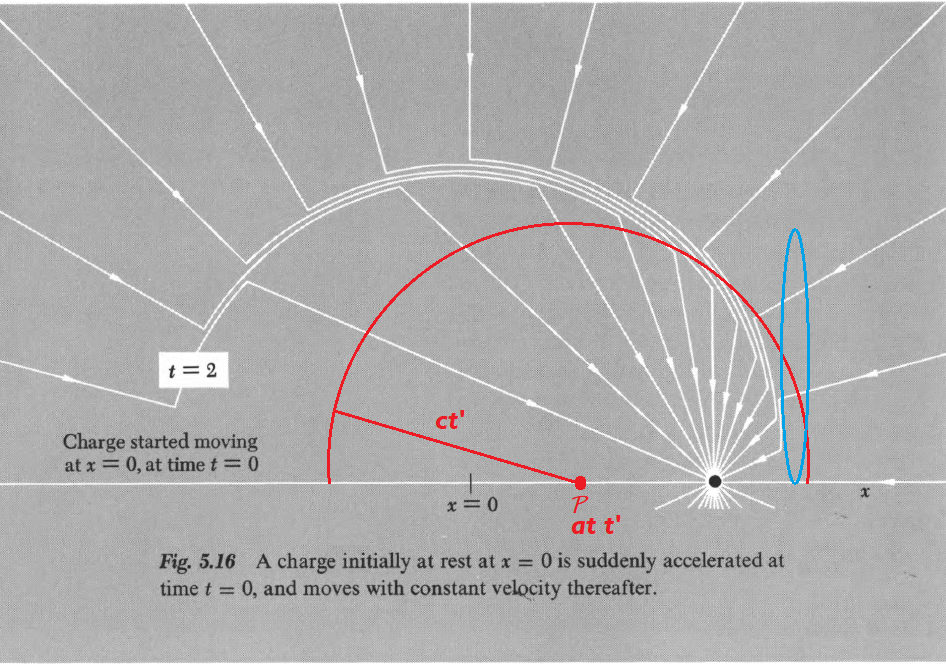
Purcell in his book Electricity and Magnetism, page $164$, explains why the electric field of an accelerated charge looks like the figure below.

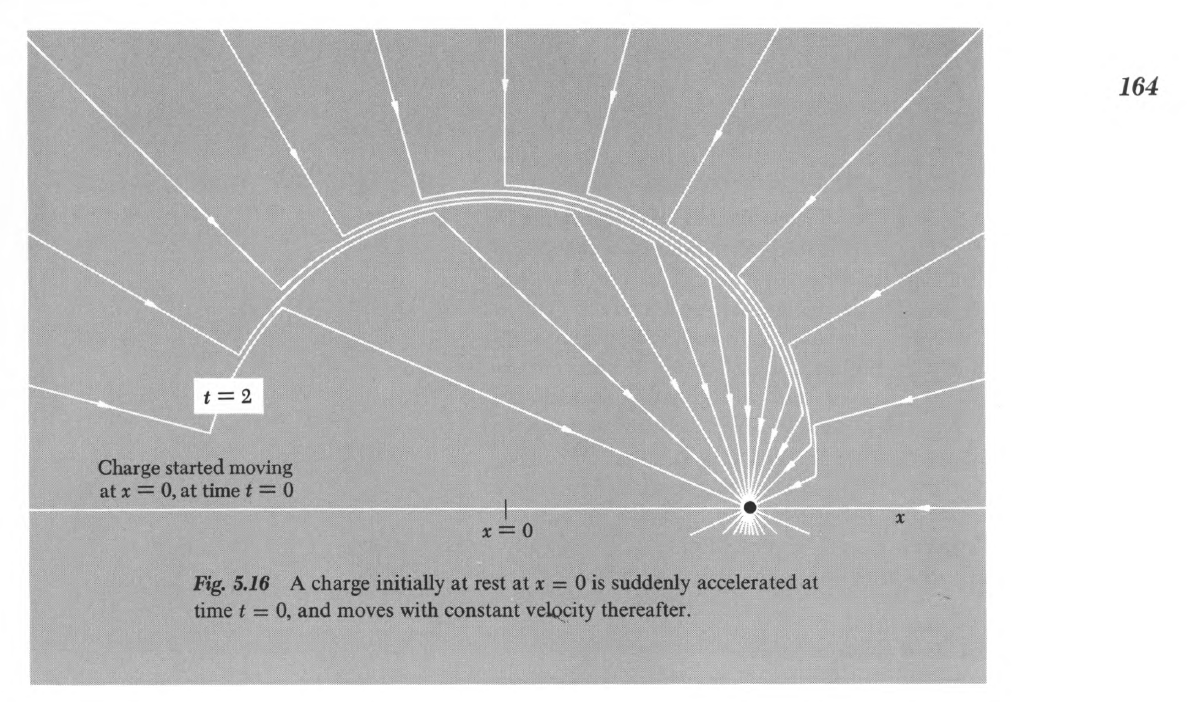
He says that because information can travel only as fast as the speed of light, if we examine the electric field at a given time $t$, at a distance $ct$ and beyond from where the particle was at rest, the field will remain unchanged, whereas at a distance less than $ct$ it will look like the field of uniformly moving charge.

What I don't understand in this explanation is why we take the place where the particle started moving as the only reference from which we can send the information that the particle started moving, and from there draw the corresponding electric field at a time $t$, why not use another intermediate point between where the particle was at rest and its position at a later time $t$.

In other words, why not use an intermediary point between the initial position and the position at time $t$ as the reference from which we can send the information that the particle has move and tell the field to adjust itself? Because the field in this case would be different from the one above: there would be a part of it that above was depicted as the field of a charge at rest whereas with the other point of reference this same part would be the field of a moving charge (in blue bellow).

Answer 1

You can do this, but you drew your circle incorrectly; if you keep track of the time carefully, it will be inside of Purcell's.

Considering the system at time $t$, his circle is centered at $x = 0$ and has radius $ct$, and if you center yours at a point $x'$, you must calculate its corresponding radius $ct'$ in terms of $x'$ and $t$. If the charge has velocity $v$, then you can verify that $$t = \frac{x'}{v} + t' \implies t' = t - \frac{x'}{v}$$ and supposing $v < c$, you can see that $ct' < ct - x'$, so your circle lies entirely inside of his. If $v = c$ then they are tangent at the point $x = ct$.