1
$\begingroup$

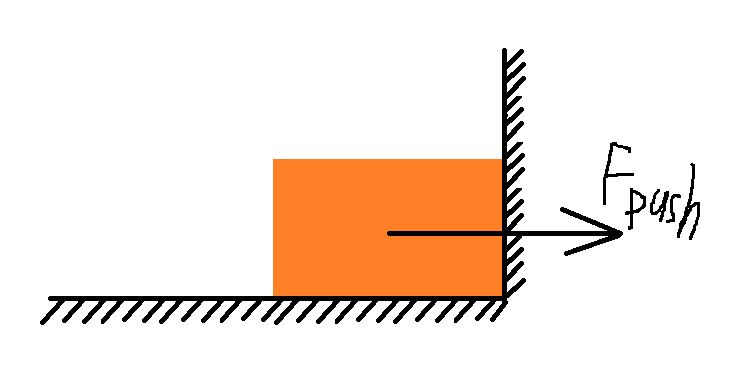
Here is the problem. A block (box) of a mass $M$ being pushed along a floor by a constant force of $F_\text{push}$. Both the static $\mu_s$ and kinetic ($\mu_k$) friction coefficients are given. I know that when the box is moving (sliding), it experiences a kinetic friction force opposing its motion. So if we write Newton's 2nd Law for the block, $ M a = F_\text{push} - F_\text{kinetic~friction}$. Now there is an unbreakable wall along its way; the box reaches (or hits) the wall and stops. But the pushing force is still there - it continues to push the box. What would be the force diagram for the box in this situation? What would be the equation of motion (i.e. Newton's 2nd Law) for the box in this situation?

enter image description here

I know that according to the 3rd Law of Newton, there will be an opposing "reaction" force (let's say $F_\text{react}$) from the wall proportional to the force the block is pressing against the wall with. But how must I count friction forces here? It should be kinetic or static friction? Since the block is not moving, I should account for static friction? But F_push was already greater than the maximum force of the static friction $F_{s,\text{max}}$. So kinetic friction must come into action? But the box is not moving...

Or should I take a different approach? Like, since the block is "stick" to the wall, it becomes a part of it. So pushing the block means pushing the wall? So there is no horizontal acting forces of friction?

I am confused here.

Improve this question
$\endgroup$
1
  • $\begingroup$ This is an indeterminate problem as the wall can generate forces that can be reacted by friction. Essentially the box can be wedged between the floor and the wall. $\endgroup$
    – John Alexiou
    Commented Oct 4, 2021 at 5:46

3 Answers 3

Reset to default
1
$\begingroup$

You only know that friction is static (no movement) and that the sum of Friction + Reactive force is equal to the pushing force, not the individual value of each. So you can treat them as a single force.

Improve this answer
$\endgroup$
0
$\begingroup$

static friction is can vary in the final situation shown by you, static friction is limiting friction it changes constantly with external force applied until it reaches max value

now in final situation the normal force from vertical wall $Nw$ would be opposite to $Fpush$(as you have shown) , so no net equation for equilibrium for forces in final situation----

$Fpush$ $=$ $Nw$ + $Fs$ -------1($Fs$ is force of friction and is static as the block is at rest but you cant say its $\mu sN${$N$ is normal force by floor} , because we do not if the net force is so high that it requires max static friction{its variable with force applied}) no Kinetic friction is considered , as there is no motion.

Note-- equation 1 is for horizontal equilibrium, same you can derive for vertical direction as well

Improve this answer
$\endgroup$
0
$\begingroup$

Draw the free body diagram of the block. You'll have vertical equilibrium by default, weight and the reaction force from the ground. Now consider the horizontal.

F=f+R,

where F is your pushing force. Since there's no relative motion, this friction must be static in nature.

Keep in mind though, we don't know the value of friction without also knowing F. If F is greater than fs(max), then the frictional force will be at its maximum value.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thanks for the comment. Then, what if F suddenly disappears here? For example, a person stops pushing the box (F = 0). Then it's: f = -R. Physically what does it mean? When two objects are in touch and at rest (when there are no "visible" pushing actions), they still are exerting on each other equal forces? $\endgroup$
    – Lit_try
    Commented Apr 12, 2020 at 8:20
  • $\begingroup$ Oh, I got it. When F becomes 0, static friction f also disappears, right? $\endgroup$
    – Lit_try
    Commented Apr 12, 2020 at 8:28
  • $\begingroup$ Yes, the reaction force from the wall becomes zero $\endgroup$
    – wavion
    Commented Apr 12, 2020 at 9:30

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.