Anomaly inflow mechanism

Question

I know very simple example of anomaly inflow. See section 4.4 in David Tong: Lectures on Gauge Theory. As I read, such mechanism have some applications in condensed matter and in quantum field theory, but I haven't face with some concrete applications. What is this application?

Essence of example:

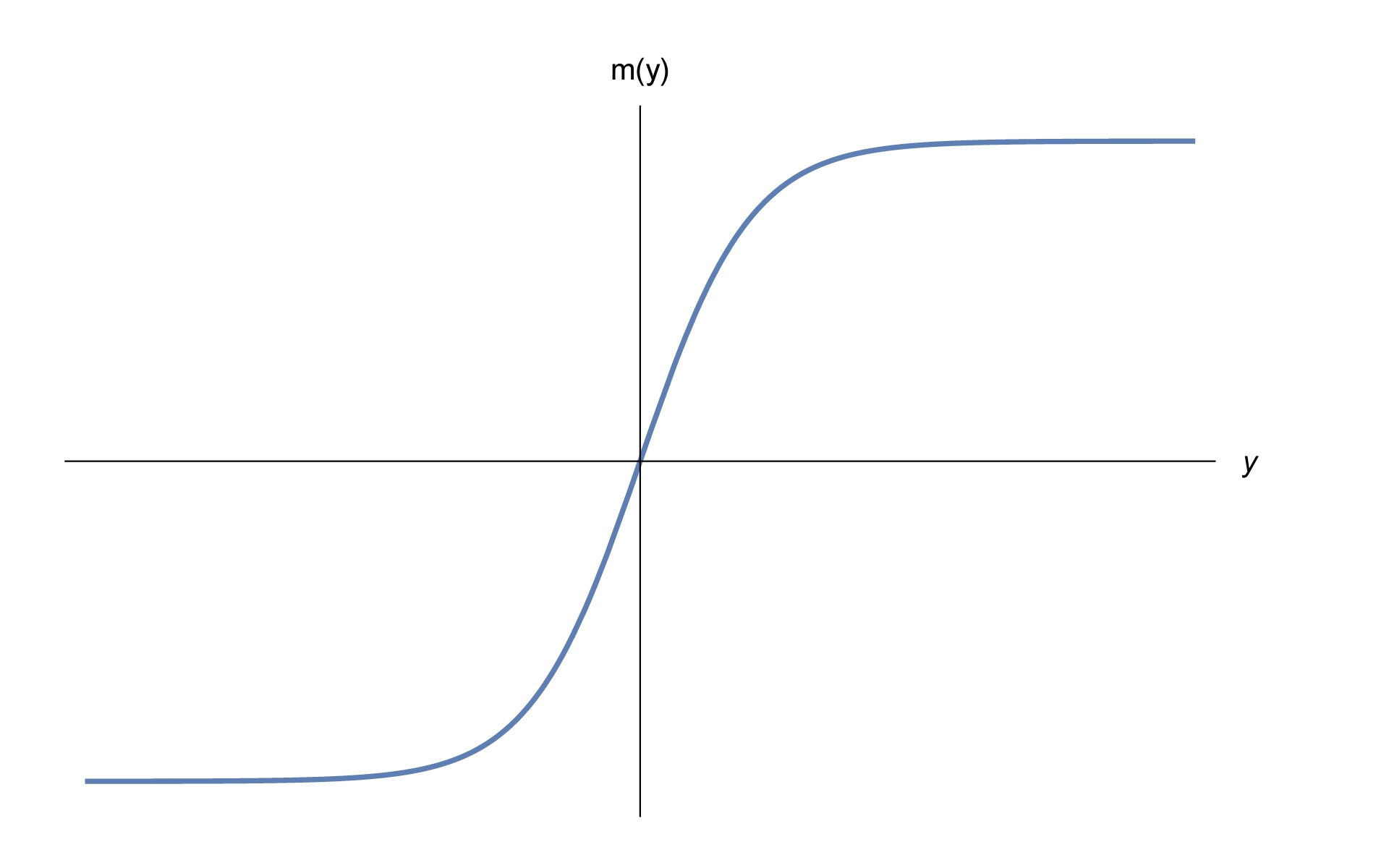
1. One consider 5d fermion with mass term, which depends on fifth coordinate (y): $$ i\not\partial \psi + i \gamma^5 \partial_y \psi - m(y) \psi = 0 $$

2. Exist special solution of this equation, after rewriting Dirac spinor $\psi$ as two Weyl spinors $\psi_+$ and $\psi_-$: $$ \psi_+ = \exp\left(- \int^y dy^\prime m(y^\prime) \right) \chi_+ (x) \;\;\;\;\;\;\;\;\;\; \psi_- = 0 $$ $$ \partial_0\chi_+ - \sigma^i \partial_i \chi_+ = 0 $$ The profile is supported only in the vicinity of the domain wall; it dies off exponentially $∼ e^{−M|y|}$ as $y → ±\infty$. Importantly, there is no corresponding solution for $\psi_−$, since the profile must be of the form $exp(+\int dy^\prime m(y^\prime))$which now diverges exponentially in both directions. So we obtain chiral fermion zero mode that lives on domain wall.

3. Chiral anomaly of this zero mode cancel with 5d Chern-Simons term. So theory $$ S = S^{5d}_{CS} + S^{4d}_{chiral fermion} $$ is gauge invariant. This known as anomaly inflow.

I wanna to understand more examples of such phenomena.

What kind of useful information can be extracted from such mechanism?

Could we consider more exotic gauge symmetries, what kind of generalisation allow such mechanism?

Also it is related to this question. As I understand, appearing of topological theory on domain walls are related to anomaly inflow in discrete symmetry. Some explanations of this phenomenona will be also appreciated!

Answer 1

The chiral edge states in the quantum hall effect are the best known example. See my ancient "Edge Waves in the Quantum Hall Effect," Annals Phys. 207 (1991) 38-52, for example. See also my answer to this question.

Answer 2

I apologise for giving not direct answer to the question asked, but what concerns applications, the idea proposed has an applications in Lattice QCD for such called domain-wall fermions, which is one possible way to implement chiral fermions for numerical simulation. As fas as I know, this approach has obtained some success in exploring chiral phenomena, but is rather expensive for numerical simulations, scaling even more badly with the size of lattice, than the more frequently used staggered fermions, for instance