0
$\begingroup$

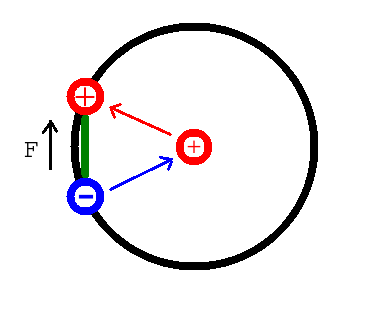
Suppose an electric dipole is confined to move tangentially on a circular track where a point charge has been placed at the center:

enter image description here

In the picture the green bar indicates that the two charges on the track form a dipole and that their separation is constrained to be constant.

The dipole will experience a tangential force at all times, implying indefinite acceleration around the track.

Where does the energy for this motion come from? What will cause the acceleration to cease?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

There is no tangential force. Both forces due to the central charge on the circumferential charges are exactly radial. So they have no effect on moving the charges on the ring.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Ok I see. It was not clear to me that the constraint forces should always oppose the net force (which is non-zero) exerted by the point charge. However, motion would require rotation but the net torque exerted by the point charge is zero. Also the potential energy is clearly constant as you move the dipole around the circle. $\endgroup$
    – creillyucla
    Commented Mar 19, 2020 at 17:23

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.