I would like some help in solving this problem.
Height is 15 cm , base is 88 cm
My attempt: $$(119.3 + m)(\sin(\theta)) = (88*9.8)$$ $$ 15^2 + 88^2 = 89.269^2 $$
$$ \sin^{-1} = 15/89.269 $$
$$ (119.3 + m)(9.6733) = (88*9.8) $$
$m = -30.14$ ....Am I missing something? I feel like it has something to do with the force of friction...
Justin,
So first off you know that if you have a base of 88 cm and a height of 15 cm on an inclined plane, we have $$\sin(\theta)=\frac{15}{x}$$ where $x= \sqrt{88^{2}+15^{2}}$. Solving, we obtain $\theta \approx 9.673$ degrees. We set up our equation, $$m_{bear}g=m_{box}g\sin(\theta)$$ Because we want the forces to cancel so the bear does not reach an unfortunate end. We then say $$88kg = (119.3+x)\sin(9.673)$$ and solve for x. I obtained $x \approx 404.3 kg$, so we would need to add an extra 400 kg to prevent the bear's fiery fate.
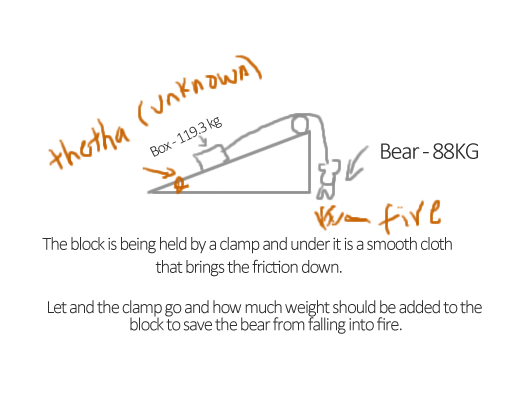
Draw a diagram with forces:
http://wstaw.org/m/2013/02/12/mixed2.png
Now we have $F_\text{box} = m_\text{box} g$ where $g$ is your gravitational acceleration with value $9.81\,\text{m/s^2}$.
The trick is now to separate the force of the into a parallel part $F_\parallel$ to the slope and another part $F_\perp$ perpendicular to the slope. The part $F_\perp$ will not contribute to the force on the rope, just the $F_\parallel$ part will do. Using trigonometry for the angle $\vartheta$, this force is: $$ F_{\text{box},\parallel} = F_\text{box} \sin(\vartheta) = m_\text{box} g \sin(\vartheta) $$
Now this force has to be exactly the same magnitude as the force on the bear, which is $F_\text{bear}$: $$ F_{\text{box},\parallel} = F_\text{bear} $$
We plug in what we have so far: $$ m_\text{box} g \sin(\vartheta) = m_\text{bear} g $$
We cancel the $g$: $$ m_\text{box} \sin(\vartheta) = m_\text{bear} $$
Since your $m_\text{bear}$ is given and $\vartheta$ has to be given and we want to know the mass that the box needs to have, we can solve this equation for $m_\text{box}$ like so: $$ m_\text{box} = \frac{m_\text{bear}}{\sin(\vartheta)} $$
I see that $\vartheta$ is given implicitly. You have $$\tan(\vartheta) = \frac{\text{height}}{\text{base}}$$
You can then solve this for $\vartheta$: $$\vartheta = \arctan\left(\frac{\text{height}}{\text{base}}\right)$$
Put this into the above equation: $$ m_\text{box} = \frac{m_\text{bear}}{\sin\left(\arctan\left(\frac{\text{height}}{\text{base}}\right)\right)} $$
Now you can put in all your numbers. If you subtract the mass you will get from the mass that you already have, you know how much more to add. If it is negative, you can even take mass away from the box and the bear will still be saved.
