Electric Flux by Gauss Law

Question

I know that if we want to find the magnitude of the total electric flux through the four faces of a tetrahedron consisting of $4$ equilateral triangles containing a charge $+q$ located at the center of the tetrahedron, we can use Gauss law since it is symmetrical and the electric field lines are perpendicular to the surface so the total electric flux in this case is $\dfrac q {\epsilon_0}$ . However, if we move the charge $+q$ very close to one of the four faces, the electric field becomes asymmetrical so I cannot apply Gauss law directly.

Is there a way to calculate the total electric flux through the four faces in this case or is there any other method to do it?

Answer 1

The total electric flux over a closed surface does not depend on the location of the source charges. It is true the flux through each surface of your tetrahedron will not be the same but the net flux through all faces will not change if you move the charge.

The way to see this is to draw a small sphere around your source charge, and suppose that small sphere is completely contained inside your tetrahedron. Any $\vec E$-field line that escapes through the small sphere must eventually escape somewhere on the surface of the tetrahedron, irrespective of where the source charge and small sphere enclosing it is located. An a sketch illustrating this argument using charge offset from the center of a sphere is shown below. As you can see there is more surface on left section intercepted by the cone but the field will be small on that part. Indeed the area of the surface intercepted grows like $r^2$ but the field decreases like $1/r^2$ so the increased area is exactly balanced by a decreased in the magnitude of the field. It is nevertheless clear that all the flux exiting through the smaller red sphere must also exit through the larger sphere.

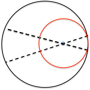
In your specific case, with the charge close to one surface, the flux through that surface would be larger than if the charge was at the center, but the flux through the other 3 surfaces would be smaller, but so in a way where the NET flux remains the same. Actually calculating the flux through each surface of the tetrahedron would be difficult because not all points of the surface are equidistant from the source and the angle betweeen $\vec E$ at the surface and $d\vec S$ at that point also changes from one point to the next.

Answer 2

However, if we move the charge +𝑞 very close to one of the four faces, the electric field becomes asymmetrical so I cannot apply Gauss law directly.

That's correct, if you only want the electric flux through each face individually and not the total flux over all the faces. To determine the flux on each face $A$, you would need to integrate the flux over that face, or

$$Φ_{A}=\int_A \overrightarrow E.d\overrightarrow A$$

Since the flux over each surface is non-uniform, the calculation would obviously be difficult.

Hope this helps.

Answer 3

The total flux does not change. However if you want to find flux through each of the face separately, we can still use gauss law by assuming the the charge to be at the centre of a bigger symmetric object.