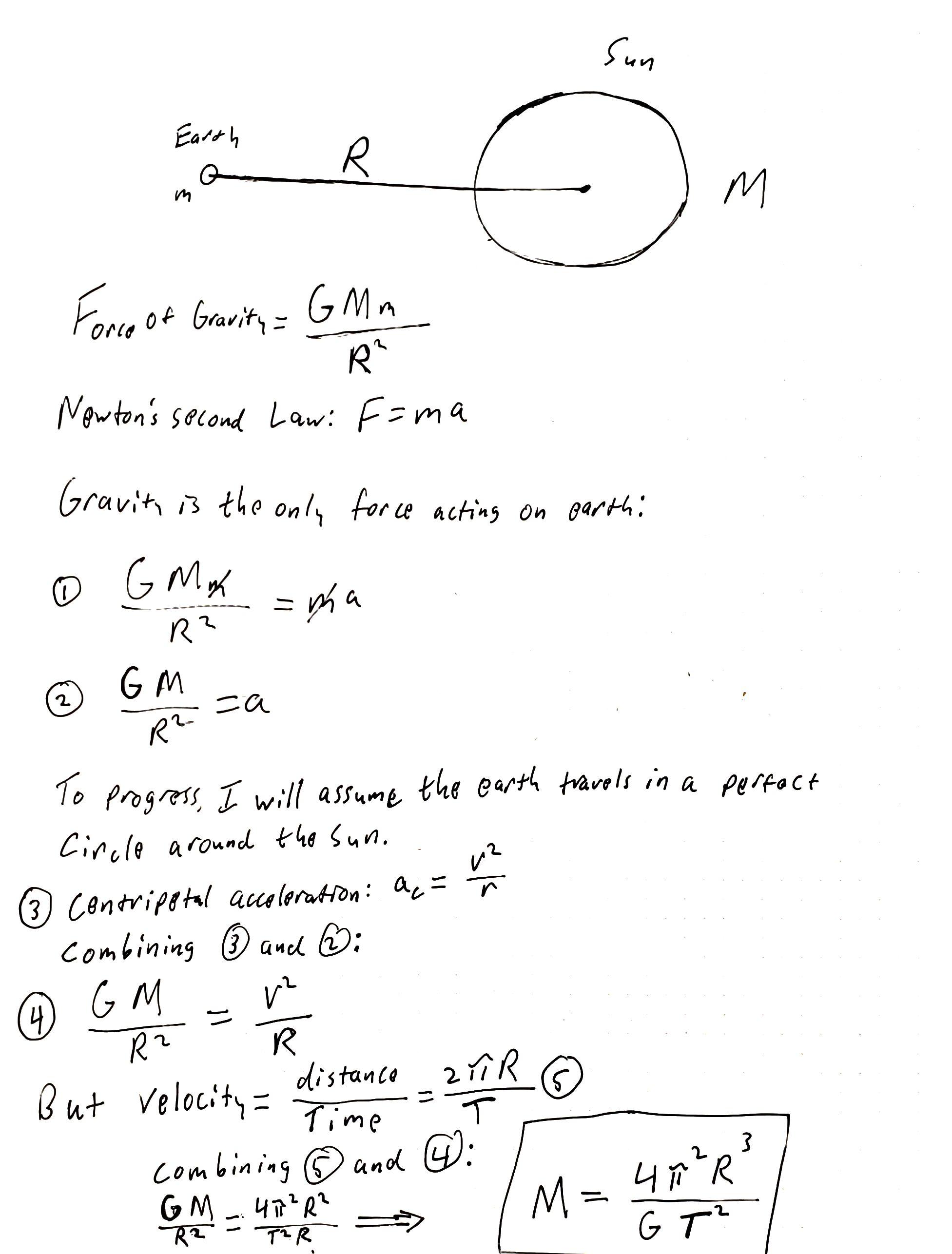
By measuring the Earth’s distance from the Sun, and knowing its orbital period, Newton’s Law of Universal Gravitation and Newton’s 2nd Law can I calculate the mass of the sun?
$\begingroup$
$\endgroup$
4
-
$\begingroup$ You need the mass of the earth $\endgroup$– Superfast JellyfishCommented Jan 30, 2020 at 6:16
-
$\begingroup$ You actually don't need the mass of the earth. Here's a hint: set the force due to gravity between the earth and the sun, equal to the centripetal force that the sun puts on the earth. A bit of algebra will "drop" the mass of the earth, and a bit more algebra will allow you to separate the mass of the sun as the only unknown. $\endgroup$– David WhiteCommented Jan 30, 2020 at 6:20
-
$\begingroup$ The answer is therefore :yes. $\endgroup$– my2ctsCommented Jan 30, 2020 at 6:56
-
$\begingroup$ You mean Kepler's third law? $\endgroup$– ProfRobCommented Jan 30, 2020 at 6:56
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
2
Earth's distance from sun: $ R $
Orbital period: $T$
Sun's Mass: $M$
Earth's mass: $m$
Newton's Law of Universal Gravitation: $ F = \frac{GM m}{r^2} $
Newton’s 2nd Law: $ F = ma$
Okay, let's see what we can do.
Actual mass of sun: $ 1.989 * 10^{30} kg $
Error = $ 3.37 \% $
So this approximation isn't bad! The earth's orbit is not exactly a circle, though. It is an ellipse. Newton discovered that the earth and sun both orbit around a common center of mass, dispelling the idea that one orbits around the other.
