In cylindrical coordinates I had derived the acceleration as :
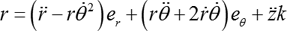
for the first component of acceleration, what is the difference between radial and centripetal ?
In cylindrical coordinates I had derived the acceleration as :
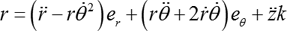
for the first component of acceleration, what is the difference between radial and centripetal ?
The radial acceleration, $\ddot r$, is responsible to change the rate of change of the radius vector, $\dot r$, which in turn is responsible to change the magnitude $r$ of the radius vector, its length.
The centripetal acceleration $-r \dot \theta^{2}$ is what makes the particle describe some curvilinear path. The "minus" signal in front of the centripetal acceleration indicates it's inward.
If we consider only the radial component in the case when $\dot{\theta} = 0$, then it becomes clear that $\ddot{r}$ is a radial acceleration, i.e. since the angular component of velocity is $0$, the particle is only moving along a straight line through the origin, and $\ddot{r}$ is the acceleration along this line. $$\\$$ Now if we consider when $\ddot{r} = 0$, with $\dot{r} = 0$ also (incidentally, this is just the condition for circular motion around the origin), then $-r{\dot{\theta}}^2$ must be the source of what is making us turn.
The only thing I can add to the existing answers that may be helpful is a visual representation, which can be found on Saif Rayyan's MIT course page here and is reproduced below