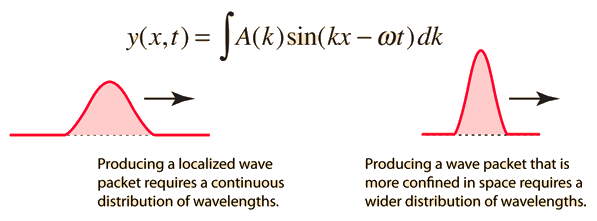3
$\begingroup$

Can we determine the probability of finding electron in a region of space if there is no boundary condition?

For example, if the wall of a one-dimensional potential well is infinitely far away then is there a way that we can find the probability of finding the electron in a given region of space?

Improve this question
$\endgroup$

3 Answers 3

Reset to default
6
$\begingroup$

I would rephrase the correct answer by Thatpotatoisaspy as:

when there are no boundary conditions there are the plane wave solutions of the quantum mechanical equations. Plane waves go from -infinity to + infinity so they are not good for modeling the probability of finding the electrons in our experiment. But, there exist wave packets, which can model the probabily:

wavepackets

The calculation tools for computing the probabilities of interaction of elementary particles do not need such modeling as they use the Feynman diagrams of interactions, which have specific rules that write down the integrals from the diagrams, without a need for modeling individual incoming particles, and they are quite good in their predictions for the probability of interactions.

Improve this answer
$\endgroup$
7
  • 2
    $\begingroup$ My answer was very very brief so thank you for expanding on this. $\endgroup$
    – Thatpotatoisaspy
    Commented Jan 6, 2020 at 11:10
  • $\begingroup$ The boundary conditions still exist, they are just different: boundedness as $|x|\to\infty$ (this will also let you find plane wave solutions). $\endgroup$
    – Ruslan
    Commented Jan 6, 2020 at 15:00
  • $\begingroup$ @Ruslan yes, that is why I went directly to the plane wave solutions $\endgroup$
    – anna v
    Commented Jan 6, 2020 at 15:12
  • $\begingroup$ My point is that without boundary conditions you have no restrictions on the wavefunction: e.g. it could be $\exp(x)$, which is obviously unphysical. $\endgroup$
    – Ruslan
    Commented Jan 6, 2020 at 15:15
  • 1
    $\begingroup$ @annav only for physically allowable states, but not for eigenstates in general (otherwise you lose all the continuous spectrum). $\endgroup$
    – Ruslan
    Commented Jan 6, 2020 at 15:53
2
$\begingroup$

I think it's important to point out that if a problem admits bound states, then there is always a boundary condition at infinity, which comes from demanding that the wave function go to zero as $x \to \pm \infty$.

It's interesting to note that for a particle in a box, the quantisation condition that leads to discrete solutions comes from demanding that the wave function vanish at (say) $x=0$ and $x=L$. Similarly, in problems like the harmonic oscillator or the hydrogen atom, the quantisation conditions arise by imposing the condition that the wave functions vanish at $\pm \infty$. (This is worked out in Griffiths' text.)

Now, the case that you mention in your question is essentially that of a free particle (a particle in a box with the walls infinitely far away), and as mentioned in the answer by Thatpotatoisaspy, you can construct a wave packet to define the particle as being ''localised'' somewhere, and thus you can speak of a particle as having some probability of being found somewhere in space.

Improve this answer
$\endgroup$
1
$\begingroup$

Yes. A good example is a wave packet for the free particle. The ordinary free particle solution is not normalisable, but you can construct another solution which is, the wave packet.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.