Path Coordinates: direction problem (doubt) in derivative of tangential vector

Question

Why is the direction of derivative of tangential vector perpendicular to the direction of the tangential vector?

Answer 1

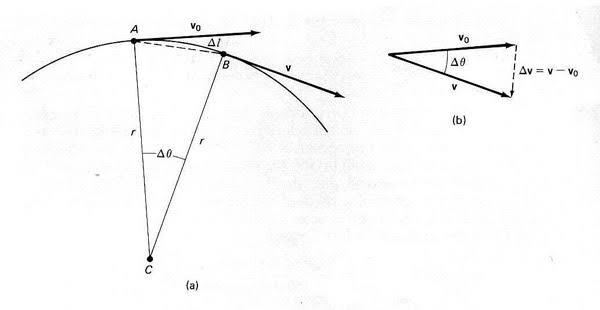
^{Image credits :- http://milesmathis.com/avr.html}

---

Here in the above diagram, you can see that the difference of a tangential velocity vector gives you the average acceleration vector. Now when $\Delta \theta \rightarrow 0$, $\Delta t \rightarrow 0$ and $\Delta v\rightarrow 0$ simultaneously, so the average acceleration becomes instantaneous acceleration which is,

$$a_{inst}=\lim _{\Delta \theta \to 0} \frac{\Delta v}{\Delta t}$$

And since $\Delta v$ is perpendicular to $v$ in the limiting case, so is $a_{inst}$. Thus the time derivative of velocity is perpendicular to the velocity.

I used to concepts of velocity and acceleration for better visualisation. These arguments can easily be transferred to your specific question. Your tangential vector is analogous to velocity and its derivative is analogous to acceleration.

Now you might wonder that is it only true in the cases where the path has a constant radius of curvature ($r$)? (Because that's what is shown in the above diagram) The answer is no. This result is very general. You can choose any arbitrary path and zoom in really close at a small segment. Then we can approximate that segment as an arc of a circle of radius $R$, where $R$ is the radius of curvature of the path at that point. This way, the result for an arc can be extended to any differentiable curve.

---

Caution :- This is only applicable where the length of the tangential vector (or the magnitude of the velocity) remains a constant.