Blinking flashlight infalling into the black hole, as frequency → ∞

Question

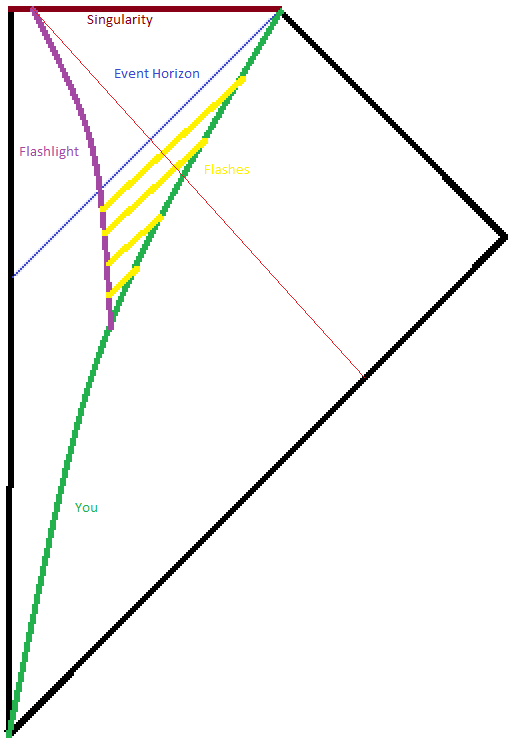
This is my third question from a series were I progressively refine my thought experiment. The others are:

• Can something (again) ever fall through the event horizon?
• Time of flashlight crossing the event horizon as seen from an external observer

Assume I am stationary over a Schwarzschild black hole of mass $M$. Some force is keeping me at a distance $d$ over the event horizon (or the black hole center, whatever is easier to answer). At time $0$ I drop a flashlight blinking at frequency $f$ into the black hole. At what time $T_{M, d}(f)$, in my own frame of reference, will I see the last blink of the flashlight?

Some assumptions (non-comprehensive list):

• Assume only general relativity (the world is not quantized, no Hawking radiation);
• The flashlight is not destroyed/spaghettified by the tidal forces;
• I can always detect the flashlight as long as it has not crossed the event horizon;
• I can set $f$ to any arbitrarily big frequency.

It would be nice if the answer was the actual function/algorithm describing $T_{M, d}(f)$, so I could graph for increasing values of $f$, but the real information I want to know is:

Is it true that $$\lim_{f \rightarrow \infty} T_{M, d}(f) = \infty \;\;\;\; \text{?}$$

Answer 1

The coordinate acceleration by proper time for a radially infalling observer is simply the Newtonian expression

$$ \ddot{r} = \frac{{\rm d}^2 r}{{\rm d} \tau^2} = -\frac{G M}{r^2}$$

which integrates to

$$ \dot{r} = \frac{{\rm d} r}{{\rm d} \tau} = -\sqrt{\frac{2 G M (r_1-r)}{r_1 \ r}} $$

so if you start at rest at $r_1$ and freely fall to $r_2$ the elapsed proper time is

$$ \tau = \int_{r_1}^{r_2} \frac{1}{\sqrt{2 G M \left(\frac{1}{r}-\frac{1}{r_2}\right)}} \, \text{d}r$$

The time dilation relative to the coordinate bookkeeper far away from the black hole is

$$ \dot{t} = \frac{{\rm d} t}{{\rm d} \tau} =\sqrt{\frac{c^2 \ r-c^2 \ r_s +r \ \dot{r}^2}{c \ (r- r_s ) \ (1-r_s/r)}} $$

For example let's plug in some numbers; if you fall from the photon sphere at $r_1=1.5 \ r_s$ you reach the horizon at $r_2=r_s=2G M/c^2$ when your proper time is $\tau=3.993468$ so if you set the intervall of your flashlight to give $100$ pulses per $1GM/c^3$ proper time, the last received pulse is that given at $\tau=3.99 \ G M/c^3$ when the flash light is at $r=2.001999 \ G M/c^2$, which is at coordinate time $t=18.43309 \ G M/c^3$.

If you want to know when this last pulse of light is observed you have to add the light travel time; the shapirodelayed coordinate velocity of a radially outgoing light ray is

$$ r' = \frac{{\rm d} r}{{\rm d} t} = c-\frac{2 G M}{r c} $$

so the light travel coordinate time from where that signal was emmitted to the observer at $r_1=3 G M/c^2$ is $t_{\rm \ light} = 13.42798 \ G M/c^3$, so the last signal which is emmitted outside of the horizon will be received at $t_{\rm obs}=t+t_{\rm \ light}=31.86107 \ G M/c^3$, or in terms of the proper time of the stationary shell observer at $r=r_1$ that $399 \rm th$ and last signal would be received at ${_{T}}_{\rm obs}=t_{\rm obs} \sqrt{1-r_s/r_1}=18.395 \ G M/c^3$.

If you set the frequency of your flashlight to the limit of infinitely many pulses per unit proper time, the last received signal will also be seen only after an infinite coordinate- or shell-time.

Answer 2

There is nothing like some badly drawn paint art to explain relativity. So here we go.

Below we see a spacetime diagram of the experiment. This type of diagram (a Penrose diagram) is drawn is such a way that light always travels on diagonal lines.

Between the time that you (the green line) drop the flash light (the purple line) and that the flashlight crosses the event horizon (blue diagonal) a finite amount of proper time will pass for the flashlight. Let call that $T$.

If the flashlight flashes with a frequency $f$ the flashlight will emit $n =\lfloor T/f\rfloor$ flashes before crossing the event horizon.

Each of these flashes (and only these flashes) will reach you. However, for each subsequent flash it will take longer to reach you.

So yes, in the limit that $f\to 0$ the number of seen flashes $n$ will go to infinity. From the diagram it is also immediately clear that this answer does not depend on whether the flashlight is freely falling or rocket power (this will change $T$ but it will always be finite if the flashlight crosses the event horizon), or whether you keep position, orbit the black hole, or return to the distant universe.

EDIT: In the limit $f\to 0$ it also follows that the last flash is emitted arbitrarily close to the event horizon. Since this flash follows the same diagonal slope as the event horizon, this means that this flash intersect the green line (you) arbitrarily close to the top right corner of the diagram, which represent $t\to\infty$ for your clock. So the arrival time of the last flash on your clock will also tend to infinity. Note that this is not because it took such a "long time" for the last flash to be emitted, but because it took a "long time" for the signal to reach you.

EDIT2: I've add another diagonal red line. Once you (the green line) has crossed this redline, there is no way for you the ever send a signal to the flashlight again. In particular, you could no longer dive into the black hole after your flashlight and reunite with it.

^*The astute reader will wonder what "long time" means, and rightly so. For the purpose of this statement, lets just think about it as far away in the diagram.