Why is it that a force that acts on two masses can cause both masses to accelerate at the same time?

Question

Suppose box A is connected to a larger box B, and both are on top of a frictionless surface. My hand applies a force to box A in the direction of box B ($F_{ha}$), causing boxes A and B to move. So box B moves. By Newton's third law, the force exerted by box B on to box A ($F_{ba}$) = -the force exerted by box A on to box B ($F_{ab}$). Now, the only force (that we care about for now) external to the system is $F_{ha}$, then $F_{ha} = F_{ab} = -F_{ba}$. So mathematically, Box A should not move.

Technically, though, we can apply a force such that Box A and Box B both move. So the net force acting on box A > $F_{ba}$. How is this possible?

Answer 1

The issue with your reasoning lies in the assumption (or, mistaken conclusion) that $F_{ha}=F_{ab}$. I can imagine why one might be led to think this is true. One might think that the force that the hand applies to box $A$ is somehow transferred to box $B$ due to its contact with box $A$ on which we are applying the force $F_{ha}$. And since this force $F_{ha}$ is simply transferred to box $B$, the force on box $B$ via box $A$ must be the same as $F_{ha}$. However, this is not true for the following reason.

It is true that the force acting on $B$ due to $A$ is essentially due to the force being applied on $A$ by our hand. So, in that sense, the box $A$ is transferring the force applied on it onto box $B$. But, the point is that box $A$ doesn't transfer all of the force onto box $B$. It transfers some of it and the difference is precisely equal to the force that would be needed to produce that acceleration with which the box $A$ would end up moving. Let's make this story more precise via figuring out an expression for the force applied on $B$ by $A$ in terms of the force applied by the hand on $A$.

Let's say the system $A+B$ accelerates with an acceleration $a$. Then, since the only external force on the system $A+B$ is $F_{ha}$, we can easily deduce that $$a=\frac{F_{ha}}{m_a+m_b}$$ Now, the only force acting on $B$ is $F_{ab}$. Thus, this acceleration must be produced by $F_ab$ in $B$. Thus, we obtain $$F_{ab}=m_ba=\frac{F_{ha}m_b}{m_a+m_b}=\frac{F_{ha}}{1+\frac{m_a}{m_b}}$$Or, in other words, the fraction of the force that is transferred by the box $A$ onto box $B$ is given by $$\frac{F_{ab}}{F_{ha}}=\frac{m_b}{m_a+m_b}=\frac{1}{1+\frac{m_a}{m_b}}$$We can verify that the net force acting on $A$ would thus be equal to $F_{ha}-F_{ab}=\frac{F_{ha}m_a}{m_a+m_b}=am_a$. This means that the net force on box $A$ is exactly what is needed for it to accelerate with the acceleration $a=\frac{F_{ha}}{m_a+m_b}$. In some rough sense, box $A$ "absorbs" this much force and transfers the rest to box $B$.

Answer 2

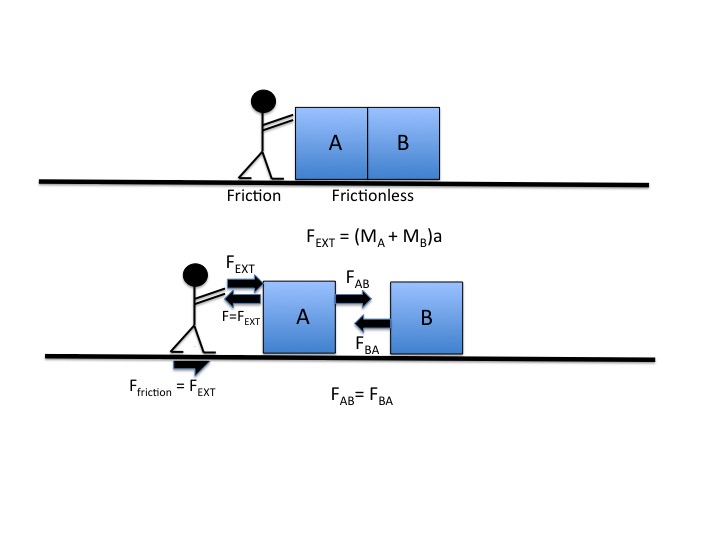
Consider box A and B together as comprising a system. See the diagrams below. You apply a force to box A in contact with box B, call it $F_{ext}$. At the interface between the boxes Box A applies a force to Box B, call it $F_{AB}$ and per Newton's third law box B applies an equal and opposite force on Box A, call it $F_{BA}$. The equal and opposite action/reaction forces $F_{AB}$ and $F_{BA}$ are internal to the system and cancel each other

But the force you apply, $F_{ext}$ is external to the system of the two boxes. Since the boxes are on a frictionless surface there is no external friction force acting on the boxes opposing your applied force.

But you also need to consider that when you push on the two boxes they together push back on you with an equal and opposite force again per Newton's third law. In order for that reaction not to cancel your externally applied force, there needs to be another external force, the static friction force between you and the surface, to oppose the reaction force of the two boxes. Imagine trying to move the boxes if you were standing on ice. As long as your external force does not exceed the maximum static friction force between your feet and the surface, you will not slip.

If you look at the bottom diagram showing all the forces you can see that they all cancel each other, except for your externally applied force to the two boxes, and thus

$$F_{ext}= (M_{A}+ M_{B})a$$

As a matter of fact, it is the static friction force between your feet and the surface that is responsible for you being able to accelerate the boxes.

Hope this helps.

Answer 3

Force balance on box A: $$m_Aa=F-F_{ba}$$ Force balance on box B: $$m_Ba=F_{ab}$$ Adding the force balances together:$$(m_A+m_B)a=F-F_{ba}+F_{ab}=F$$

Answer 4

There is no reason why $F_{ha} = F_{ab}$. If we write down the forces on the system:

$$ F_{ha} + F_{ab} + F_{ba} = (M_A + M_B)a $$

And as $F_{ba} = - F_{ab}$ then $F_{ha} = (M_A + M_B)a$

So, internal forces cancell each other and won't produce any acceleration.

If you think about it, you don't need two separate boxes. When you push any object, everý slice of it is in contact with the next one. If reaction forces cancelled external forces, nothing would ever move.