Understanding the statistical mechanics of Recombination Epoch ("Cosmology" Weinberg)

Question

I also posted this in Astronomy.stackexchange, but realize it is primarily the physics I am trying to understand, not astronomy.

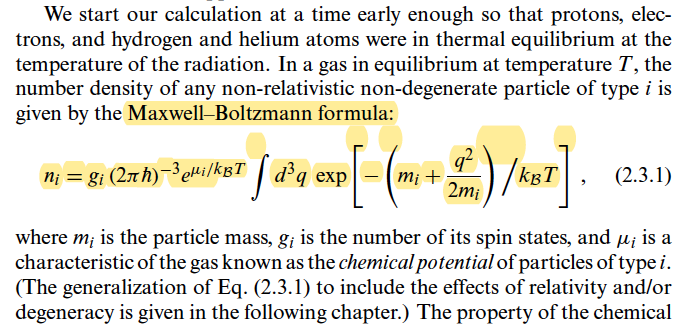
In Steven Weinberg's 'Cosmology' Chapter 2.3 (pg 113), he begins with a number density for some particle via the Maxwell-Boltzmann Formula:

While I have some familiarity with statistical mechanics, I can't figure out how this equation was derived. The spin states and fugacity are obvious enough for any quantum microcanonical ensemble. The $(2\pi\hbar)^{-3}$ and the integral however I am not sure where they come from. I am used to working with canonical ensembles in terms of partition functions and that doesn't seem to be the case here unless I am missing something.

Answer 1

Most of formula (2.3.1) is a standard grand canonical ensemble for a relativistic Maxwell-Boltzmann (MB) distribution. Here the energy $E_i=\sqrt{(pc)^2+(m_ic^2)}~=~m_ic^2+ \frac{p^2}{2m_i}+{\cal O}(c^{-2})$; speed of light $c=1$; and momentum $p\equiv q$. The normalization factor $(2\pi\hbar)^{-3}$ implements the semiclassical quantization rule that there is 1 quantum state per unit volume $(2\pi\hbar)^3$ of a 6D phase space.