Effect of magnetic field on time period of a spring pendulum

Question

Let us consider that we have a spring pendulum, and a magnet nearby. Assuming that the spring is being attracted toward the magnet, does the period decrease?

The formula of time period is = 2𝜋√m/k Will the magnetic have the same effect as increasing g?

Answer 1

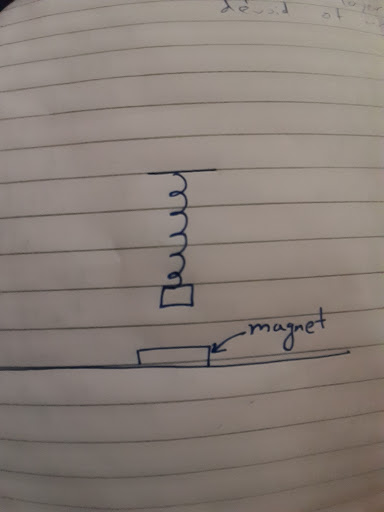
Non-technical version: the magnet is pulling the mass down, and the magnitude of this force increases as the spring extends. The spring is pulling the mass up, and the magnitude of this force also increases as the spring extends. The effect of the magnet is therefore to decrease the effective stiffness of the spring, since the magnet is working against the spring. The net force on the mass from the spring & magnet combined increases less quickly than the force from the spring alone would; in other words, adding the magnet is basically equivalent to replacing the original spring with a less stiff one. Since the period of a mass on a spring decreases as the spring's stiffness decreases, the period of the oscillations with the magnet present will be lower than without the magnet present.

---

Technical version: Let $z$ be the vertical coordinate of the mass, with positive $z$ measured downwards from the unstretched location of the spring. The mass will experience three forces:

• Gravity, with $F_g = +mg$;
• The spring force, with $F_s = - k z$; and
• A force $F_m(z)$ from the magnet. This force will be (presumably) downwards for all $z$ values, and will generally be increasing as $z$ increases: $dF_m/dz > 0$.

Let $z_0$ be the coordinate at which the system is in equilibrium. At this point, the net forces on the mass must cancel: $$ F_g + F_s + F_m = mg - k z_0 + F_m(z_0)= 0. $$ Let's now imagine displacing the mass a small distance $\epsilon$ from equilibrium; in other words, $z = z_0 + \epsilon$. Since $\ddot{z} = \ddot{\epsilon}$, Newton's second law becomes: \begin{align} m \ddot{\epsilon} &= F_g + F_s + F_m \\ &= mg - k(z_0 + \epsilon) + F_m(z_0 + \epsilon) \\ &\approx mg - k z_0 - k \epsilon + F_m(z_0) + F_m'(z_0) \epsilon \end{align} where we have expanded $F_m(z_0 + \epsilon)$ in a Taylor series in the last step. From the equilibrium condition, this then reduces to $$ m \ddot{\epsilon} = -(k - F_m'(z_0)) \epsilon $$ and so the frequency of the oscillations is $$ \omega = \sqrt{ \frac{k - F_m'(z_0)}{m}} < \sqrt{\frac{k}{m}}. $$ Since the angular frequency of the mass in the absence of the magnet is $\sqrt{k/m}$, we conclude that the period of the oscillations increases in the presence of the magnet, and so the period decreases.

(This all assumes that $F_m'(z_0) < k$. If this was not the case, then the equilibrium position would be unstable.)