There is no asymmetry!
Or, the asymmetry is a result of using the magnetic vector $\mathbf b$, instead of the magnetic bivector $B$. The former is a directed line segment; the latter is a directed plane segment; they're perpendicular to each other.
$$\mathbf b=b_x\mathbf e_x+b_y\mathbf e_y+b_z\mathbf e_z$$
$$B=-b_x\mathbf e_y\mathbf e_z-b_y\mathbf e_z\mathbf e_x-b_z\mathbf e_x\mathbf e_y$$
Here $\mathbf e_x$ is the unit vector along the $x$-axis, and similarly for $y$ and $z$. And $\mathbf e_x\mathbf e_y$ is the unit bivector in the $x,y$-plane. (Actually there are two; the other is $\mathbf e_y\mathbf e_x=-\mathbf e_x\mathbf e_y$.) The vectors are being multiplied with the geometric product, which is associative, and satisfies $\mathbf v\mathbf v=\lVert\mathbf v\rVert^2$ for any vector $\mathbf v$. Multiplying a vector with a bivector produces two parts: another vector, called $\mathbf v\cdot B$; and a trivector, called $\mathbf v\wedge B$:
$$\mathbf vB=(v_x\mathbf e_x+v_y\mathbf e_y+v_z\mathbf e_z)(-b_x\mathbf e_y\mathbf e_z-b_y\mathbf e_z\mathbf e_x-b_z\mathbf e_x\mathbf e_y)$$
$$=(v_yb_z-v_zb_y)\mathbf e_x+(v_zb_x-v_xb_z)\mathbf e_y+(v_xb_y-v_yb_x)\mathbf e_z\\+(-v_xb_x-v_yb_y-v_zb_z)\mathbf e_x\mathbf e_y\mathbf e_z$$
$$\mathbf v\cdot B=(v_yb_z-v_zb_y)\mathbf e_x+(v_zb_x-v_xb_z)\mathbf e_y+(v_xb_y-v_yb_x)\mathbf e_z$$
$$=\mathbf v\times_3\mathbf b$$
$$\mathbf v\wedge B=(-v_xb_x-v_yb_y-v_zb_z)\mathbf e_x\mathbf e_y\mathbf e_z$$
$$=(-\mathbf v\cdot\mathbf b)I$$
Here $I=\mathbf e_x\mathbf e_y\mathbf e_z$ is the right-handed unit trivector (the left-handed one is $-I$), and $\times_3$ is the vector cross product which only works in 3D. (In contrast, geometric algebra works in any number of dimensions.) We also have $IB=\mathbf b$, an instance of Hodge duality.
So the Lorentz force $q\,\mathbf v\times_3\mathbf b$ can be replaced with $q\,\mathbf v\cdot B$. Geometrically, this is the projection of $\mathbf v$ onto the $B$ plane, rotated $90^\circ$ in that plane, and scaled by $q\lVert B\rVert$.
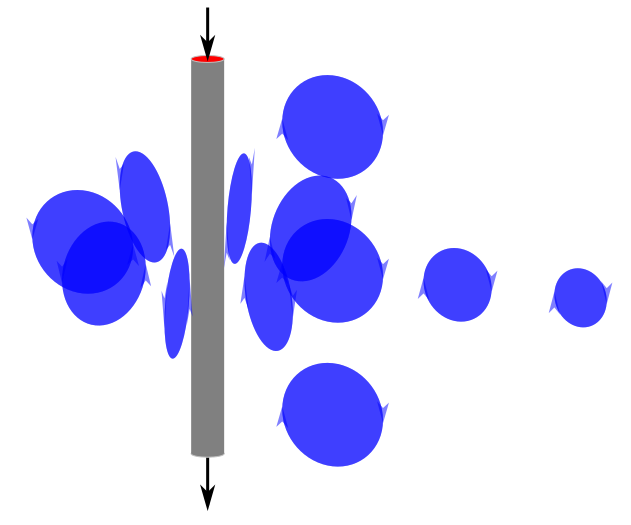
Here's a 3D illustration of the magnetic bivector field around a wire with current flowing downward. The magnitude of $B$ is represented by the area of the disk, which decreases with distance from the wire. The plane of $B$ always contains the wire. There is rotational symmetry, and mirror symmetry.
More information: http://www.av8n.com/physics/clifford-intro.htm , https://en.wikipedia.org/wiki/Geometric_algebra

