If a system having rigid boundaries is set into vibration with just right initial conditions, it would vibrate at a certain frequency (normal mode). But why we take the general motion to be superposition of all the normal modes , under arbitrary initial conditions. It doesn't make sense to me. Does this mean the system is vibrating at all possible frequencies at the same time?
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
Yes, in general the motion will involve multiple normal mode frequencies simultaneously.
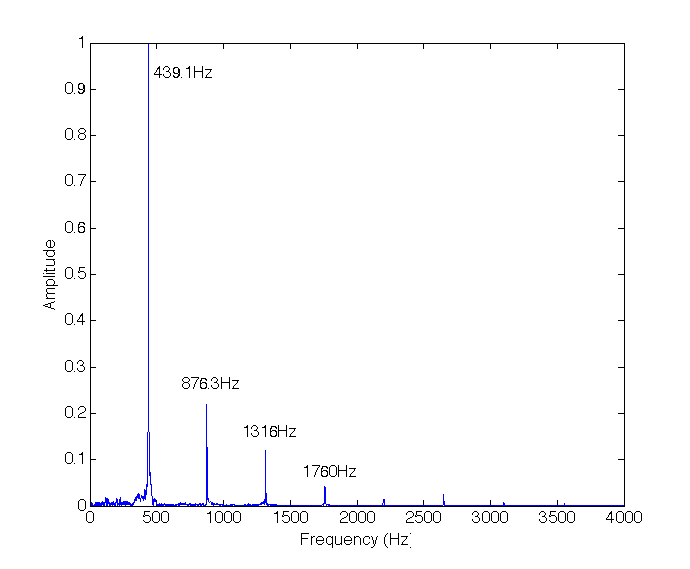
For a familiar example of motion with multiple simultaneous frequencies, consider a musical instrument — a piano, say. When you hammer a piano string, the string produces multiple harmonics in additional to the fundamental frequency. These additional frequencies change the sound you hear, and the pattern of harmonics is what determines the timbre or tone quality of a given instrument. I've included a plot of the frequency spectrum of a piano note (source). The amplitudes in the plot correspond to the amplitudes in the linear combination of different normal modes.
