0
$\begingroup$

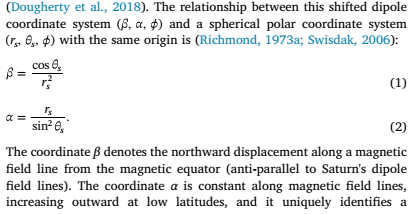
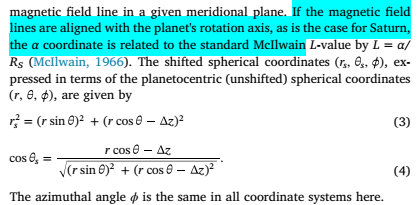
Introduction

A planetary magnetic field $\vec{B}$ can be described outside of the planet using Gauss coefficients $g_n^m$ and $h_n^m$ and a spherical harmonic expansion:

$$\vec{B} \;=\; -\vec{\nabla} V(r,\theta,\phi)$$

and

$$V(r,\theta,\phi) \;=\; \sum_{n=1} \sum_{m=0}^l R_p \left(\frac{R_p}{r}\right)^{n+1} \big[g_n^m \cos(m\phi) + h_n^m \sin(m\phi) \big] P_n^m(\cos\theta) \text{,}$$

where $R_p$ is the planetary radius and $P_n^m(\cos\theta)$ are the associated Legendre polynomials.

If we assume the magnetic field is axially symmetric, then only the $m=0$ terms remain:

$$\begin{align} V(r,\theta,\phi) \;=&\; \sum_{n=1} R_p \left(\frac{R_p}{r}\right)^{n+1} g_n^0 P_n^0(\cos\theta) \\ =&\; \sum_{n=1} R_p \left(\frac{R_p}{r}\right)^{n+1} g_n^0 P_n(\cos\theta) \text{,}\end{align} $$

where $P_n^0(\cos\theta) = P_n(\cos\theta)$ are the Legendre polynomials.

My Question

Suppose I have the axisymmetric Gauss coefficients (say, $g_n^0$ for $1 \le n \le N$) for a given planet in a coordinate system centered on the planet. Let's suppose the magnetic equator is located northward from the planet's equatorial plane by a distance $\Delta z$, which implies the magnetic field is not symmetric about the planet's equator.

I want to treat the magnetic field as a dipole, probably in a coordinate system shifted northward along the polar axis by $\Delta z$. How could I calculate from those coefficients the "best-fit" dipole field in the shifted coordinate system?

Improve this question
$\endgroup$
4
  • $\begingroup$ Transform the field to Cartesian coordinates and make a shift. $\endgroup$
    – Alex Trounev
    Commented Mar 15, 2019 at 22:20
  • $\begingroup$ @AlexTrounev: How would I do that if I only have the Gauss coefficients for the unshifted field? Sure, I could transform the coordinates and vector components, but I'd still have the higher order terms. If I shifted only the dipole term, I'd lose the effect of the higher-order terms on the shifted dipole. How would I get the dipole term in the shifted coordinate system? $\endgroup$
    – jvriesem
    Commented Mar 26, 2019 at 21:50
  • $\begingroup$ You have a function $V(r,\theta , \phi )$. Transform this function to Cartesian coordinates $V(x,y,z)$. Make a shift $z=z'+\Delta z$. $\endgroup$
    – Alex Trounev
    Commented Mar 26, 2019 at 22:42
  • $\begingroup$ @AlexTrounev That’s what you said in your first comment. My reply asked a question. Shifting coordinate systems — by itself — doesn’t give a new value or expression for a coefficient in the unshifted frame. (I actually do as much already in a similar problem.) Shifting coordinate systems is trivial. I don’t know how that would affect the dipole term. Perhaps it would help if you expressed your intentions mathematically: something like $g_1^{0,’} = f(g_1^0)$, where the prime denotes the shifted frame. $\endgroup$
    – jvriesem
    Commented Apr 8, 2019 at 4:33

1 Answer 1

Reset to default
0
$\begingroup$

First write the equations with the higher order terms (https://astronomy.stackexchange.com/questions/51307/the-path-of-a-particle-in-planets-magnetic-field-doesnt-not-seem-to-change-wit/51313#51313). once you have done that all you need to do is replace the coordinates (r,theta, phi or x,y,z) with the transformed ones as show below

I am not re typing since it is nicely written in the papers. shifted dipole coordinate enter image description here J.W. Vriesema, et al. Icarus 344 (2020) 113390 Also look at Swisdak, M., 2006. Notes on the Dipole Coordinate System. pp. 1–6. arXiv:0606044 http://arxiv.org/abs/physics/0606044

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.