Tell me more ×
Physics Stack Exchange is a question and answer site for active researchers, academics and students of physics. It's 100% free, no registration required.
up vote 9 down vote favorite
5

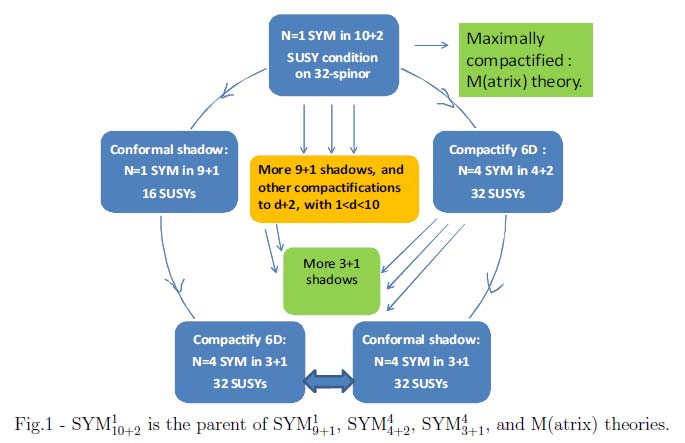
I have recently been looking into the two-time theories and the implied concepts.

For me this seems slightly hard to grasp.

How can I see the basic concept in this theory in a fundamental way based on its implied interaction with normal 3+1 dimension?

I am interested specifically in how gauge symmetries that effectively reduce 2T-physics in 4+2 dimensions to 1T-physics in 3+1 dimensions without any Kaluza-Klein remnants.

share|improve this question
1  
Possible duplicates: physics.stackexchange.com/q/43322/2451 and links therein. – Qmechanic♦ Dec 9 '12 at 21:24
7  
"slightly hard to grasp." My friend, if you have understood one time dimension, you are already a king among physicists. – kηives Dec 10 '12 at 1:19
2  
Great comments if someone could combine them into a coherent and collective answer that would be great. If I have the time tonight, I will try to do this myself. – Argus Dec 10 '12 at 20:20
2  
@Jerry No, theories with two time dimensions can be ok if these are only infinitesimal, such as applied in Cumrun Vafa's F-theory for example. If they were macroscopic there would of course be large problems. – Dilaton Dec 11 '12 at 17:39
3  
@jerry next time, use the comments area for stuff which doesn't answer the question.. :) – Manishearth♦ Dec 12 '12 at 11:01
show 7 more comments

Know someone who can answer? Share a link to this question via email, Google+, Twitter, or Facebook.

Your Answer

 
discard

By posting your answer, you agree to the privacy policy and terms of service.

Browse other questions tagged or ask your own question.