2
$\begingroup$

As far as I know current is the amount of charge passing per unit time.

$$I=q/t$$

When current passes through a resistor, the resistor resists the flow of current so the amount of charge going to the resistor and leaving the resistor is the same (no charge accumulation)

but the time component increases, i.e. it takes more time for the charge to travel through the resistor.

Maybe I'm missing something here.

My question is related to If the current is increased, is there more charge flowing or is it moving quicker?

Improve this question
$\endgroup$
5
  • $\begingroup$ A counter question: if the current at the two ends of the device were different, where would the charge go? A resistor does not radiate electrons, and if the current were different, there would be either a buildup of charge or charge being generated out of nowhere (which both can't happen). $\endgroup$
    – nate
    Commented May 13, 2020 at 18:46
  • $\begingroup$ I'm trying to say, charges are indeed conserved but, they move through resistor slowly , decreasing the current (but amount of free electrons is the same). For some reason everyone's assuming that current = charge, but current = charge/ time $\endgroup$
    – Chemist
    Commented May 14, 2020 at 2:02
  • $\begingroup$ “it takes more time for ...” More time compared to what? $\endgroup$
    – Dale
    Commented May 14, 2020 at 3:19
  • $\begingroup$ Pl have a look at my answer and share your thoughts. $\endgroup$
    – Chemist
    Commented May 14, 2020 at 3:23
  • $\begingroup$ This is how I think of it: Due to its position in the electric field, it has some electric potential energy. Now, as it moves in the direction of the field, the potential energy reduces and is converted to kinetic energy. But, there are various obstructions in a resistor. So, as soon as it gains the kinetic energy it loses it due to Collison (predominantly as heat). So, overall the rate of the charges moving is the same. Feel free to correct me if wrong. $\endgroup$
    – Kaushik
    Commented Jul 27, 2020 at 7:25

8 Answers 8

Reset to default
2
$\begingroup$

If the current is larger more charge flows through the resistor per second.

What you are missing is the fact that the current through your resistor is controlled not only by the resistance of your resistor but the circuit elements of a complete electrical circuit of which your resistor is one part.

Your resistor not only has an effect on the current passing through it but also the currents passing through other circuit elements to which it is connected.
In the end as there is no source or sink of charge within your resistor, the current entering your resistor coming from the circuit to which it is connected to is equal to the current leaving the resistor and that current is going into the circuit to which your resistor is connected to.

Improve this answer
$\endgroup$
3
  • $\begingroup$ physics.stackexchange.com/a/108050/141548 $\endgroup$
    – Chemist
    Commented Jan 13, 2019 at 8:13
  • $\begingroup$ It still doesn’t make sense though $\endgroup$
    – Chemist
    Commented Jan 13, 2019 at 8:14
  • $\begingroup$ I found the culprit, I always used to assume current flowing the wire is equivalent to water flowing through pipe. Could you please add more details to the answer though, so they it will benefit the other viewers $\endgroup$
    – Chemist
    Commented May 13, 2020 at 17:56
1
$\begingroup$

This is what you are missing here:

Let's model your system as a water hose where a small length of it is constricted in diameter. We'll consider that constricted length to be our resistor.

The flow of water through the hose is analogous to the flow of electrical charge through a circuit and the pressure responsible for squeezing the water through the resistor in the hose is analogous to the voltage that is pushing the charge through the circuit.

Since our hose has no leaks, every bit of the water flowing into one end of the constriction has to flow out the other end. Analogously: charge is conserved, so all the charge flowing into one end of the resistor in the circuit has to come out the other end.

This is the reason that a resistor does not make the current flowing through it disappear.

Improve this answer
$\endgroup$
0
0
$\begingroup$

We know that charge on the conducting material(resistor) is zero. Then amount of charge flowing into= amount of charge flowing out of resistor. Hence rate of charge flowing in = Rate of charge flowing out. Therefore current doesn't change.

Improve this answer
$\endgroup$
7
  • $\begingroup$ I have mentioned the same , the charge does not stay there but it moves slowly due to restriction by resistor. Causing time component to increase $\endgroup$
    – Chemist
    Commented Jan 13, 2019 at 7:29
  • $\begingroup$ If we are able to store the charge continuously then current is not same on both sides. $\endgroup$
    – Sandesh Goli
    Commented Jan 13, 2019 at 7:30
  • $\begingroup$ I agree that the charge does not stay there but it moves slowly $\endgroup$
    – Chemist
    Commented Jan 13, 2019 at 7:31
  • $\begingroup$ We know that i=neav (v=drift velocity). In resistor 'v' decreases, 'n' increases. All the variables adjust themselves. $\endgroup$
    – Sandesh Goli
    Commented Jan 13, 2019 at 7:33
  • 1
    $\begingroup$ @NagaSandeshGoli even the time component will increase, along with the number density. If you use the current density formula ($j$), you can see the relation. $\endgroup$
    – Karthik
    Commented Jan 13, 2019 at 7:42
0
$\begingroup$

Imagine water draining a tank though a hose.

The amount of water in the hose is constant, so what goes in comes out.

That’s true whether the hose is large and free flowing with a lot of water moving, or small and constructed with only a little flowing.

The flow of water (analog to the current)does not decrease from the input to the output of the hose.

Improve this answer
$\endgroup$
1
  • 3
    $\begingroup$ See charges going inside are coming out I totally agree with that, but current is not an object it’s measure of rate of flow of charge so my argument is that rate of flow of charge decreases due to resistance $\endgroup$
    – Chemist
    Commented Jan 13, 2019 at 7:52
0
$\begingroup$

The image below, courtesy of Professor Richard Fitzpatrick, from the University of Texas at Austin, shows a resistor R connected in a simple circuit to a battery, which has internal resistance r. Simple resistor circuit

The battery supplies an (almost) constant voltage E, and by Ohms's law, the current I in the circuit is given by $$\mathrm{I=\frac E {R+r}}$$

If the resistance of R is increased the current in the circuit decreases, and vice versa. But for a given resistance the current is constant over the whole loop. That's because charge is conserved, so the charge flowing into any point of the loop must equal the charge flowing out of that point.

So yes, the resistor restricts the current, but it does so over the entire loop. If the current leaving the resistor were lower than the current entering the resistor, that would mean that charge was somehow leaking out of the resistor (or being destroyed by it!), and leaving the circuit. The resistor loses energy, by emitting heat, but it doesn't lose charge.

Improve this answer
$\endgroup$
0
$\begingroup$

Maybe this could help. As a first approximation consider the Drude/free electron model

Conductivity = $n e^2 \tau / m$

$n$ is electron density. That changes from material to material. $e$ and $m$ are electron charge and mass which are universal constants $\tau$ is the mean time between collisions. This could change with temperature, addition of impurities etc.

When you have a higher resistance you have lower current . It could be because it has a lower $n$ or smaller time between collisions.

When you say less charge or more time you have to look at exactly the situation. Compared to what? If you are replacing a carbon resistor with another of higher value, actually neither $\tau$ nor $n$ change. Only dimensions. If you are changing the temperature, ( to a first approximation) $n$ does not change. If you replace carbon with copper both change.

Improve this answer
$\endgroup$
0
$\begingroup$

This is in addition to farcher's answer.

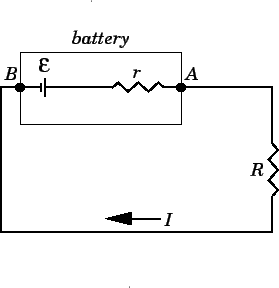
An analogy for The current in the circuit

Scenario

A person is standing , and there is a very long multiple line of restless kids along with their parents, kids are moving around (due to hot weather) in front of a the person , and there is a Small stretch of Barricades in between the kids s somewhere in the the line. Something like this 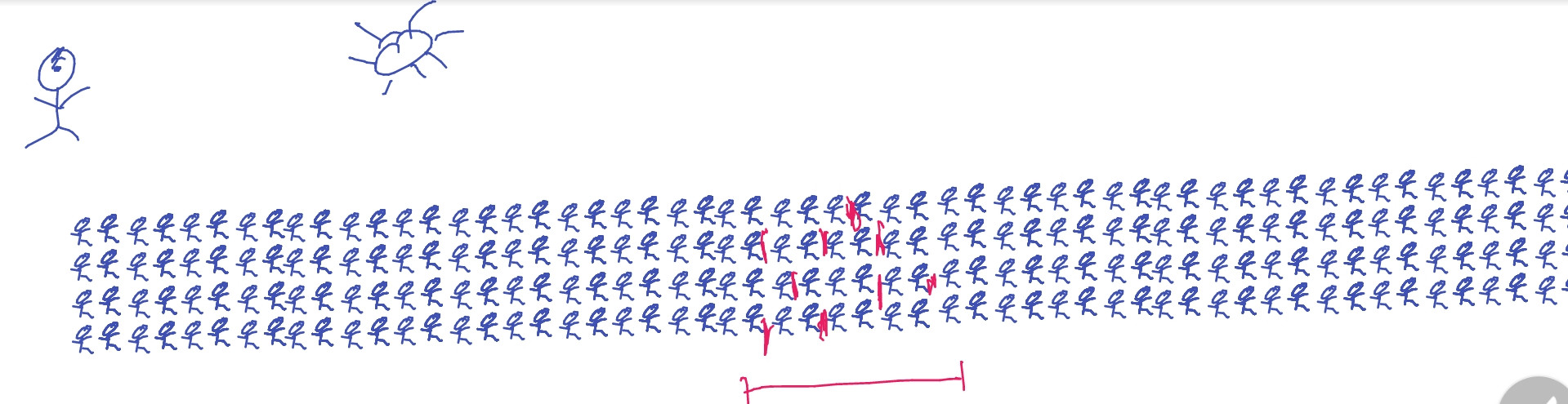enter image description here (I forgot to draw the parents, assume they are there.)

Scene

Now the person(battery) shouts "snake" (creates electric field in the wire), the kids in front of him immediately hear it and starts running away from him ( effect of electric field on charges),

[but kids at the other end haven't stated to move as sound didn't reach them yet (speed of light is capped and not infinite, so is electric field)],

now moving kids stumble with other's parents and fall down , but get up and start running again this keeps repeating, (parents are smart won't run ; ) )

and region where the barricades(resistor)¹ are present , the kids stumble with barricades a lot more (high Collison frequency, and decrease in time between collisions), beacause of this the kids before the barricades experience traffic snake effect, aka traffic wave . [Video on it] (https://m.youtube.com/watch?v=Q78Kb4uLAdA)

So the all kids before the Barricades move slower than their max capability ( if barricades wasn't there at all). Till now we just saw what happens upto charge flow to a certain point in the circuit

Now comming back to real world

It's a fact that charge leaving battery is equal to charge entering the battery,

so when resistor is connected as explained above charge leaving the battery decreases

So consequently charge entering the battery decreases

, Therefore current decreases in the entire circuit.

So basically this explains the statement " resistor not only has an effect on the current passing through it but also the currents passing through entire circuit to which it is connected".

P.S

So statement in my question is disproved. Also this an original analogy that I came up with, although not perfect , but it makes people visualise lots of things.

¹Please note that barricades in the resistor actually don't exist , the obstacles in the resistor is of similar size as that of condutor. Here it's said so to simplify the explanation.

Improve this answer
$\endgroup$
0
$\begingroup$

I got your problem. Look the charges entering the resistor feel more forces when they enter the resistor and thus slow down but the moment they leave the resistor there is no restricting force at all on them and it is similar to the conditions which was earlier (before the entry) I.e the only force is due to electric field . So the charges regain their previous speed and thus current doesn't drop after passing through the resistor.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.