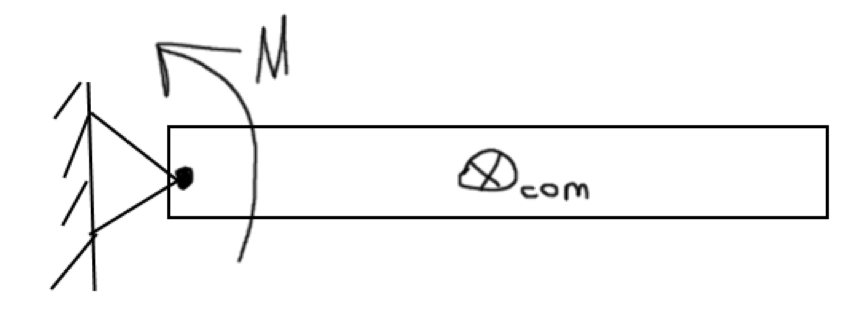
A rigid body with a net moment applied to it is clearly NOT in static equilibrium. Therefore it moves. The only thing that may be puzzling is how it moves, consistent with Newton's laws of motion.
To get started untangling the confusion, forget about the pin. You now have a rigid body with no constraints on its motion, and a moment applied to it. The moment will cause angular acceleration about the COM, and since there are no net forces, the COM will not move.
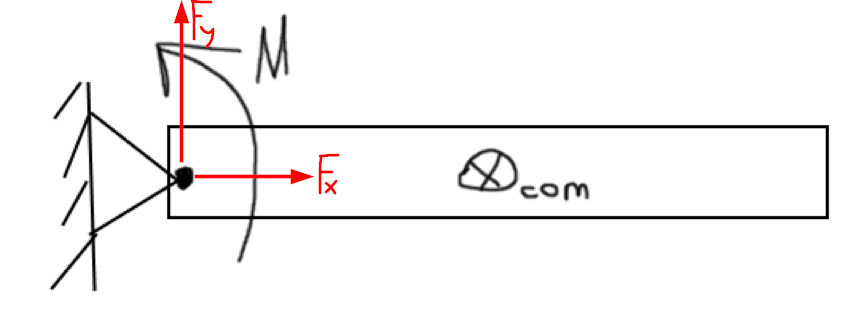
But in your example, this can't happen, because the end of the bar is constrained not to move by the pin. The pin will apply a constraint force to the bar, perpendicular to it, to prevent it moving.
That constraint force will cause the COM to accelerate upwards, such that the motion of the bar is a rotation about its end, not about its COM. If the bar as shown in your picture is along the $x$ axis, then $\ddot y = (l/2)\ddot \theta$ where $l$ is the length of the bar, $y$ is the acceleration of the COM in the $y$ direction, and $\theta$ is the angle of rotation of the bar.
That is where the "force to accelerate the COM" is coming from. As the bar rotates, the reaction force will change, because there will also be a component along the length of the bar, causing the centripetal acceleration that makes the COM move in a circle.
Note that the reaction forces do no work, and therefore the easiest way to formulate the equation of motion is equate the work done by the moment and the kinetic energy of the bar, and use the constraint equation given earlier to link the translational and rotational motion of the bar.