Can I calculate Young's modulus from density? Is there any mathematical relation between Young's modulus and density?
$\begingroup$
$\endgroup$
3
-
$\begingroup$ For an ideal gas, the bulk modulus is proportional to density (at constant temperature). That is the only mathematical connection. Nothing similar exists for solids. $\endgroup$– user137289Commented Dec 9, 2018 at 12:35
-
$\begingroup$ For many solid materials, the ratio of $E$ to $\rho$ tends to be similar, but there is no "exact" mathematical relationship - unless you can calculate both of them accurately from the atomic structure of the solid, which is probably not what the OP had in mind! $\endgroup$– alephzeroCommented Dec 9, 2018 at 13:36
-
$\begingroup$ For the free electron gas, one can derive this mathematical relation: ps.uci.edu/~cyu/p238A/LectureNotes/lecture2/lecture2_html/… $\endgroup$– user137289Commented Dec 9, 2018 at 14:10
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
1
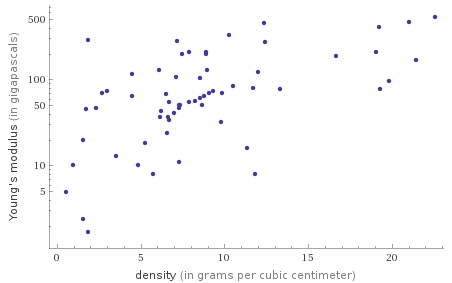
There's no general mathematical relation between stiffness (as parameterized by an elastic modulus such as Young's modulus) and density for all materials, but a relationship can be defined for an ideal gas, and a general trend exists for condensed matter.
For fluids such as gases and liquids, Young's modulus is zero; you won't encounter any resistance if you slowly pull on these materials uniaxially. However, the bulk modulus (i.e., the resistance one encounters when trying to compress these materials using pressure) is not zero; for an ideal gas, it is exactly equal to the pressure. One can relate this relation to the density $m/V$ using the ideal gas law $PV=nRT$ and the molecular weight of the gas.
For condensed matter, the stiffness generally scales up with the density:
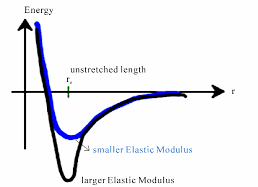
The reason is that both properties depend on the pair potential between atoms; if the atoms are strongly bonded, then their spacing is generally small; thus, the density is high. In addition, the curvature of the pair potential at the equilibrium position is large, which is exactly equivalent to saying that the material is stiff:
(A third implication to strong bonding is that the pair potential dips deep and that the material is refractory, i.e., it has a high melting temperature):
An active area of research is to precisely define the pair potential in condensed matter to theoretically predict both stiffness and density; this is something you might pursue in an academic or industry environment.
answered Dec 9, 2018 at 19:07
-
$\begingroup$ Those graphs are for the elements. I suspect that including salts, oxides, and other compounds would leave even less of a correlation. $\endgroup$– user137289Commented Dec 9, 2018 at 20:36