Topological indices for systems that lack translational invariance

Question

I have a 1D discrete, finite system that lacks translational invariance. It appears to have edge states, in much the same way as an SSH model has edge states. In the SSH model we can study the infinite version of the finite chain to calculate a topological index, the Zak phase. I am trying to think if I can define (or there already exists) some kind of topological index that captures the presence of these edge states. However my system lacks translational invariance. Any suggestions on how to approach this? I have found the Bott index, but it appears to only be defined for 2D systems.

Answer 1

The Bott index is essentially limited to finite, 2D systems with periodic boundary conditions. There may be some applications of the Bott index to a 1D system that will be found someday. I assume you have a new system and you want an established index to compute.

What you are looking for are real-space formulas for the index/K-theory. There are formulas for open boundary conditions and different formulas for periodic boundary conditions. For an index you compute based on periodic boundary conditions, see the work of Emil Prodan and coauthors. Some of his papers numerical studies invoving 1D systems in class AIII.

Since you are seeing boundary states, I assume you are fine working with open boundaries. I wrote out formulas for all five relevant symmetry classes for 1D systems in section 4 of my paper "K-theory and pseudospectra for topological insulators" but I did not publish a study of 1D systems.

A numerical study, in class BDI and in 1D, using the pseudospectral index, was published recently: Liu, Dillon T., Javad Shabani, and Aditi Mitra. "Long-range Kitaev chains via planar Josephson junctions." Physical Review B 97.23 (2018): 235114.

A word of warning. The formulas involves the operator $\kappa X + iH$ where $X$ is position (here assumeing that the system is centered at position zero and the spectral gap also around zero). The small positive consant $\kappa$ is a necessary tuning parameter. Roughly speaking, one requires is to be chosen so that $\kappa X$ has norm roughly equal to the gap in the corresponding infinite system. One must experiment.

Assuming you are working in class AIII, the formula involves the grading operator $\Gamma$ which in $+1$ on the even basis vectors and -1 on the odd basis sites. In physics this is often denoted $S$. The index is the integer $$\frac{1}{2} \mbox{sig}(\kappa X + iH)\Gamma .$$ The signature can be computed without finding all the eigenvalues quickly, and is just the number of positive eigenvalues minus the number of even eigenvalues.

Answer 2

The index for spectrally gapped, one-dimensional chiral systems (i.e. the disordered version of the SSH model) was already defined for both bulk (infinite) or edge (half-infinite) systems in the work of Schulz-Baldes and Prodan (see their Springer textbook from a few years ago for an introduction). This essentially extends the ground-breaking work of Bellissard, van Elst and Schulz-Baldes to the chiral case in all dimensions.

Allow me to summarize the results succinctly: when you have chiral symmetry (being implemented by some operator $\Pi=\begin{bmatrix}Id&0\\0&-Id\end{bmatrix}$), your Hamiltonian (which obeys $\{H,\Pi\}=0$) is necessarily written in block form as $H=\begin{bmatrix}0&S^\ast\\S&0\end{bmatrix}$ for some operator $S$ (not necessarily self-adjoint). If you consider the half-infinite system, the index is simply the Fredholm index of $S$ (the spectral gap condition combined with the locality of Hamiltonians guarantee that $S$ is indeed a Fredholm operator). This index counts the signed (by chirality) number of zero modes of the edge Hamiltonian.

Conversely if you consider the infinite system, if $P$ is your Fermi projection (for chiral systems one must put the Fermi energy at zero) then your index is $2 tr(\Pi P [X,P])$ where $tr$ is the trace-per-unit-length and $X$ is the position operator.

Prodan and Schulz-Baldes also prove the bulk-edge duality for spectrally gapped systems in their text book, showing that these two indices are equal if you take an infinite system and cut it in the middle.

Answer 3

Case Study

Let's see if I've understood some things. Consider the (simplified) Hamiltonian from this paper

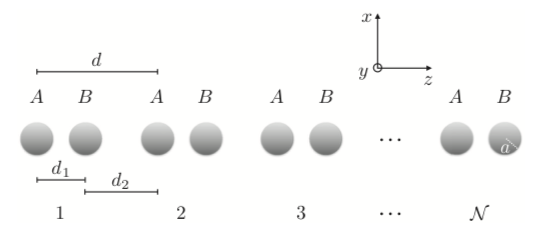
$$H=\sum_{n=1}^N \Omega_1 a_n^\dagger b_n + \sum_{n=1}^{N-1} \Omega_2 a_{n+1}^\dagger b_n + \text{H.c.}$$

which describes interactions between nearest neighbours on a dimerised chain with basis particles $A$ and $B$. Here, $\Omega_m = (1/2)(a/d_m)^3$, $d_1=d(1+\epsilon)/2$, $d_2=d(1-\epsilon)/2$, $d=d_1+d_2$ and $\epsilon \in \mathbb{R}$ controls the dimerisation. We choose $a=d/6$.

Infinite system (periodic boundary conditions)

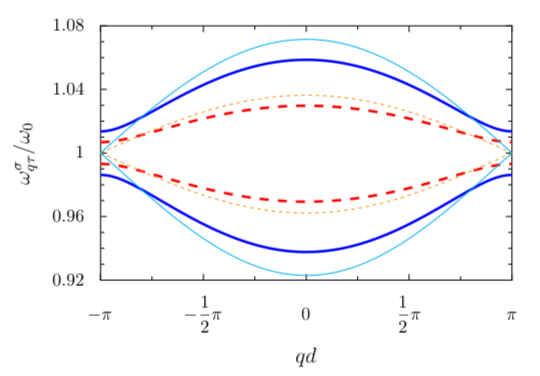
For $\epsilon<0$ the system has a Zak phase of zero and no-edge states. For $\epsilon>0$ the system has a Zak phase of $\pi$ and does have (two) zero-energy edge states. Of course these topological indices have been calculated from the momentum space representation which requires periodic boundary conditions. In addition we can analytically calculate the band-gap between the upper and lower band as $\Delta=2|\Omega_1-\Omega_2|$(see image below, concentrate on just the blue curves for example).

Finite system

Let's try to calculate a topological index for the finite system with open boundary conditions (where the edge states actually manifest). The Hamiltonian can be rewritten as

$$H=\Psi^\dagger \mathbf{H} \Psi,$$

where $\Psi^\dagger=(a^\dagger,b^\dagger)$, $a^\dagger = (a_1^\dagger, a_2^\dagger, \dots, a_N^\dagger)$ (and similarly for $b^\dagger$) and

$$\mathbf{H} = \begin{pmatrix} 0 & \mathbf{\Omega}\\ \mathbf{\Omega}^\dagger & 0 \end{pmatrix}. $$

Here

$$\mathbf{\Omega} = \begin{pmatrix} \Omega_1 & \Omega_2 & 0 & 0 &\dots \\ 0 & \Omega_1 & \Omega_2 & 0 & \dots \\ \vdots & \vdots & \ddots & \ddots & \dots \end{pmatrix}. $$

is a band-diagonal matrix with $\Omega_1$ along the diagonal and $\Omega_2$ along the 1-diagonal.

This system is in the class BDI (I get the same result if I use the measure for the AIII class), therefore we can use the index developed in Loring's paper (here)

$$Z=(1/2)\text{sig}(\kappa \mathbf{X} \mathbf{\Gamma} + \mathbf{H}).$$

Here $\text{sig}(M)$ is literally just the number of positive eigenvalues of $M$ minus the negative ones.

We choose the tuning parameter to be $\kappa=\Delta$ which is the band-gap in the infinite system.

Following this paper, we choose $\mathbf{X}$ to be a diagonal matrix with the diagonal elements the literal physical positions of the particles, but in units of the chain length $L=Nd$ and centred around zero, i.e.

$$\text{diagonal}(\mathbf{X})=(1/L)(-L/2,-L/2+d_1,-L/2+d,-L/2+d+d_1,\dots,L/2)$$

Finally we have $\mathbf{\Gamma}=\sigma_3 \otimes \mathbb{1}_N$, where $\sigma_3$ is a Pauli matrix.

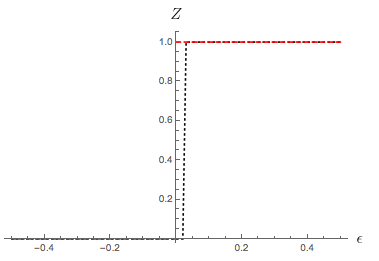
Below I plot the Zak phase (in units of $2\pi$, in red dashed line) and the finite index $Z$ (in black dotted line). We see that they more-or-less agree. Although the finite index remains 'topologically trivial' $Z=0$ for values of $\epsilon$ slightly above zero. Perhaps this makes sense, because here the spectrum has a small band-gap.

Ok, so I seem to have arrived at something sensible. Although the whole thing feels like a black box to me. I just can't digest what the motivation is for the measure. A 'tuning' parameter $\kappa$ also makes me very uncomfortable, because if I get the 'wrong' answer this just allows me to tune it to the 'right' answer. What if I don't know what the right answer is? What if I can't calculate the band-gap of the infinite system (because it doesn't have any sort of translational invariance for example). How can I be confident in my result? I'm also not sure if I interpreted what $\mathbf{X}$ should be correctly. Is it correct? Can I also choose it to be something slightly different?

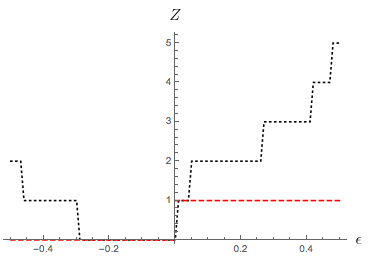
For example, what if I choose $\kappa=2\Delta$ then I get this incorrect result