0
$\begingroup$

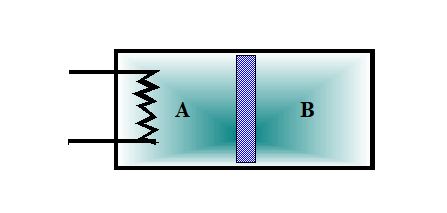
A rigid cylinder contains a freely moving piston. Initially, it divides the cylinder into equal volumes, and each side of the piston contains 1 mole of an ideal gas at $5^{\circ}C$ and $1$ bar. An electrical resistance heater is installed on side A of the piston and is energized to slowly heat the gas on side A to $170^{\circ}C$. If the tank and the piston are perfect insulators, calculate the heat added to the system by the resistance heater. The molar heat capacities of the gas are: $C_v= (3/2)R$ and $C_p=(5/2)R$.

enter image description here

My approach: We can calculate $P,V,T$ of both sides initially. Then some heat $Q$ is added to the system. $A$ is heated up and the piston is pushed towards $B$. Therefore some work is done on $B$ and some work is lost by $A$. We can calculate the total $\Delta U_A = nC_v\Delta T$. We can use the adiabatic relationships $PV^\gamma = k$ or $TV^{\gamma -1} = k'$ on system $B$. However, we do not know the exact volume lost by $B$ to get the final results. We can say that the final pressures on both sides are the same because the piston is in equilibrium. Although, I still have one degree of freedom. How do I calculate work without knowing the final volume? And following that, how do I calculate the heat added to the system?

Improve this question
$\endgroup$
5
  • $\begingroup$ Is the same ideal gas on both sides? $\endgroup$
    – Bob D
    Commented Sep 11, 2018 at 20:15
  • $\begingroup$ Yes, it is the same ideal gas $\endgroup$
    – Sat D
    Commented Sep 11, 2018 at 20:17
  • $\begingroup$ You solve for both the final pressure and the final volumes using the equations you have written. You already said that you need to match the final pressures. Let P be an unknown. Then calculate the volumes on the two sides in terms of P, and solve for P so that the final volumes add up to the initial volume. $\endgroup$
    – Chet Miller
    Commented Sep 11, 2018 at 22:38
  • $\begingroup$ I updated my solution. $\endgroup$
    – Thermodynamix
    Commented Sep 12, 2018 at 0:51
  • $\begingroup$ @Drew. I don't like it. There is a much simpler way. See my answer. $\endgroup$
    – Chet Miller
    Commented Sep 12, 2018 at 2:26

2 Answers 2

Reset to default
1
$\begingroup$

We have some constraints:

$P_{B2} = P_{A2} = P_2$ for mechanical equilibrium at end of process.

$V_A + V_B = V = constant$ throughout entire process.

Now write the ideal gas law for both systems at state $2$, solve for $V_{A2}$ and $V_{B2}$, and sum $V_{A2}+V_{B2}$ to get Equation 1:

$V = \frac{nRT_{A2}}{P_2} + \frac{nRT_{B2}}{P_2}$

where $P_2 = P_{B2} = P_1(\frac{V_1}{V_{B2}})^{\gamma}$ and $T_{B2} = T_1(\frac{V_1}{V_{B2}})^{\gamma - 1}$.

Applyng the First Law of Thermodynamics to the entire system ($A$ + $B$) yields Equation 2:

$\Delta E = n c_v(T_{A2}+T_{B2}-2T_1) = {Q}$

where $T_{B2} = T_1(\frac{V_1}{V_{B2}})^{\gamma - 1}$.

Now we have 2 equations and 2 unknowns ($V_{2B}$ and $Q$), so we can solve for both. Use Equation 1 to solve for $V_{2B}$, and then use with Equation 2 to solve for $Q$.

Improve this answer
$\endgroup$
0
1
$\begingroup$

$$V_i=\frac{nRT_i}{P_i}=\frac{(1)(8.314)(278.2)}{(1)(100000)}=0.0231\ m^3$$

$$V_{Bf}=V_i\left(\frac{P_i}{P_f}\right)^{1/\gamma}=0.0231\left(\frac{1}{P_f}\right)^{1/\gamma}$$ $$V_{Af}=\frac{nRT_{Af}}{P_f}=\frac{(1)(8.314)(443.2)}{(100000)(P_f)}=\frac{0.0368}{P_f}$$ where $P_f$ is in bars. $$V_{Af}+V_{Bf}=0.0462$$ Solve for $P_f$

The rest is easy.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.